With $x=2$ and $b \ge 1$ the claim is true.
Proof: If $G$ contains a cycle, there is your $2$-regular subgraph. Otherwise $G$ is cycle-free, so it is a forest. A finite forest has some leaves, that is, vertices of degree $1$. But this is impossible since we have assumed that all vertices have degree $x=2$ or $bx \ge 2$.
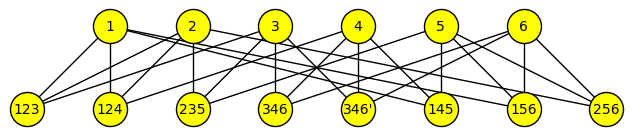
With $x=3$ and $b=4/3$ the claim is false. The following $(3,4)$-biregular graph has no $3$-regular subgraph.
This example is Figure 2 in Asratian et al. (2009). They note that it has no full $3$-regular subgraph (full meaning "one that covers all of the $4$-degree vertices"), but we can in fact find that it has no $3$-regular subgraph at all. It suffices to consider all $2^8-1=255$ nonempty subsets of the $3$-degree vertices (lower layer); in each case, take the subgraph of those vertices and all their neighbors; and observe that in all cases the resulting subgraph is noncubic. (This was, of course, a straightforward computational way; surely there are finer methods.)
Asratian, Armen S.; Casselgren, Carl Johan; Vandenbussche, Jennifer; West, Douglas B., Proper path-factors and interval edge-coloring of (3,4)-biregular bigraphs, J. Graph Theory 61, No. 2, 88-97 (2009). ZBL1198.05037.