The answer is no.
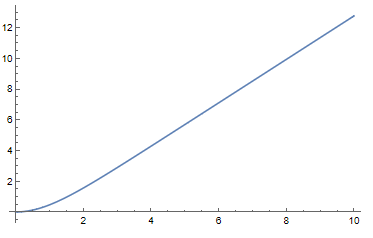
Indeed, suppose that $n=1$ and $f(x)=e^{-x}$. Then $$X(t)=2\ln\cosh\frac{t}{\sqrt{2}}.$$
So, the velocity $X'(t)=\sqrt{2} \tanh \dfrac{t}{\sqrt{2}}$ is increasing with $t$, and/but $X(t)\sim t\sqrt2\to\infty$ as $t\to\infty$.
Here is the graph $\{(t,X(t))\colon0\le t\le10\}$: