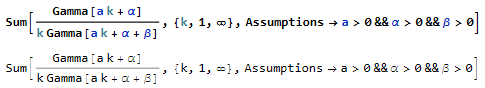Mathematica can do nothing this expectation in general:
So, it is highly unlikely that this expectation can be expressed in terms of elementary, or even special, functions.
However, we have this:
Also, writing $\ln(1-x^a)=-\sum_{k=1}^\infty\dfrac{x^{ak}}k$, we see that the expectation in question is $$-\frac{\Gamma (\alpha+\beta )} {\Gamma (\alpha)}\sum_{k=1}^\infty\frac{\Gamma (a k+\alpha )}{k\,\Gamma (a k+\alpha +\beta )}.$$ It is highly unlikely as well that the latter sum can be expressed in terms of elementary, or even special, functions: