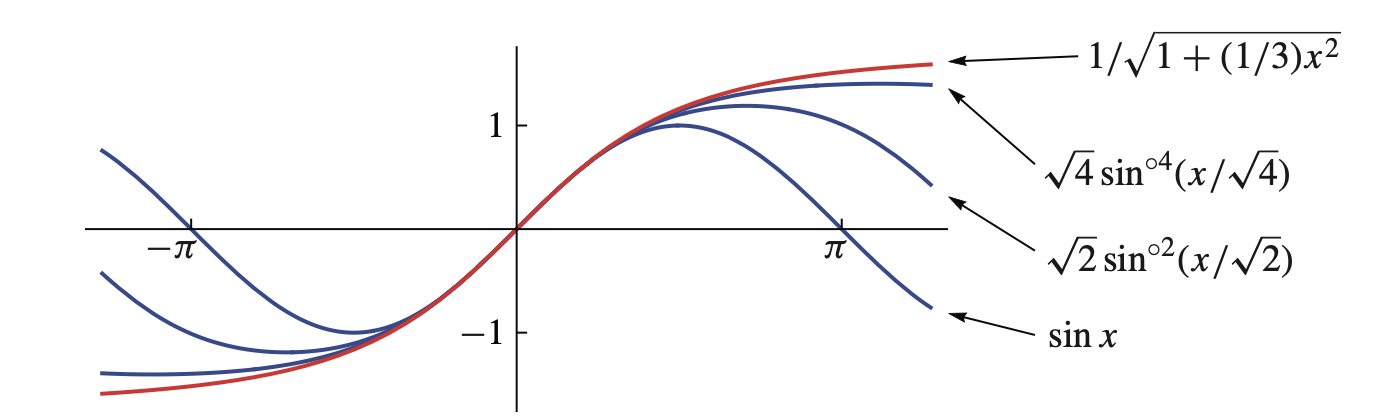
A rescaling is needed for a nontrivial limit. As discussed in Iteration of Sine and Related Power Series, denoting the $n$-th iterate by $\sin^{\circ n}x$, one has the limit
$$\lim_{n\rightarrow\infty}\sqrt n\sin^{\circ n}(x/\sqrt n)=\frac{x}{\sqrt{1+x^2/3}}.$$
The graph (from the cited paper) shows that the limit is attained quite rapidly.
Without the rescaling the iterated sine converges to zero (for the reasons indicated in the comments to the OP). The convergence is slow, see the graph.

