I am sudying enriched categories, and as I wrote in my previous question, this is very difficult because there are no elementary books on this topic (so I hope that specialists in category theory will not kill me for what I am asking here).

In particular, I am looking from time to time at the Tannaka duality theorem for modules over monoids $A$ in a symmetric monoidal category $V$, and I have a feeling that it can be proved without the Yoneda lemma which is suggested as an intrument in this situation).

Am I right?

My impression is that everything must be simple, although I can't restore the details (and I hope that somebody will help me).

Suppose we have a monoid $A$ in a symmetric monoidal category $V$ and we consider the enriched category $_AV$ of modules over $A$, and the forgetful functor $F:{_AV}\to V$. It's easy to see that the family of maps $$ \alpha_X:A\to[F(X),F(X)], $$ where $\alpha_X$ corresponds to the $A$-module structure $\mu_X:A\otimes X\to X$ on $X\in\operatorname{Ob}({_AV})$, is what is called a wedge from $F$ to $F$.

Our aim is to prove that for any other wedge $$ \beta_X:B\to[F(X),F(X)] $$ there is a unique morphism $\omega:B\to A$ such that the diagrams in the family 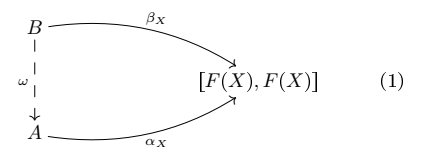(with $X\in\operatorname{Ob}({_AV})$) are commutative.

I guess that this $\omega$ is defined as the composition $$ B\stackrel{\beta_A}{\longrightarrow}[A,A] \stackrel{[\iota,1_A]}{\longrightarrow}[I,A] \stackrel{\rho_{[\iota,1_A]}^{-1}}{\longrightarrow}[I,A]\otimes I \stackrel{\operatorname{ev}^A_I}{\longrightarrow}A $$ (where $I$ is the unit in $V$, $\iota:I\to A$ the unit in the monoid $A$ and $\rho_{[\iota,1_A]}$ the right identity for $[\iota,1_A]$), but I don't understand how to prove the commutativity of diagrams (1).

In particular, the special case of (1)

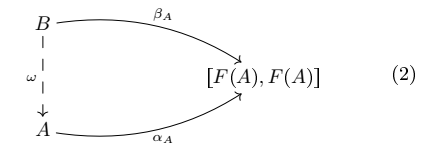
is also a puzzle for me. This is the same as the equality of the morphisms $$ B\otimes A\stackrel{\beta_A\otimes 1_A}{\longrightarrow}[A,A]\otimes A \stackrel{[\iota,1_A]\otimes 1_A}{\longrightarrow}[I,A]\otimes A \stackrel{\rho_{[\iota,1_A]}^{-1}\otimes 1_A}{\longrightarrow}[I,A]\otimes I\otimes A \stackrel{\operatorname{ev}^A_I\otimes 1_A}{\longrightarrow}A\otimes A \stackrel{\mu_A}{\longrightarrow}A $$ and $$ B\otimes A\stackrel{\beta_A\otimes 1_A}{\longrightarrow}[A,A]\otimes A \stackrel{\operatorname{ev}^A_A}{\longrightarrow}A $$ but why are they equal? I can check this only in the situation when $A$ is a usual monoid (in the category of sets) or a usual algebra.

I would appreciate if anybody could show this "bookkeeping" (as Fosco Loregian calls it), because for an outsider like me this is like a chinese writing. As I wrote in my previous question, each elementary step in this field is a problem, it requires weeks of pondering for me.