I quote from Barry Simon, Harmonic Analysis: A Comprehensive Course in Analysis, Part 3 (page 197):
That any positive harmonic function is constant is due to Bôcher (1902), although the theorem is often named after Picard’s rediscovery (1923) — there is often reference to the Liouville–Picard theorem.
Bôcher states the theorem in a footnote:
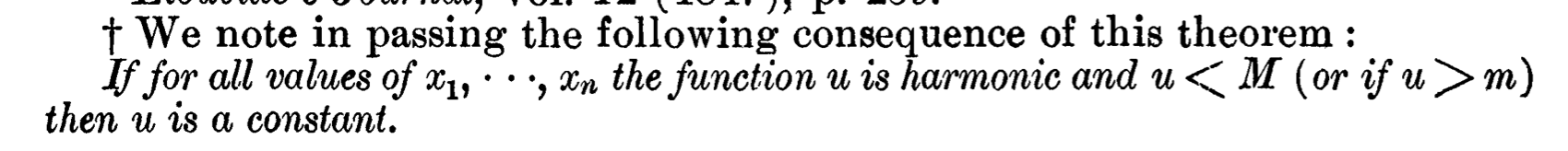
M. Bôcher, Singular points of functions which satisfy partial differential equations of the elliptic type, Bull. Amer. Math. Soc. (2) 9, (1903), 455–465.
É. Picard, Deux théorèmes élèmentaires sur les singularités des fonctions harmoniques, C. R. Acad. Sci. Paris 176 (1923), 933–935.
