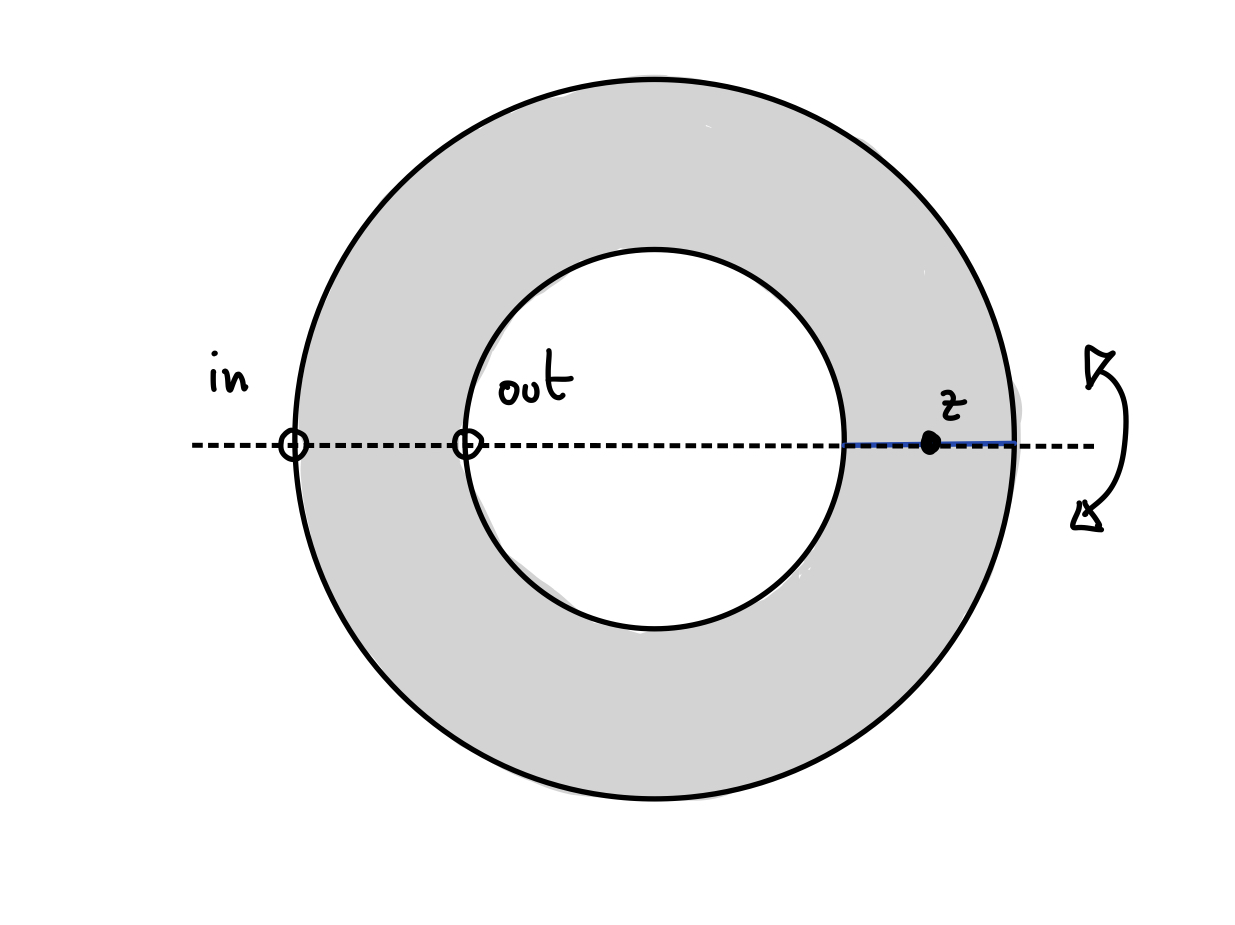
I'm interested in understanding a little bit better the space of configurations of an annulus with a marked point on the interior boundary component (let's call it "out") a marked point on the exterior one (say "in") and an interior marked point "z" free to move on a segment connecting the two boundary components. Moreover I wish to consider only those configurations satisfying a symmetry condition given by reflection along the horizontal axis

As far as I understood (following mainly the PhD thesis of Melissa Liu ) to construct such moduli space for an annulus $(A,\text{in},\text{out},z)$ we start with the moduli space of the double of the annulus $(A_{\Bbb C},\sigma,\text{in},\text{out},z,z')$ were $\sigma$ is the antiholomorphic involution (should be well defined up to iso from the construction of the double). The way I'm thinking about it is to consider the standard torus $S^1\times S^1$ in $\Bbb R^3$ and the plane $(z=0)$ to be the plane of symmetry. $\sigma$ corresponds to reflecting along this plane. In this model the boundaries of the annulus lie on such plane and hence $\sigma(\text{in})=\text{in}$, $\sigma(\text{out})=\text{out}$ and we require $\sigma(z)=z'$.

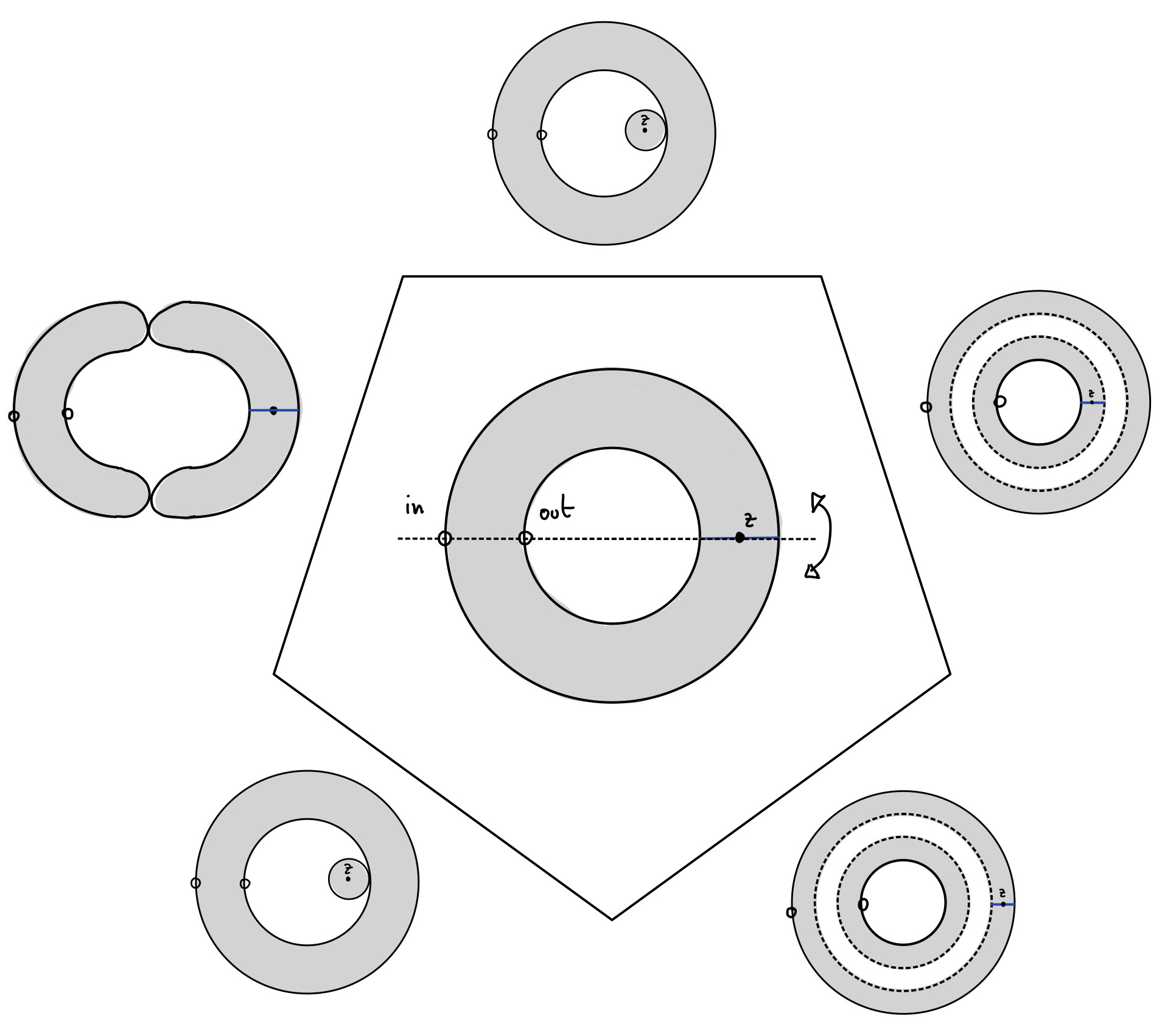
In this setting my additional symmetry appear as another symmetry along (for example) the plane $(x=0)$. Forcing this kind of symmetry (and taking the compactification) gives me a pentagon as a moduli space, where the two coordinates are basically the conformal parameter of the annulus and the position of the marked point $z$ along the blue segment. The boundary marked points $\text{in}$ and $\text{out}$ are not allowed to move.

My expertise in this specific topic is very limited and I'm trying to navigate through the literature a little. I definitely don't want to re-discover the wheel here, so I'm wondering if you're aware of works considering a similar situation to mine.

What I'm trying to understand here is whether I still obtain the structure of manifold with corners for my moduli space of symmetric configurations of my annulus $(A,\text{in},\text{out},z)$ as suggested by the picture.

As the tag suggests, my background is symplectic geometry, I would appreciate some reference in case of extensive use of algebraic geometry.