Suppose $S_t$ is distributed on $[0,1]$ with the pdf $$\frac{1+30\,t\,x^2(1-x)^2}{1+t},$$ which is one way to get a distribution as suggested in the comments with concentration in the middle of the interval. Then $$E[k-i|S_t]=\frac{462+1100t+791t^2+160t^3}{1848(1+t)^2(3+8t/5)}$$ and $\rho(\alpha|S_t)$ has a more complicated algebraic expression in terms of $\alpha$ and $t$.
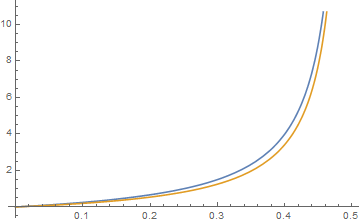
This allows us to show that the uniform distribution ($S_0$) does not minimize $\rho$, though it may come close. Specifically, $$\rho(\alpha|S_4)<\rho(\alpha|S_0)$$ for any $0<\alpha<1/2$. Here is a graph of those two functions:
This $\rho(\alpha|S_4)$ comes close to the minimum among all $\rho(\alpha|S_t)$ whenever $0<\alpha<1/2$. Probably there are families of distributions other than $S_t$ which would get lower values still.