Golla and Hayden gave awesome responses to the question. Their arguments can be generalized in the following fashion.
Following their notations, recall that $K_1$ is the figure-eight $4_1$ knot and in general $K_n$ is the twist knot $(2n+2)_1$ in $S^3$.
They together proved $$S^3_{1/3}(K_1) \cong S^3_{+1}(K_3).$$
Note that the right-hand side is the Brieskorn sphere $\Sigma(2,3,19)$.
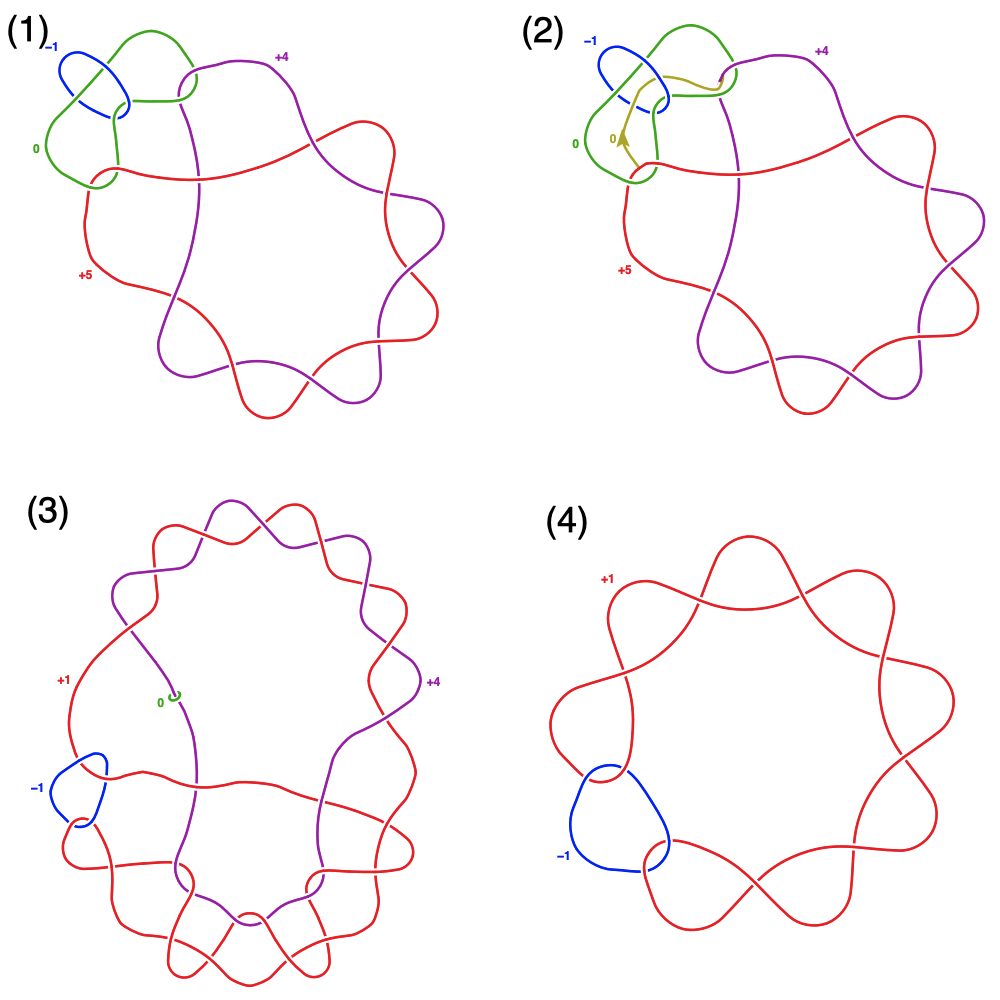
a- Handle diagrams of Golla is generalized to the next case as follows:
b- With the observations of Hayden, we have $$S^3_{1/4}(K_1) \cong S^3_{+1}(K_4).$$ There is a pattern in the Kirby calculus diagrams. Thus we may eventually prove that $$S^3_{1/n}(K_1) \cong S^3_{+1}(K_n).$$ Similarly, the right-hand side is the Brieskorn sphere $\Sigma(2,3,6n+1)$.
c- This part is about rational homology cobordism classes of $\Sigma(2,3,6n+1)$.
Definition: A knot $K$ in $S^3$ is called rationally slice if it bounds a smoothly properly embedded disk $D$ in a rational homology ball $X$.
Theorem(Kawauchi, (Kaw79) + (Kaw09)) Any hyperbolic amphichiral knot in $S^3$ is rationally slice. Consequently, $K_1$ is rationally slice in $S^3$.
Now we need an extra observation which is probably known to experts in low-dimensional topology and can be seen as the rational analogue of Gordon's theorem:
Lemma: For each $n$, $S^3_{1/n}(K_1)$ bounds a rational homology ball.
Proof: The figure-eight knot $K_1$ bounds a smooth disk $D$ in a rational homology ball $X$. The tubular neighborhood of $D$, $\nu(D)$, is $B^2 \times D$ in $X$.
Think $K_1$ and $D$ respectively as a belt sphere and co-core of $4$-dimensional $2$-handle $B^2 \times B^2$. So, we have $B^2 \times D = (X \setminus \nu(D))⋃ B^2 \times B^2.$
Now remove this $2$-handle and reattach it with a framing differing from the initial one by $n$ left-handed twists. Then the boundary $3$-manifold changes by $1/n$-surgery on $K_1$. Since we don't change the rational homology of $4$-manifold, we are done.
Therefore, we have a "theorem":
Theorem: For each $n$, Brieskorn spheres $\Sigma(2,3,6n+1)$ bounds a rational homology ball.
Remark: The cases $n=1$ and $n=3$ are known by Fintushel-Stern (FS84) and Akbulut-Larson (AL18). For the cases $n=2$ and $n=4$, they bound contractible $4$-manifolds due to classical results of Akbulut-Kirby (AK79) and Fickle (F84). Hence they a priori bound rational homology balls.