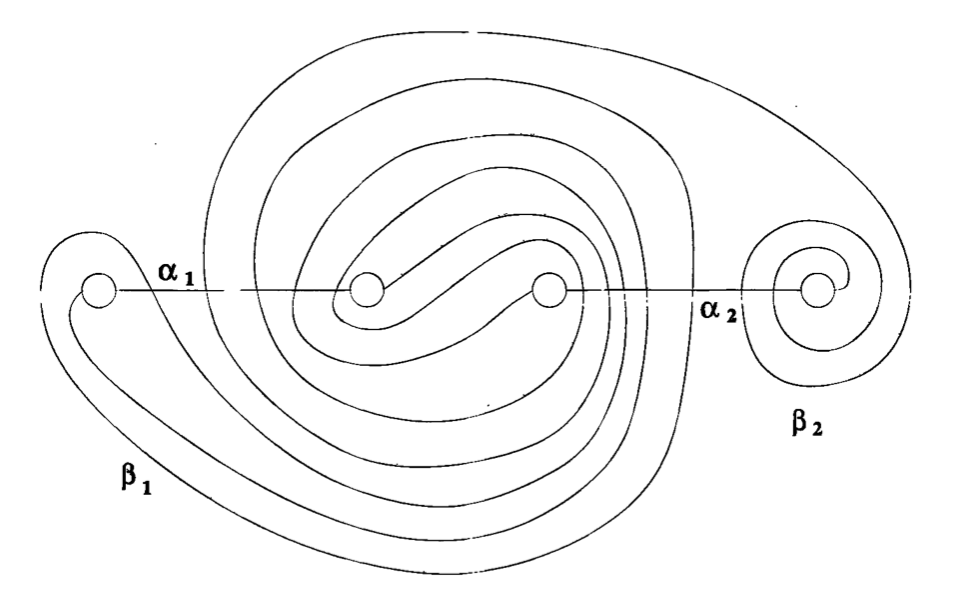
This is a late reply but it should be still helpful. It is from Zoltan Szabó's PCMI lecture notes.
Consider the following Heegaard splitting:
It is the genus $2$ Heegaard splitting for the $3$-manifold $W_2$.
To generalize this example to the family $W_n$, let us focus the $\beta_2$ cycle winding the right circle twice. Instead of twisting around the right circle two times, twist $n$-times to obtain the Heegaard splitting of $W_n$.
Let $K$ be the right-handed trefoil in $S^3$. Then show that
Let $Y$ be the $3$-manifold whose Heegaard diagram obtained by the Heegaard diagram of $W_n$ by omitting the $\beta_2$ curve. Then $Y$ is homeomorphic to $S^3 \setminus K$.
In general, $W_n$ is homeomorphic to $S^3_{n-4}(K)$, which is the $3$-manifold obtained by $n-4$-surgery along $K$ in $S^3$.
In particular, $W_3$ is homeomorphic to Poincaré homology sphere $\Sigma(2,3,5)$.
Further, $W_2$ is homeomorphic to $\Sigma(2,3,4)$ (Manolescu's example) and $W_1$ is homeomorphic to $\Sigma(2,3,3)$. The latter two are the boundaries of the plumbing graphs $E_7$ and $E_6$ respectively.