Let $\phi=(p\vee(q\wedge r))\wedge (r\vee(p\wedge q))$ and let $\chi = (p\wedge (r\vee (p\wedge q)))\vee (r\wedge (p\vee (q\wedge r)))$. For your De Morgan lattice ${\bf M}$ we have $\phi\models_{\bf M} \chi$, but we have $\phi\not\models_{\bf L} \chi$ for the De Morgan lattice:
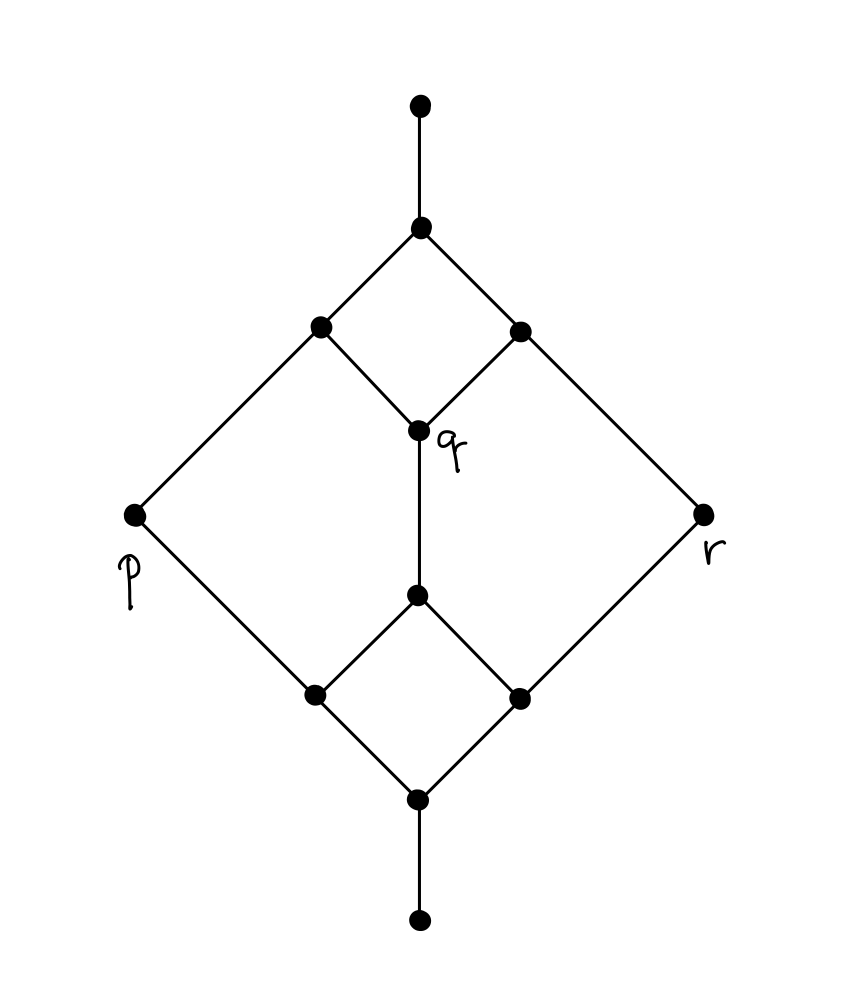
The complementation is defined by the self-duality that fixes $p$ and $r$.
This example is more complicated than Adam's example, but it has the property that it is purely about the underlying lattice.
The justification is this: Let ${\bf L}^*$ be ${\bf L}$ minus its top and bottom. ${\bf L}^*$ is the splitting lattice for the $p$-modular law, which is $\phi\leq \chi$. Any lattice either satisfies the $p$-modular law, or has a copy of ${\bf L}^*$ as a sublattice. Your lattice ${\bf M}$ doesn't have a sublattice isomorphic to ${\bf L}^*$, so it satisfies the $p$-modular law. On the other hand, ${\bf L}$ does not satisfy the $p$-modular law, as you can see by assigning to the variables $p, q, r$ the values indicated in the figure.
Notice that if ${\mathbf L}$ is any bounded lattice, then the ordinal sum ${\mathbf 1}+{\mathbf L}+{\mathbf L}^{\partial}+{\mathbf 1}$ has a proper De Morgan complementation satisfying all four bullet points of the problem. Any canonical example ${\mathbf M}$ would have to fail every lattice identity that failed this ordinal sum, hence every lattice identity that failed in ${\mathbf L}$. Since ${\mathbf L}$ is arbitrary, it follows that the underlying lattice of any canonical example would have to generate the variety of all lattices. Since your lattice is uniformly locally finite, it cannot generate the variety of all lattices.
