Return to Revisions

I would just approximate $\sin^2$ by 1/2, it oscillates rapidly and the average over one period should be a sensible estimate, then $$S(p,q,s)\approx \tfrac{1}{2}H_q^{(s)},$$ the Harmonic number. 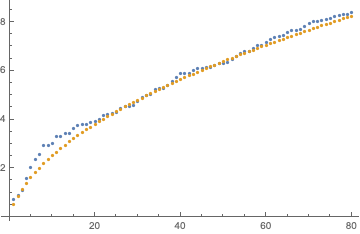For large $q$ and $s<1$ this gives $$S(p,q,s)\rightarrow q^{-s} \left(\frac{q}{2 (1-s)}+\tfrac{1}{4}+{\cal O}(q^{-2})\right)+\tfrac{1}{2}\zeta (s),$$ while for $s=1$ one has $$S(p,q,1)\rightarrow=\tfrac{1}{2} \ln q+\tfrac{1}{2}\gamma_{\rm Euler}+\frac{1}{4 q}+{\cal O}(q^{-2}).$$ Here is a comparison for $p=1$, $s=1/2$ (blue the exact sum, orange the large $q$ asymptotics).