The following is probably a bad question, but hopefully, it might have a very good answer.
In category theory there is a quite famous analogy between topoi and commutative rings, I was never convinced by this analogy, but the best way to see how far an analogy can be pushed is to challenge it. Clicking on the link that I provided above you can have an extensive presentation of the analogy, the general motto can be grasped by the following table.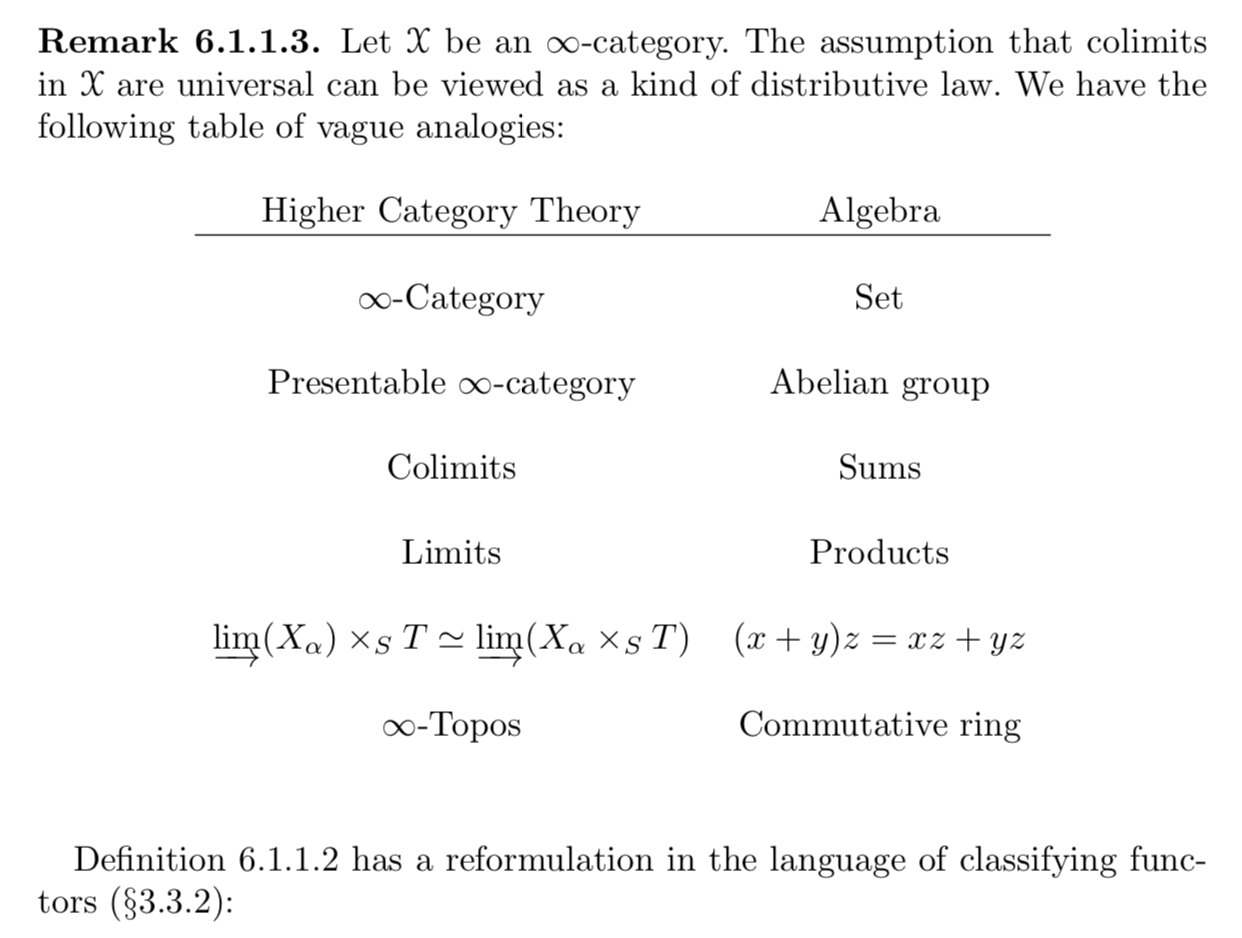
That correspond to Rem 6.1.1.3 of my version of HTT by Lurie.
Q. According to this analogy, what should be a field?
Maybe I should say why this might be a stupid question or even a stupid challenge for the analogy. In fact it might be the case that:
- The notion of field is interesting only in low dimension.
- The correct generalization of the notion of field looks very different in categories and trivializes for sets because of their intrinsic rigidity.
