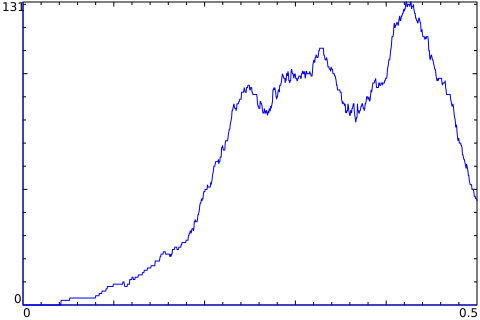
I gathered some statistics using the Cremona tables. There are 3337 elliptic curves with conductor $\leq 10^4$, rank 1, trivial torsion group and positive discriminant (which means that the group of real points has two connected components). Among them 446 have the generator of $E(\mathbf{Q})$ located on the identity component. This is not really enough to draw any conclusion, but here is the histogram representing the location of the generator $P$ on the identity component, in other words the real number $z_P \in (0,1/2)$ such that $E(\mathbf{Q})$ is generated by $z_P \Omega_E^+$, where $\Omega_E^+$ is the real period.
