Here, I try to give a exemple for each of the number one and three curves with a convex polyhedron where I just draw the net. The geodesic are the dots lines (which are staight on the net). I think that one can also construct the second one as well by changing the angles of the triangle in the first exemple.
I don't know any theorem that gives a general answer for your question. My only gess is that if you don't have a trivial estimate that says that on a subset $B$, $\int_B K \geq 4\pi $, then we should be able to construct a exemple.
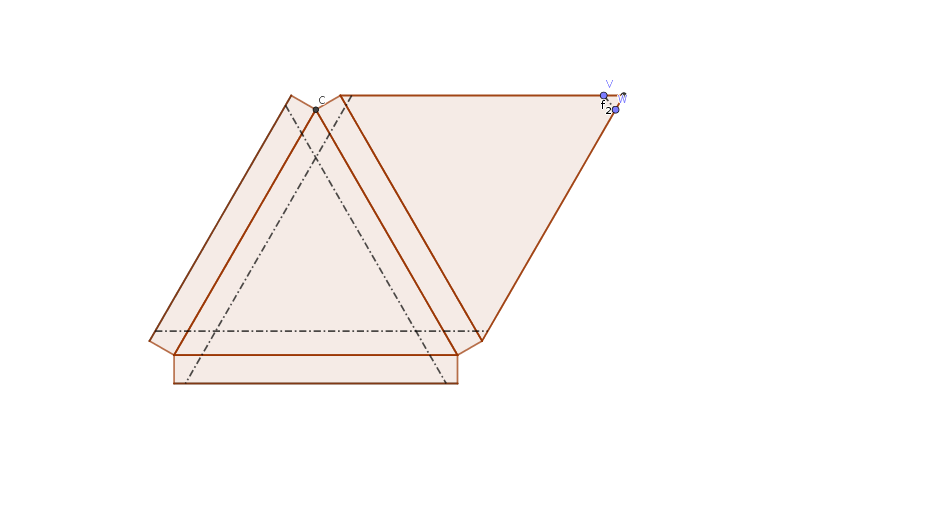
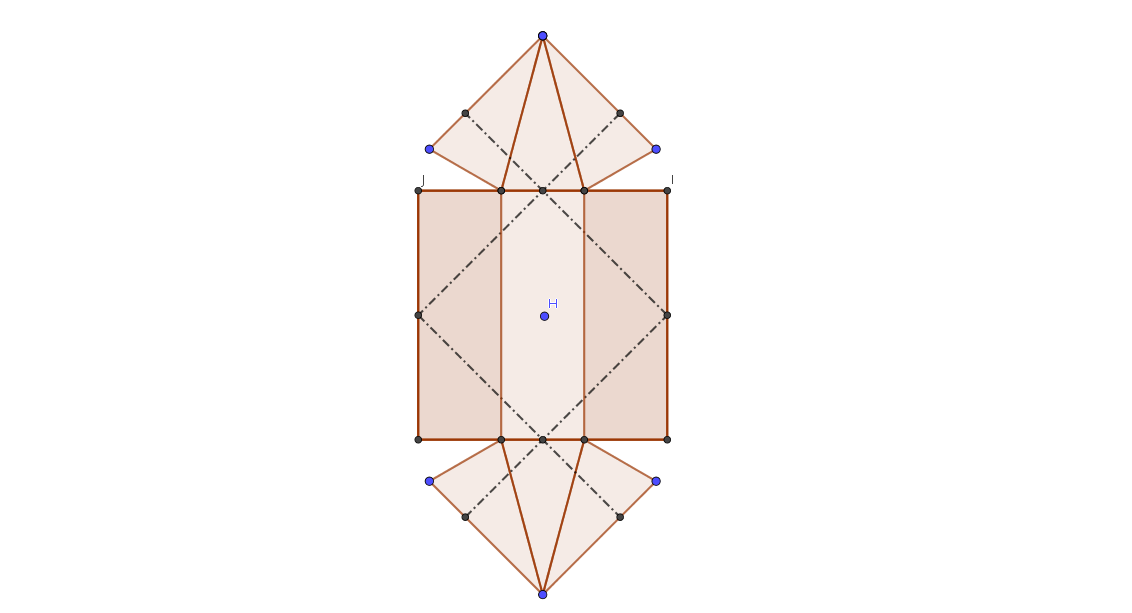
EDIT : I just give some details of what anton says in his comment : in each small loop the integral of curvature is $\pi+\alpha_i$ with $\alpha_i$ the angle of the crossing. Because the curvature is positive the sum of the angles of the triangle is larger than $\pi$ and then the total curvature is larger than $3\pi+ \sum \alpha_i > 4\pi$. (However with a polyhedron we can still obtain an equality)
