There are various ways of putting additional data on a fixed 2-complex and then defining a notion of "discrete conformal equivalence" between different assignments of data, see e.g. the answers to this question or the field of circle packing (mentioned in the comment of Thomas Kojar and the answer of Adam P. Goucher).
In some of these settings there do indeed exist local transformations which allow you to change the combinatorics of the 2-complex; e.g. one can subdivide triangles in a circle packing or perform star-triangle transformations on certain types of discrete Riemann surfaces, e.g. isoradial graphs or "surfel" surfaces.
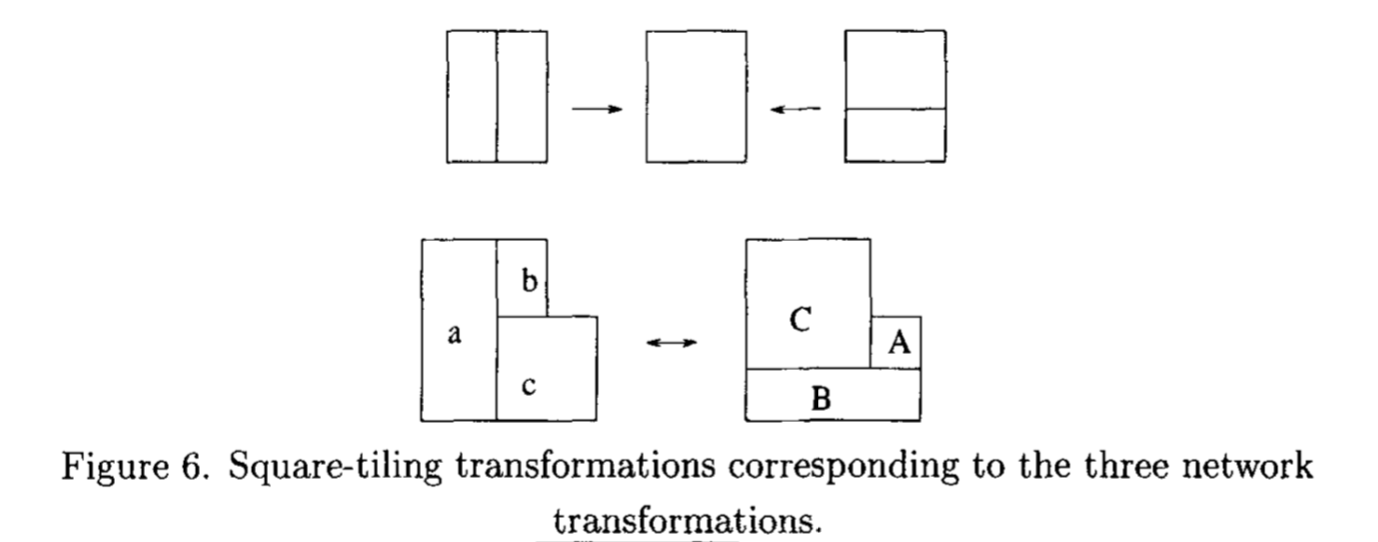
A bit more classically, tilings of rectilinear polygons by rectangles (à la Brooks, Smith, Stone, Tutte) can be viewed in this light as well (see e.g. "Squaring rectangles" by Cannon, Floyd and Parry). The rough idea is that rectangle tilings can be constructed from currents and potential differences in a resistor network; the potentials in a resistor network and the currents form a pair of conjugate harmonic functions. Then the classical electrical equivalence moves (including the "original" star-triangle transformation) lead to local transformations of square tilings which change the combinatorics; here are two figures from Kenyon's "Tilings and discrete Dirichlet problems".
First, a depiction of the transformations of the underlying resistor network:
And their realization as transformations of the rectangle tiling:
There are likely more examples. Unfortunately I don't know of an overarching framework which captures this phenomenon (nor even of an exhaustive survey), as this is a rather broad field with influences from conformal geometry, combinatorics, statistical physics, and computer graphics. The references given above are by no means meant to be complete or even representative.