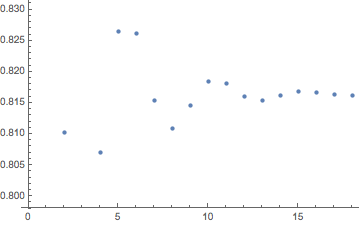
I understand from the OP that the motivation for this question is to find a series expansion in powers of $t$ of $$I(t)=\int _{0}^{t}\exp(-x^2 \operatorname{erf}(x))dx.$$ This follows directly from the series expansion of the error function, $$I(t)=\sum_{p=0}^\infty c_p t^p=t-\frac{t^4}{2 \sqrt{\pi }}+\frac{t^6}{9 \sqrt{\pi }}+\frac{2 t^7}{7 \pi }-\frac{t^8}{40 \sqrt{\pi }}-\frac{4 t^9}{27 \pi }+\frac{(\pi -28) t^{10}}{210 \pi ^{3/2}}+O\left(t^{11}\right).$$ The series for $t=1$ seems to converge to $I(1)=0.8164...$, at least that is what the numerics suggests: here is a plot of $\sum_{p=0}^{N+7} c_p $ as a function of $N$.