I asked a more general version of this question in an earlier question, Regions on a sphere that avoid a fixed point set.
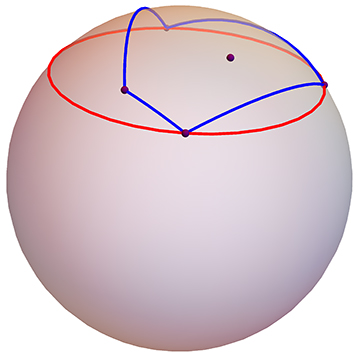
A $5$-point set, its convex hull (blue) and smallest enclosing circle (red).
The OP's version is an $8$-point set. An upperbound was proved: "for an $n$-point set $P$, the area of an avoiding set cannot be larger than $\frac{n-1}{n} A$, where $A$ is the area of the sphere." For $n=8$, this gives an upper bound of $\frac{7}{8}=0.875$ which is smaller than the OP's $0.9$.
To address the OP's specific questions:
I want to know where this question originates from.
My version was original, but ...
Is this question part of some general type of questions that are encountered in a more general setting?
Yes, avoiding sets. For example, there is quite a bit of literature on mutually avoiding sets, going back to Erdős. There is also considerable literature on sets avoiding integral (or rational) distances. See, e.g., the question Integer-distance sets. Or: Kurz, Sascha, and Valery Mishkin. "Open sets avoiding integral distances." Discrete & Computational Geometry 50.1 (2013): 99-123.
