I can muddy the waters...!
According to editor E. Scholz of Hausdorff’s Collected Works (2008, p. 884):
In a note of 3/20/1933 (Nachlass, fasc. 449) and in a further undated note (fasc. 571), Hausdorff symbolized the functoriality property of homology (in our later terminology) with a commutative diagram of homomorphisms between the terms of two sequences of groups $(A_n)_{n\in\mathbf N}$, $(A'_n)_{n\in\mathbf N}$:
(Nachlass, fasc. 571, leaf 1).
Could this have, somehow, made its way out of Bonn (where Hausdorff lectured on combinatorial topology that year) and to Hurewicz, Eilenberg, Steenrod, et al.? This seems not impossible, as e.g. Tucker (1932, footnote 4) mentions discussion of Hausdorff letters in the Princeton seminar. Maybe also noteworthy: Hausdorff’s colleague and mentor in Bonn was Eduard Study, author of another early commutative diagram.
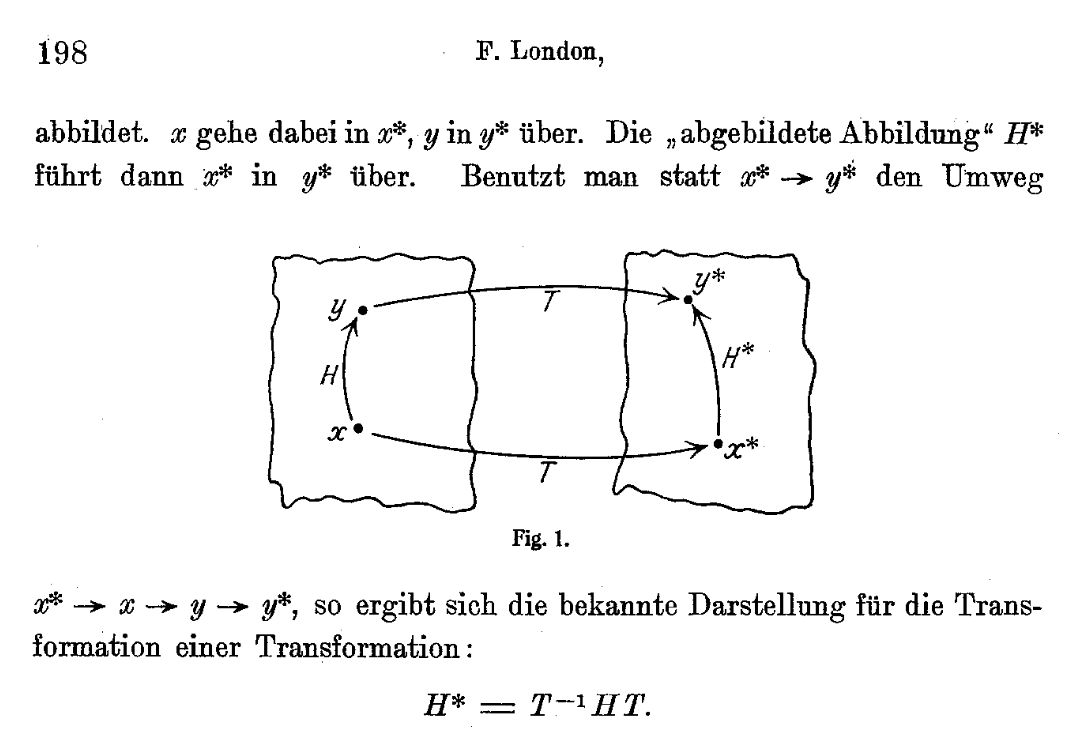
Addition: Six years earlier, F. London’s Winkelvariable und kanonische Transformationen in der Undulationsmechanik, Z. f. Physik 40 (1927) 193-210 already had this:
(For the story of the arrows themselves, see the relevant questions on $\to$ and $\mapsto$.)