Following up on Anthony Quas's post, let $g(x) = 4x(1-x)$ and $h(x) = \sin(\pi x)$. The equations $g^{(n)}(x) = 1/2$ and $h^{(n)}(x) = 1/2$ each have $2^n$ roots in $[0,1]$. If we sort those roots in order as $y^n_1 < y^n_2 < \cdots < y^n_{2^n}$ and $z^n_1 < z^n_2 < \cdots < z^n_{2^n}$, we should have $f(y_i) = z_i$.
To see that there is unlikely to be a closed form solution, note that $y^n_1$ behaves like $c_1 4^{-n}$ for some constant $c_1$ and $z^n_1$ behaves like $c_2 \pi^{-n}$. So we must have $f(y) \sim c_3 y^{\log(\pi)/\log(4)}$ as $y \to 0$. Similar arguments should show that we have $f(y^n_i+\delta) - z^n_i \sim c \delta^{\log(\pi)/\log(4)}$ as $\delta \to 0$ for every $y^n_i$ (with the constant $c$ depending on $(n,i)$). This is not how a nice function behaves!
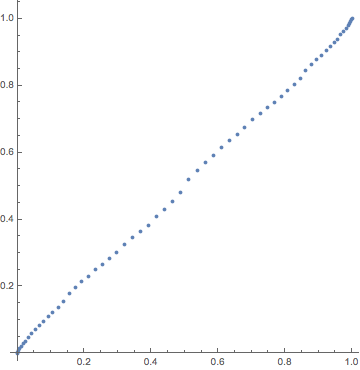
Here is a plot, and list of values, for $n=6$:
0.0002 0.0005
0.0014 0.0033
0.0038 0.0079
0.0074 0.0132
0.0121 0.0211
0.0181 0.0282
0.0252 0.0369
0.0335 0.0465
0.0429 0.0604
0.0534 0.0716
0.0650 0.0832
0.0776 0.0942
0.0912 0.1092
0.1058 0.1220
0.1214 0.1375
0.1379 0.1546
0.1552 0.1790
0.1734 0.1974
0.1924 0.2153
0.2121 0.2310
0.2325 0.2505
0.2536 0.2659
0.2752 0.2833
0.2974 0.3012
0.3201 0.3262
0.3432 0.3454
0.3666 0.3651
0.3904 0.3835
0.4145 0.4082
0.4388 0.4292
0.4632 0.4543
0.4877 0.4813
0.5123 0.5187
0.5368 0.5457
0.5612 0.5708
0.5855 0.5918
0.6096 0.6165
0.6334 0.6349
0.6568 0.6546
0.6799 0.6738
0.7026 0.6988
0.7248 0.7167
0.7464 0.7341
0.7675 0.7495
0.7879 0.769
0.8076 0.7847
0.8266 0.8026
0.8448 0.821
0.8621 0.8454
0.8786 0.8625
0.8942 0.878
0.9088 0.8908
0.9224 0.9058
0.9350 0.9168
0.9466 0.9284
0.9571 0.9396
0.9665 0.9535
0.9748 0.9631
0.9819 0.9718
0.9879 0.9789
0.9926 0.9868
0.9962 0.9921
0.9986 0.9967
0.9998 0.9995