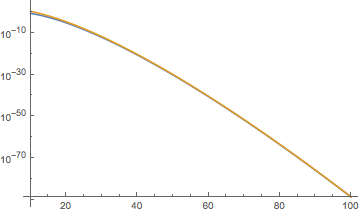
We could just take the large-$n$ asymptotic of $J_n(z)\rightarrow (2\pi n)^{-1/2}(ez/2n)^n$, and then
$$f_n(\rho)\rightarrow \frac{1}{n}(\tfrac{1}{2}e\rho/n)^n.$$
This seems to be quite reasonable (blue is the exact result, gold is the asymptotic expression, plotted as a function of $n$ for fixed $\rho=10$):