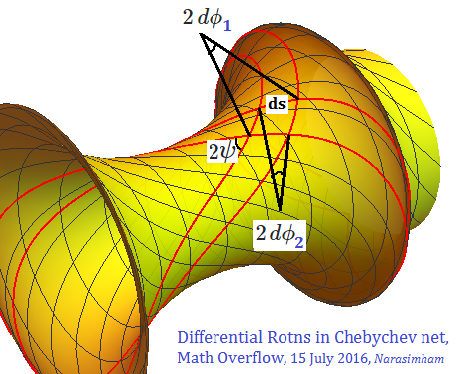
A Chebyshev net obeying Sine-Gordon equation is drawn on a surface of constant negative Gauss Curvature $K$ so that the asymptotic differential rhombic element corners lie on lines of maximum/minimum normal curvature.

Show that principal rotation of surface normals across diagonals ($\phi_1$ = const, $\phi_2 $ = const.) of rhombus are related as:

$$ d \phi_1^2 + d \phi_2^2 = - K ds^2 \tag{0} $$

which is a hyperbolic metric with rotation $\phi_1,\phi_2$ as parameters.

EDIT1:

The following fully reinforces original view of hyperbolic geometry where the parameters $ (\phi_1, \phi_2 )$ take the place of $(u,v)$ in the Euclidean metric upto an invariant constant $a$ :

$$ ds^2= du^2 + dv^2 \tag{1} $$

Let $ K= -1/a^2$ , primes with respect to hyperbolic geodesic asymptotic arcs

$$ \phi_1^{\prime2} +\phi_2^{\prime2} = \frac{1}{a^2} \tag{2}$$

Taking components of arc along direction inclined at $\psi$ to fiber along maximum and minimum curvature directions we have by definition of constant $K$:

$$ \frac{d \phi_1}{ds \cos \psi} \cdot \frac{d \phi_2}{ds \sin \psi} = \frac{ \phi_1 ^{\prime}}{ \cos \psi} \cdot \frac{ \phi_2 ^{\prime}}{ \sin \psi} =\frac{1}{a^2} \tag{3} $$

Solving (2),(3) we obtain derivatives of rotation w.r.t. arc in each direction as:

$$\phi_1^{\prime} = \frac { \cos \psi} {a}, \;\phi_2^{\prime} = \frac { \sin \psi} {a} \tag{4} $$

as one solution taken out of two interchangeable solutions. Squaring and adding,

$$ \boxed{ds^2 = a^2( d \phi_1^2 + d \phi_2^2) } \tag{5} $$

What prompts me to post this is: Recognition of this observed identity between Euclidean and Hyperbolic parameters to hopefully remove vagueness (in my mind at least) while recognizing these rotations as hyperbolic parameters:

Thus the above is the curvilinear hyperbolic geodesic Pythagoras theorem. Hypotenuse is allowed only along hyperbolic geodesics and components only along maximum/minimum normal curvature lines.

I have no access to good literature references/ sources but had held this view within myself that... this was Beltrami's original conceptualization.Request enlightened members to please make corrections and give comments on my view.

$$ (u,v) \leftrightarrow a (\phi_1 , \phi_2 ) \tag{6} $$

In this connection I quote from the text book authored by DJ Struik, Lectures on Classical Differential Geometry, Second edition pp 153 left bottom:

The whole geometry of Lobachevski-Bolyai could thus be interpreted on a surface of constant negative curvature , parallel lines becoming geodesics (Emphasis mine). Beltrami proved that the consistency of implied consistency of Lobachevski-Bolyai geometry, since an inconsistency in the latter could be interpreted as an inconsistency in the theory of surfaces of constant negative (Gauss) curvature which itself is based on Euclidean postulates.

Above image is made on Mathematica based on the metric correspondence (6). The discussion is for any surface, not necessarily that of revolution as pictured.

EDIT2:

Derivation:

$ \kappa_{1,2}$ principal curvatures. Euler's normal curvature relation:

$$ \kappa_n =\kappa_1 \cos^2 \psi + \kappa_2 \sin ^2 \psi =0 ;\, \kappa_1 \kappa_2 = -1/a^2 \, \rightarrow \kappa_{1,2}= (-\tan\psi/a, \cot\psi/a) \tag{7}$$

Line segment components along curvature extrema directions :

$$ 2 \, d \phi_1 = 2 \, ds\, \cos \psi \, \kappa_1,\, 2\,d\phi_2 = 2 \, ds \, \sin \psi \, \kappa_2,\ \tag{8} $$

Combining (7),(8) to eliminate $\kappa_{1,2}$ we get (5) or (0).

EDIT3:

Constant negative hyperbolic lines $K$ in the large for orthogonal lines should accordingly be given by:

$$ \cosh (ds/a) = \cosh d \phi_1\, \cosh d \phi_2\ $$

and in the small by

$$ 1 + (ds/a)^2/2 \approx (1 + d\phi_1^2/2)\,(1 + d\phi_2^2/2) $$

so that we have same:

$$ ds^2 = a^2(d\phi_1^2 + d\phi_2^2 ). \tag{10=5} $$