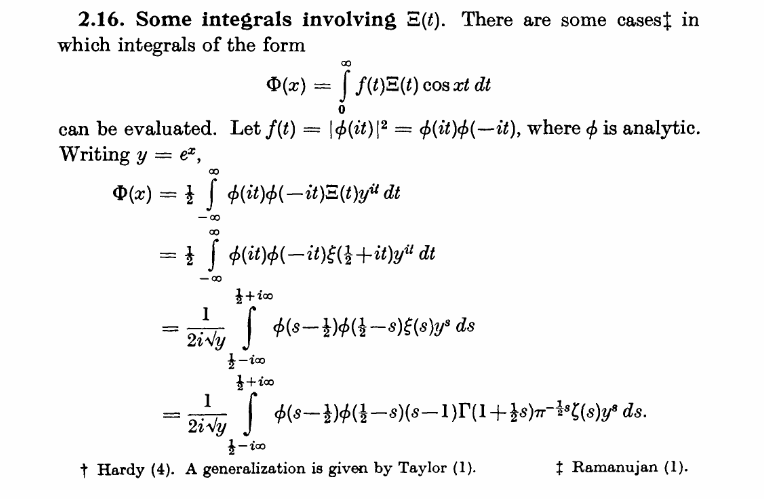
Story

I'm trying to prove following identity.

$$\int_0^\infty \frac{\Xi(t)}{t^2 + \frac{1}{4}} \cos(xt) dt = \frac{1}{2} \pi (e^{\frac{1}{2}x} - 2e^{-\frac{1}{2}x} \psi(e^{-2x}))$$

where

$$\psi(x)=\sum_{n = 1}^{\infty} e^{-n^2 \pi x},$$

and

$$\Xi(t) = \xi(\frac{1}{2} + it)$$

is the xi function on the critical line.

Problem

I only don't understand the following equality:

$$-\frac{1}{4 i \sqrt{y}} \int_{\frac{1}{2} - i\infty}^{\frac{1}{2} + i\infty} \Gamma(\frac{1}{2}s) \pi^{-\frac{1}{2}s} \zeta(s) ds = -\frac{\pi}{\sqrt{y}} \psi(\frac{1}{y^2}) + \frac{1}{2} \pi \sqrt{y}.$$

Where does the $\frac{1}{2} \pi \sqrt{y}$ come from? When I substitude with $s = 2w$ I only get the first summand. We know that by Mellin transform theorem \psi(x) can be recovered by the Mellin transform integral over $\Gamma(s) \pi^{-s} \zeta(2s)$.

Source

This is from Titchmarsh's book "The Theory of the Riemann Zeta-function" p. 35−36