I find the problem of hearing the shape of a drum fascinating. Specifically, given two connected subsets of $\mathbb R^2$ with piecewise-smooth boundaries (or a suitable generalization to a riemannian manifold) it is in general impossible to use the spectrum of the laplacian to uniquely identify the shape of the domain.

As a simple example, reported in the Wikipedia link above, these two shapes

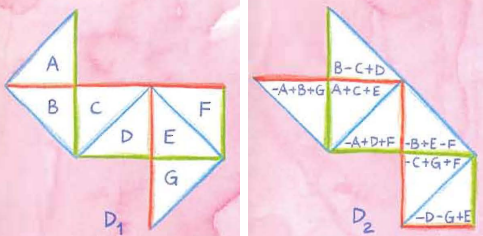
have identical laplacian spectra, as explained more at length by Gordon and Webb in American Scientist 84 no. 1, 46 (1996) (jstor). This can be shown by taking any eigenfunction $\varphi$ on $D_1$, cutting it up into the marked triangle regions, and taking the specified linear combinations on $D_2$ to make a laplacian eigenfunction with the same boundary conditions, correct matching at the internal boundaries, and the same eigenvalue.

What bugs me about this problem is that the spectrum is not really what you hear on a real-world drum. More specifically, the spectrum gives you the frequencies of the fundamentals and overtones that the drum is allowed to produce, but it does not tell you in what proportions it will do so. If you hit a circular drum in the centre, you will excite a large amplitude of the fundamental, resulting in a deeper sound, but if you drum it near the edge you will produce a rapping sound with a stronger proportion of higher overtones.

The set of sounds that a drum can produce is therefore better modelled by the pair of

• its Laplace-Beltrami spectrum, together with
• the set of eigenfunction-weight sequences $\{ (|\varphi_n(x)|)_{n=1}^\infty : x\in D\}$, with $\varphi_n$ the Laplace-Beltrami eigenfunctions. (A global normalization factor on each $(|\varphi_n(x)|)_{n=1}^\infty$ is obviously ignorable.)

In this paradigm, two isospectral surfaces $D_1$ and $D_2$ would still be 'acoustically distinguishable' if, say, $D_1$ contains a point $x^*$ such that the sequence of eigenfunction weights $(|\varphi_n^{(1)}(x^*)|)_{n=1}^\infty$ at $x^*$ is not matched by the eigenfunction weights $(|\varphi_n^{(2)}(y)|)_{n=1}^\infty$ of any $y\in D_2$. In other words, you can't see the drum, and you can't see where the drummer is hitting it, but if drummer 1 can produce a sound that drummer 2 cannot, then the drums must be different.

(Alternatively, one might have access to a function $u\mapsto (|\varphi_n(f(u))|)_{n=1}^\infty$, where $u$ lives in some standard domain $D_0$ like the unit disk and $f:D_0\to D$ is an unknown (homeo/diffeo/etc)-morphism. That is, you get a control that specifies where the drum should be hit, but you don't know exactly what it does. You can then say two drums $D_1$ and $D_2$ are indistinguishable if there's a morphism $g:D_1\to D_2$ such that $(|\varphi_n^{(2)}(g(x))|)_{n=1}^\infty=(|\varphi_n^{(1)}(x)|)_{n=1}^\infty$ for all $x\in D_1$, i.e. a mapping from each point $x$ in $D_1$ to an identically-sounding drum point in $D_2$.)

So, to come to my question: has this paradigm been explored in the literature? For the known isospectral-but-not-isomorphic regions (or more complex manifolds), are any pairs known to be "acoustically (in)distinguishable"? Does this concept have an established name in the literature? If it has been explored, are there good introductory surveys (say, pitched at a level where a physicist might understand them)? If it hasn't, are there fundamental reasons why this is a much harder problem overall?