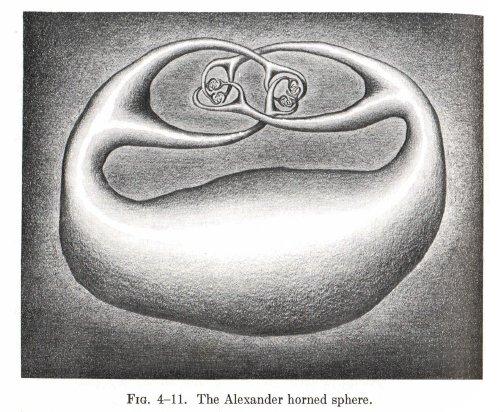
The Schoenflies conjecture was asserting that the two complements of an embedded $2$-sphere $S^2$ in $S^3$ were simply connected. A kind of generalized Jordan theorem.
Antoine's necklaces gave a first counterexample, and that counter-example was reworked by Alexander to obtain the horned sphere :
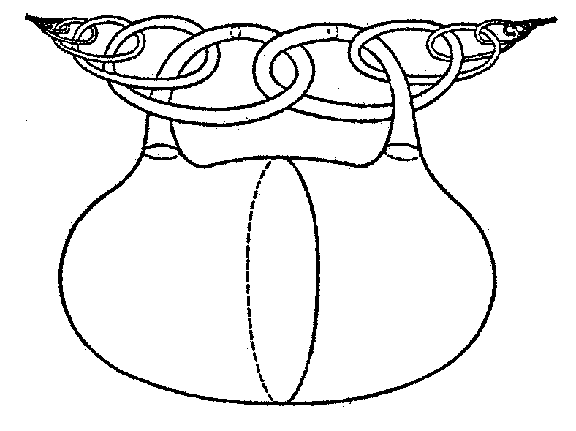
In this counterexample, the set of singualar points of the embedding is a Cantor set, so is quite big. Later, Artin and Fox developped the notion of wild arcs, and found the following simpler counterexample, where there are only two singular points :