Take $\chi(s)= 2^s\,\pi^{s-1}\,\sin\left(\frac{\pi\,s}{2}\right)\,\Gamma(1-s)$, so that $\zeta(s)=\chi(s)\,\zeta(1-s)$.

The zeros of $\chi(s)=-1$ and the non-trivial zeros $\rho$ of $\zeta(s)$, seem to have a remarkable correlation, although the zeros of the first are distributed much more regularly than the $\rho$s. Answers to this question, strongly suggest that it can be proven that all zeros of $\chi(s)=\pm1$ are on the critical line.

I wondered whether the zeros of $\chi(s)=-1$ could be 'morphed' into the $\rho$s by replacing the $-1$ by a function of $s$. The most obvious choice is to replace $-1$ by $\frac{\zeta(s)}{\zeta(1-s)}$, but this immediately destroys the $\rho$s.

However, when replacing $\frac{\zeta(s)}{\zeta(1-s)}$ by their finite Euler products with $p_n$ is the $n$-th prime, I got:

$$\chi(s)+\prod_{n=1}^N \left( \dfrac{p_n^{s}- p_n^{2s-1}}{p_n^{s}-1} \right)=0$$

and this yielded an encouraging outcome that is illustrated in the graph below ($N=47$, $y$ is absolute):

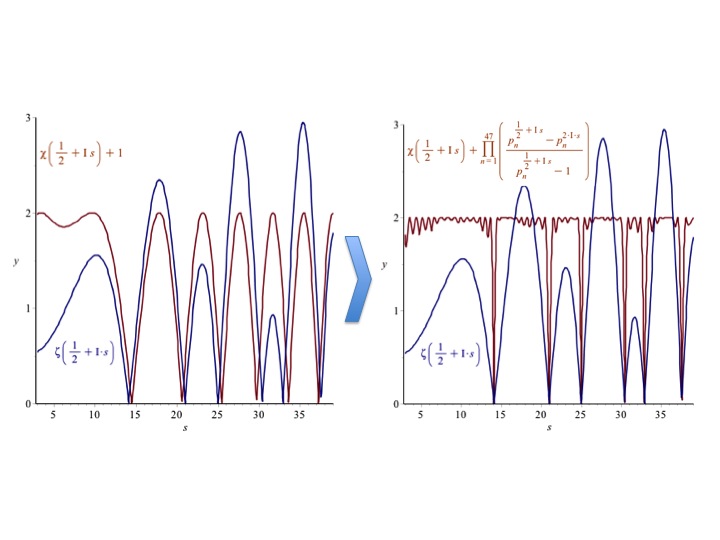
There is a snag though, since there now also exist other complex zeros at the sides of the strip. The good news is that these zeros are all clustered around the lines $\Re(s)=0$ and $\Re(s)=1$ and seem to get even more 'attracted' to these lines for increasing $N$. See graph below ($N=13$):

Question:

I observed that for each $N$ (even only using $p_1=2$), the zeros in scope all firmly appear to reside on the critical line. Could it therefore be proven that an infinite number of zeros of e.g.:

$$\chi(s)+ \dfrac{2^{s}- 2^{2s-1}}{2^{s}-1} =0$$

must reside on the critical line?

P.S.: Just a 'trivial' observation:

When we would take 'prime' $p=1$, we do get all the trivial zeros of $\zeta(s)$. Only for the real primes, the non-trivial zeros are induced and apparently moving closer to the $\rho$s for incremental $p_N$.