Imagine the elements of a group-like structure as puzzle pieces with essential two sides, an IN-side and an OUT-side.
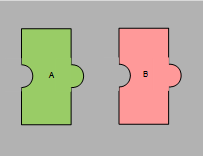
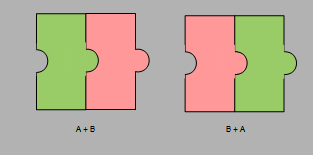
You can compose two such pieces in two obvious ways:
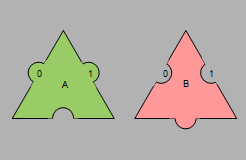
Now consider triangular puzzle pieces with at least one IN- and one OUT-side. These are 2-simplices with a non-trivial partition of their sides.
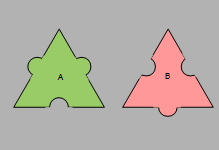
As long as two sides of the same kind are not distinguished (i.e. the simplices are symmetric), there are again two ways to compose two such elements:

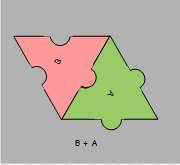
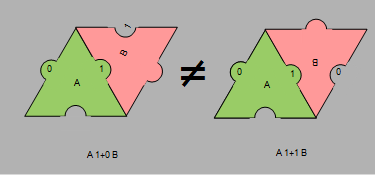
But when two sides of the same kind are distinguished:
a single operator + doesn't suffice anymore. One has to specify which of the (eventually) two OUT-sides of the first piece is to be plugged into which of the (eventually) two IN-sides of the second piece:
I wonder:
(1) In which specific (algebraic or simplicial resp. topological) contexts do such asymmetric pieces appear?
(2) How then is the problem of notation solved, especially: how are "words" (conglomerates) of such pieces symbolically written down (which is trivial for group-like structures and symmetric simplices by the use of + or $\circ$ or even no symbol at all).
Note that the composition is supposed to be in a natural way associative.
A related question concerns the possibility that cycles are allowed.

For group-like structures, cycles are not allowed (and in the rigid picture of puzzle pieces cycles are not even possible), for simplex-based structures cycles are supposed to be allowed:
(3) How is the problem of notation solved for possibly circular conglomerates?
