I recall a discussion on this question on math.sci.research quite a few years ago. John Conway had tried various moves to supplement Reidemeister moves. If I recall correctly, his guess was that no finite collection of moves will give monotonic simplification.
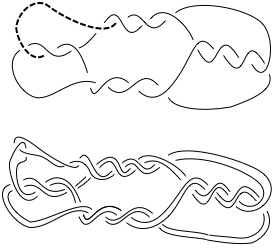
The Goeritz unknot below admits a simplifying move along dashed the arc shown. If one takes its untwisted double (as Ryan suggested) one again gets an unknot. (I hope I got the twists right below, but if not then adding twists shouldn't hurt). I don't see any obvious simplifying moves, or even any helpful lateral moves, for the bottom diagram.
Added: Marco correctly points out that while the doubling construction destroys the simplification at top left, it does not kill the one at bottom right. There is nothing to stop one from doing an additional untwisted doubling, with the clasp put at lower right. Perhaps that will do the trick.