Michael Ghrist's web site has some interesting notes on robotics and algebraic topology:
My work focuses on those methods in applied mathematics which are topological in nature. Such methods have the feature of being very robust: topological results are tolerant of the "noise" inherent in physical systems. Such techniques are therefore both elegant and effective in engineering and science.
I first came across him reading in the Notices about the theory of barcodes and persistent homology.
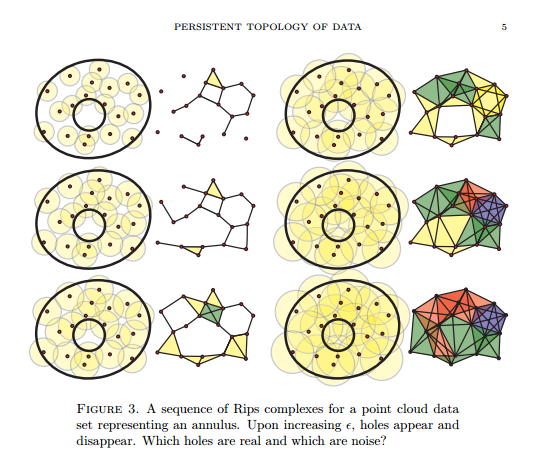
Currently, a lot of tools only use a 1D graph theory based approach. Using the Rips complex, a tool from geometric group theory, shows how to find topological features in data sets made of discrete points. Somehow we have to "complete" the point-set into a topological space.
There is an even more direct example of Grothendieck's influences in the theory of sensor networks.
