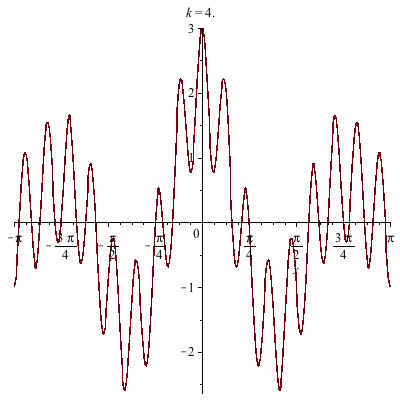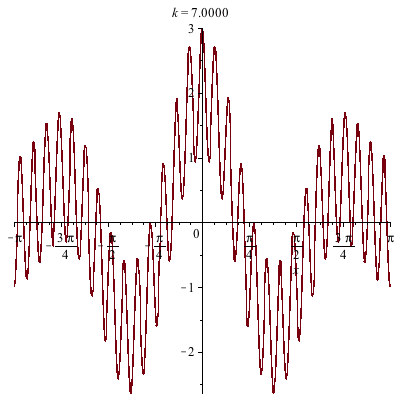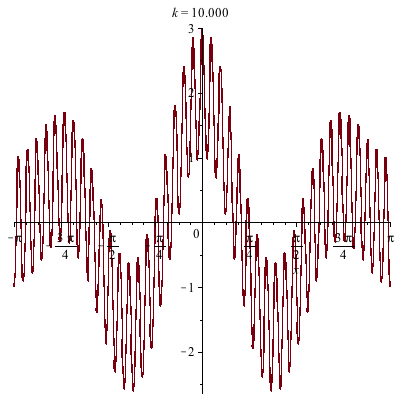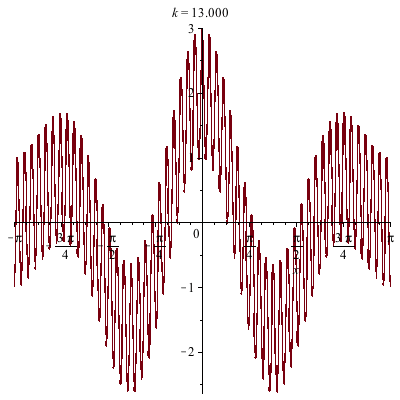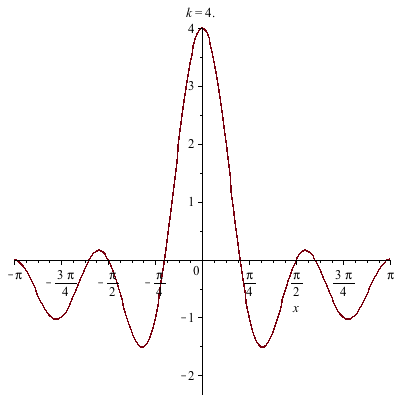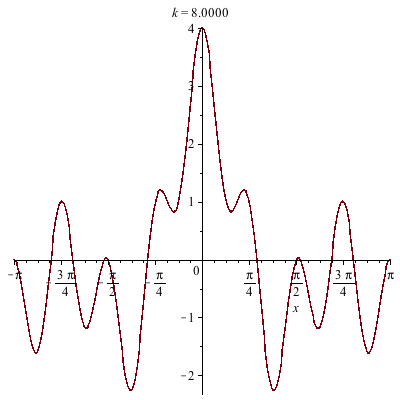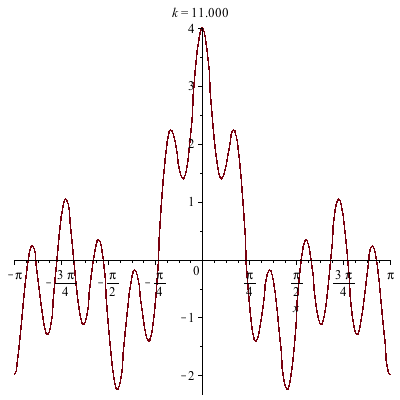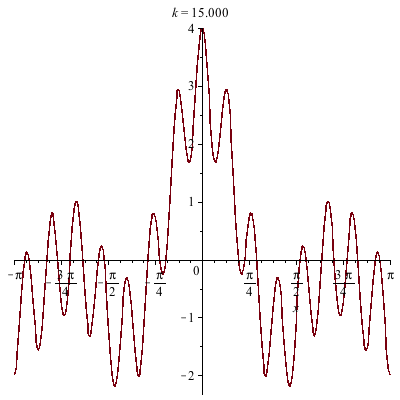
Above you see a $12$-frame animation of the family of trig polynomials
$$ P_k(t) =\cos t+\cos 2t+\cos 3t+\cos kt,\;\;\; k=4,\dotsc, 15. $$
I have included it to illustrate the fact that there seems to be another quantity relevant to your question, besides $n$, namely the degree $d=\max\{k_i;\;\;i=1.\dotsc, n\}$. In the above example $n=4$, but the degree varies from $4$ to $15$.
The next animation may be more suggestive because you can see large intervals where the trig polynomials are negative. More precisely, below is a $10$-frame animation of the trig polynomials
$$P_k(t) =\cos 2t+\cos 3t+ \cos(4k+1)t, \;\;k=4,\dotsc, 13. $$