In Waldhausen's Algebraic K-Theory of Spaces, he defines a cylinder functor on a category $\mathcal C$ with cofibrations and weak equivalences (henceforth called a Waldhausen category) as the following:

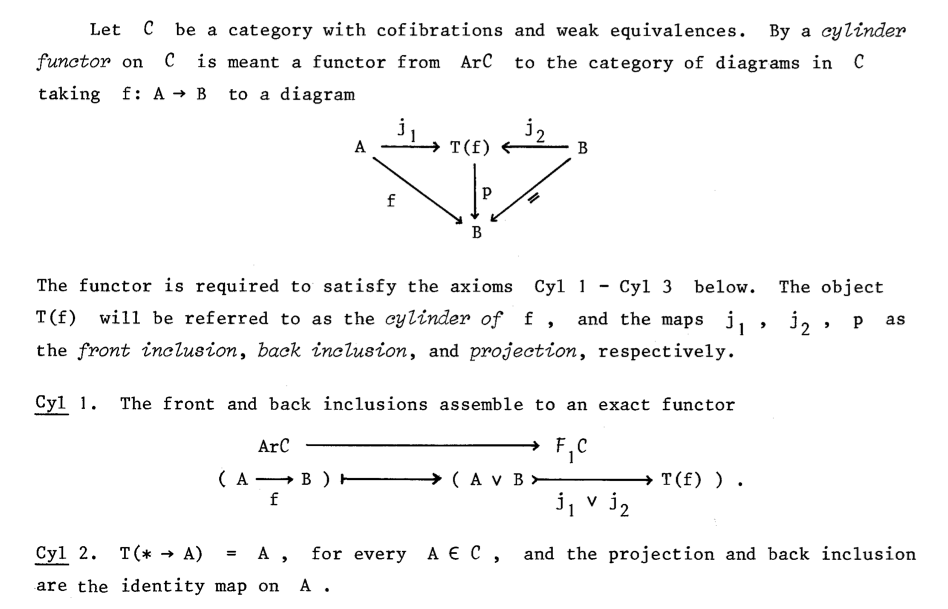
($F_1\mathcal C$ is the category of cofibrations in $\mathcal C$.)

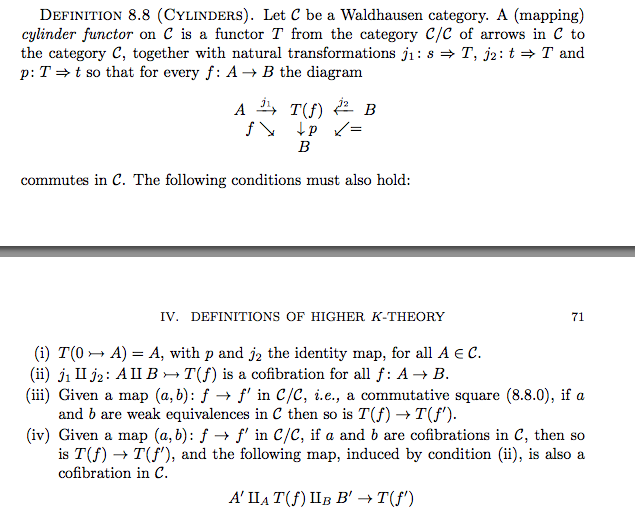
Weibel in his K-book defines it like this:

Finally, Gunnar in these online notes defines it like this:

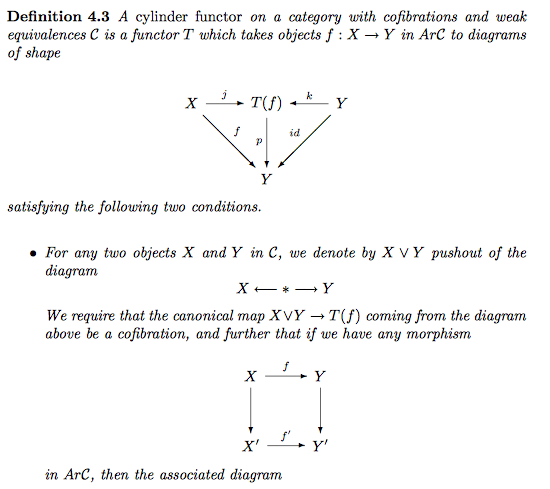
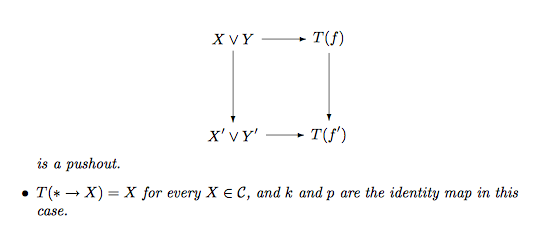
It's not evident to me that these definitions are all equivalent. Waldhausen's one is possibly the most concise. At first I thought that Weibel was fleshing out what it means for that functor in "Cyl 1" to be exact. If this is to be the case then condition iv. of Weibel should reformulate the fact that the functor of "Cyl1" takes pushouts along cofibrations to pushouts along cofibrations (one of the conditions on the definition of "exact functor"), but it isn't apparent that this is the case, if only for the trivial fact that a pushout in $Arr \mathcal C$ involves more arrows than there are on condition iv.

Gunnar's definition is closer in spirit to Weibel, but it's not really the same either. The map that iv. of Weibel asserts to be a cofibration in the case that $a, b$ are cofibrations, Gunnar's definition takes it to be an isomorphism for any $a,b$... Gunnar doesn't mention weak equivalences, either.

How do these definitions relate to each other?