Return to Revisions

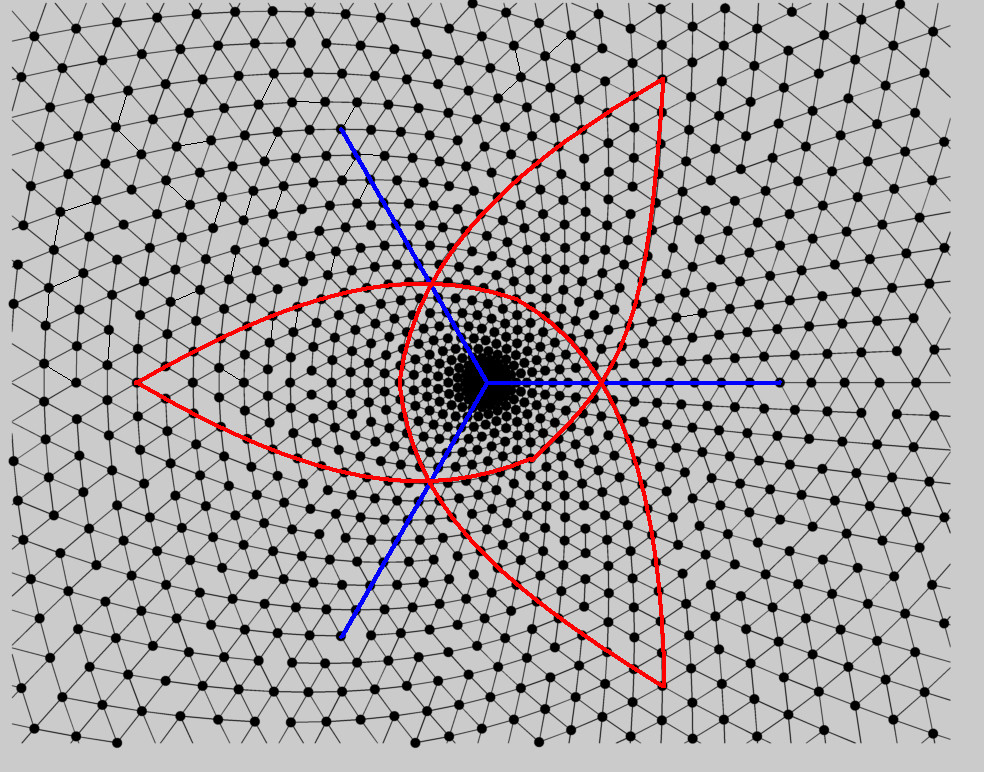
As we can see in the picture, here are the rules.

1. The pattern has the symmetries of a triangle. I made with blue three rays starting at the origin, and making equal angles.
2. For each blue ray, there is an array of parabolas which are symmetric with respect to that ray.
3. The parabolas symmetric with respect to the same line are distributed so that the distance between the apexes of two consecutive parabolas is larger, when the distance to the center is larger. This follows from the complex map used.
4. Also, the parabolas are larger as the distance to the center increases.
5. The dots are at the intersections between pairs of parabolas.

Now, that we know the rules, it is pretty straightforward to generalize to $\mathbb R^3$.

1. Take a regular tetrahedron, with the center in the origin.
2. Draw four blue rays, starting from origin, and going through the four vertices of the tetrahedron.
3. Build sequences of paraboloids which have as revolution axes the blue rays.
4. Make sure the paraboloids are at the same distances, and have the same ratios, as in the planar case.
5. Draw the dots at the intersections made by triples of paraboloids.