As we can see in the plot below, Mertens function: $M(x)\equiv \sum_{n=1}^{x}\mu(n)$ has wild swings from positive to negative and back again.

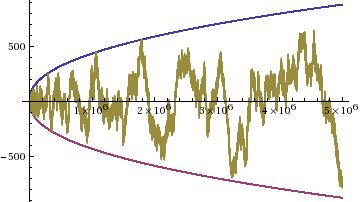
When we use: $$x=\frac{1}{2+\frac{1}{\phi+2}}\text{, where }\phi \text{ is the golden ratio,}$$ as the power for each $n$ we get the blue line and using its negative we get the red line. Both lines hug the extremes of the sum quite nicely. I have checked to about $10^{10}$ without finding any exceptions.

I have found only one reference to $\phi+2$ in the literature, specifically in "Mathematical Constants" by Steven R. Finch, page 418, where it is shown as the $\text{Tutte-Beraha constant} B_{10}$.

My question is: could $\phi+2$ be used to explain the behavior of the Mertens function? Or would this be a case of the Law of Small Numbers?