I will as usual act as Devil's advocate (or cubical advocate) by saying that there are some things we can do with cubical sets (with connections) which we cannot (maybe others can!) do with simplicial sets, namely:
Algebraic inverses to subdivision (multiple compositions);
Tensor products.
These properties are exploited and used (paradigmatically!) in the book Nonabelian algebraic topology: there is a Higher Homotopy Seifert-van Kampen Theorem, and some theorems on homotopy classification of maps (in the non simply connected case), and so rewrite a portion of algebraic topology without using singular homology or simplicial approximation. Also these results would not, I believe, even have been conjectured simplicially.
This is not to deny the advantages of the simplicial approach, which include its large literature, or some disadvantages of the cubical approach.
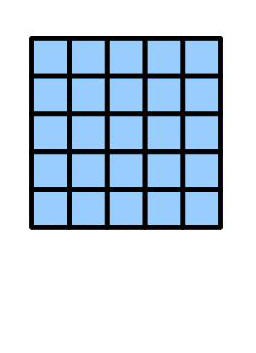
I started in the 1960s with drawing many times the diagram of a subdivided square pictured as
(see also Cubical vs. simplicial singular homology ) and saying to myself: surely there should be some mathematics which expresses that? Then Ehresmann's book "Catégories et structure" came out and the answer was clear: double categories (or groupoids)! In these one can describe the above as an array $(a_{rs})$ or as a composition $[a_{rs}]$ and this makes sense for the singular cubical complex, and also for double categories or groupoids. So one expresses "algebraic inverses to subdivision", while the corresponding globular or simplicial expressions are difficult or not available.
This role of cubical sets for intuition and conjecture is also suggested by the fact that Dan Kan's first results were cubical. Then problems were found with realisations and so, instead of trying to fix the cubical, they simply gave it up.
We came across connections for cubical sets for other reasons, namely to describe commutative cubes in terms of double groupoids.
January 9, 2015: See also this presentation at Galway, Dec, 2014, on "A homotopical approach to algebraic topology via compositions of cubes". Note that a simplicial approach of a similar type seems a non starter. However many aspects of a cubical approach have not yet been developed, because of the apparent success of the simplicial methods.
November 3, 2017 I can now refer to a more developed presentation of the philosophy of, background to, and development of the cubical approach in a paper Modelling and Computing Homotopy Types: I to appear in Indagationes Math. in 2017 in a special issue to honor L.E.J. Brouwer. One main point is that the cubical theory has been enriched by the notion of connections which rescue it from some problems of the more usual theory, and which the make the expanded theory applicable in places not available for the simplicial theory.