Can Morley's theorem be generalized?

Question

Morley's theorem states that in any triangle, the three points of intersection of the adjacent angle trisectors form an equilateral triangle.

In a talk some years ago, David Rusin made the provocative claim that Morley's theorem is a rare example of a striking theorem that defies generalization. The first ideas that come to everyone's mind—passing to higher dimensions or hyperbolic geometry for example—don't work.

The proof by Alain Connes yields a mild generalization of sorts, but not a very satisfying one in my opinion. Wikipedia claims that there are "various generalizations" of Morley's theorem, but by this it seems to mean extensions of Morley's theorem, i.e., further equilateral triangles that one can construct. This is not what I would, strictly speaking, call a "generalization."

So is David Rusin correct?

Are there no satisfactory generalizations of Morley's theorem?

Answer 1

Please forgive me if you are aware of this result (as it is linked from the Wikipedia page, albeit in another context), but there is a paper by Richard K. Guy called "The lighthouse theorem, Morley & Malfatti—a budget of paradoxes" in the American Mathematical Monthly. The eponymous theorem could be considered a generalization of Morley's theorem:

Lighthouse Theorem. Two sets of $n$ lines at equal angular distances, one set through each of the points $B$, $C$, intersect in $n^2$ points that are the vertices of $n$ regular $n$-gons.

Naturally, it is not clear how this would qualify as a generalization, but the connecting observation is the following:

The Morley Miracle. The nine edges of the equilateral triangles of the Lighthouse Theorem for $n=3$ are the Morley lines of a triangle.

Properly, the Lighthouse Theorem should be enlarged to include enough observations to make this connection. For example, the $n^2$ lines of the $n$ regular $n$-gons form $n$ families of $\binom{n}{2}$ parallel lines; if $n$ is odd, then the $n$-gons are homothetic. Moreover, there is an angle duplication result that establishes the presence of the trisectors.

From Guy's point of view, the particularly pleasant appearance of Morley's theorem is due to the fact that $\binom{n}{2} = n$ for $n=3$. For comparison, the case $n=2$ is even simpler and may be regarded as the statement that the altitudes of a triangle concur. (The $n$ $n$-gons are an orthocentric system.) The case $n=4$ gives some properties of Malfatti circles. For all of these interpretations, Guy wrestles with the "paradox" that you recover theorems about a triangle even though you don't start with any triangles.

Again, my apologies if you're aware of all of this. I imagine you may be, in which case I justify my answer as simply too long for a comment!

Answer 2

The generalization I was hoping for would start with: "Given any simplex in R^n, ..."; the case n=2 of this theorem would then be Morley's theorem.

I recall starting with a random tetrahedron in R^3 and trying a bunch of constructions looking for something regular to appear: I believe the variations I tried included trisecting and quadrisecting the dihedral angles, and drawing a few sets of regularly-spaced rays out of each vertex. Any three planes, any ray-plane pair, and occasional pairs of rays provide points of intersection, but I don't recall finding even any isosceles triangles among those points of intersection. Perhaps I miscalculated (or am mis-remembering)?

Answer 3

Morley originally found this theorem as a trivial case of much more complicated theorems. Anyone who says this theorem defies generalization is really just saying that they are unaware of its history.

See Oakley and Baker's 1978 paper for extensive discussion of Morley's theorem and over 100 references.

See also this question and answer, somewhat similar in spirit to the present question.

Answer 4

NATURAL GENERALIZATIONS OF MORLEY'S THEOREM

The question regarding possible generalizations of Morley’s theorem beyond equilateral triangles with vertices intersections of angle trisectors is at least premature if not vague. Only recently the exact 18 combinations of trisectors’ sorts have appeared in bibliography. Also it was found that 12 combinations of angle trisectors have intersections at vertices of an equilateral triangle respectively. So the angle trisectors of a triangle pass through the vertices of at least 54 equilaterals. Such studies provide a better understanding of Morley’s theorem before embarking for sweeping statements in abstract structures which are reduced to the theorem.

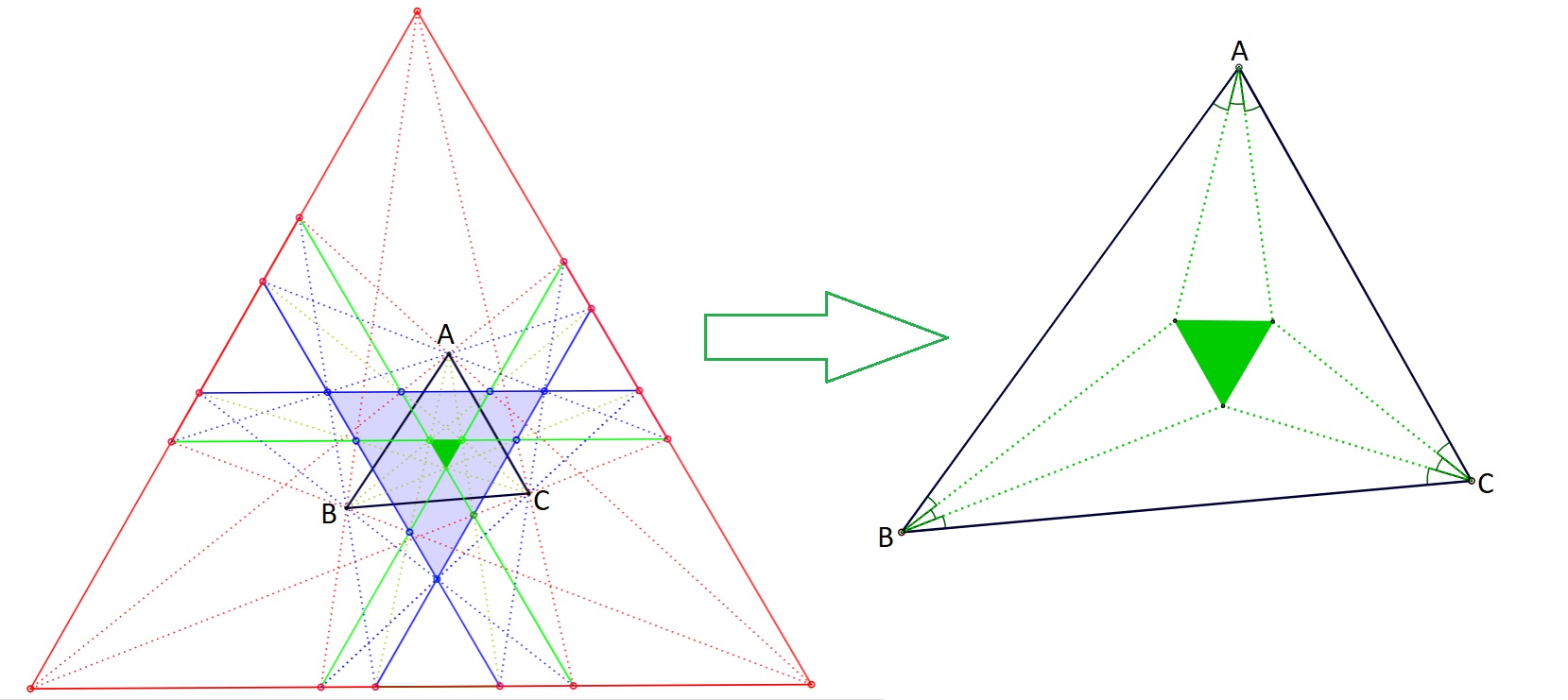
As noted, Morley's theorem holds just for triangles in Euclidean geometry but it does not in Elliptical or Hyperbolic geometries. This is also true for the Pythagorean Theorem. But the hypothesis of the theorem is subject to other natural generalizations which might imply the formation of an equilateral triangle. For instance, instead of angle trisectors we may consider isogonal lines, proximal to each angle’s sides respectively. These isogonal lines intersect at the vertices of an equilateral if and only if they are angle trisectors. This reveals that Morley’s theorem is a limit case of isogonal lines to each of the three angle sides respectively.

1. Morley's theorem origin. Morley concluded the theorem from a more general observation, among complicated cubic equations, while was studying meticulously cardioids tangent to the lines of the sides of a triangle.

In a triangle the intersections of trisectors proximal to a side are on (the meetings of) three triples of parallel lines making equilateral triangles.

The observation refers to the six trisectors of an angle, as outside it there are two more angles, its exterior and its explementary. Moreover, the proximal to a side trisector is the one which bisects the angle between a side and the other trisector.

From the observation the theorem follows readily.

As the interior trisectors, proximal to sides respectively, meet at the vertices of an equilateral, the same holds true for the intersections of exclusively exterior and explementary trisectors.

In a triangle the trisectors of same kind for all angles, proximal to sides respectively, meet at the vertices of a corresponding equilateral.

In fact, the observation ensures the formation of 18 equilaterals with vertices intersections of proper kinds of trisectors. Instead of describing the way that a particular equilateral is obtained, with an astonishing short sentence confirms the equilaterals existence.

While the theorem is specific and immediately understood, the description for the formation of all equilaterals is, at least initially, rather confusing. This may be the reason of not being widely known in contrast to the fame of the theorem. Subsequently the theorem has almost monopolized attention.

2. Morley's Extension Theorem. Morley’s theorem refers to the interior trisectors of a triangle. But 18 of its variants hold for proper combinations of trisectors. In the celebrated Alain Connes' paper Remark 1 notes ”... one obtains in this way the 18 nondegenerate equilateral triangles of variants of Morley’s theorem” but no details are provided.

In the following figure 6 equilaterals are depicted with vertices intersections of different combinations of trisectors kinds.

The next figure illustrates 3 triplets of equilaterals. Each one in a triplet shares a vertex with the equilateral formed by the intersections of same kind trisectors. Thus, their vertices are intersections of one kind trisectors for one angle and another kind trisectors for the other two angle.

In the paper A Trigonometrical Approach to Morley’s Observation we proved the following theorem using trigonometry. For a concise statement let the corresponding kind of interior, explementary and exterior trisectors be exterior, interior and explementary, respectively.

Morley's Extention Theorem In a triangle, the trisectors of same kind for all angles, a distinct kind for each, or a kind for one and its corresponding kind for the other two, proximal to sides respectively, meet at the vertices of an equilateral.

The Extension theorem may be considered as generalizing Morley's theorem statement. Informally and not precisely it states in a triangle the trisectors proximal to sides respectively, meet at the vertices of an equilateral without reference to trisectors' kinds.

Since each of these equilaterals has a vertex shared with another, they are intertwined in a selfsame agglomeration that its arrangement implies readily the alignments of trisectors’ intersections that Morley observed.

The above theorem covers 18 from 27 possible combinations of trisectors proximal to sides. It leaves out the cases that a kind for one angle and another kind for the other two angles, intersect at vertices of a non-equilateral triangle. The following figure indicates 3 from the 9 combinations of trisectors, proximal to sides, meeting at the vertices of a non-equilateral triangle on the arrangement of Morley triangles.

In our paper A holistic approach to Morley's general theorem a geometric proof of the Extension theorem is given. It demostrates 54 equilaterals which inlcude the previous 18 and 36 more equilaterals with vertices intersections of trisectors of just two angles. For example, the next figure illustrates equilaterals with vertices from which one is intersection of same kind trisectors and two are intesections of combinations of the remaining kinds, distal to AB.

Noticeably, the vertices of the 54 equilaterals are on 3 sets of 12 circles passing through two vertices of the triangle.

In a forthcoming paper, we will present uniform simple proofs for all valid Morley variants using basic properties of incenter and excenter of a triangle.

3. Morley's theorem cannot be generalized by considering (n)sectors instead of trisectors. The (n)sectors of an angle proximal to it sides are isononal to them and the following holds

In a triangle, isogonal lines to each angle sides, proximal to sides respectively, meet at the vertices of an equilateral if and only if they are trisectors.

The known proofs of the above are still rather complicated.

Incidentally, the intersections of isogonal lines to the sides of the angles of a triangle, proximal to their sides respectively, determine a Hofstadter triangle. So the interior Morley triangle is a Hofstadter triangle. But from the discussion above it is clear that the definition of Hofstadter triangle may be generalized to include outside isogonal lines too. So a generalized Hofstadter triangle is a Morley triangle if and only if the isogonal lines are angle trisectors.

Instead of angle (n)sectors, side (n)sectors or perpendicular to side (n)sectors may be considered. However it is easily seen that none of them leads to an equilateral triangle.

Thus Morley's theorem is an extreme rare case.

Answer 5

Please see here is our work about extension of Morley's theorem

On some extensions of Morley's trisector theorem

Answer 6

A recent "generalization":

Tran, Q. H. "Morley’s trisector Theorem for isosceles tetrahedron." Acta Mathematica Hungarica (2021): 1-8. DOI.

Abstract. We extend Morley’s trisector theorem in the plane to an isosceles tetrahedron in three-dimensional space. We will show that the Morley tetrahedron of an isosceles tetrahedron is also isosceles tetrahedron. Furthermore, by the formula for distance in barycentric coordinate, we introduce and prove a general theorem on an isosceles tetrahedron.

A "Morley tetrahedron" is determined by planes trisecting each dihedral angle.

Tran, Q. H. is likely MO user TranQuangHung.

Answer 7

We have tried to generalised Morley Trisector Theorem on 2n sided convex polygon and we make some condition and takes 1/k times of angle which intersect at n points and makes Regular polygon .for more details you can see our results which we have published on maths stock exchange here : https://math.stackexchange.com/questions/4339644/morleys-trisector-theorem-on-2n-convex-polygon In above link , we take {P1,P2,....,P2n} points which makes convex Polygon for n greater than or equal to 3 . And we choose alternate points and makes two Regular polygon with Coincident centroid and takes point which divide angle by 1/k times of angle alternatively for all K belongs to Real number and then pass the line through those points alternatively and they always form a regular n polygon . (See above link for more details!!) .

Answer 8

Possibly not what you are looking for but it does correspond to the question raised in the title. One can determine those auxiliary triangles $A_1B_1C_1$ of $ABC$ which are equilateral in terms of the swing angles of its vertices with respect to the sides. Recall that if $AB$ is a segment, then the swing angles of a third point $C$ are $\angle CAB$ and $\angle CBA$.

We use complex numbers to formulate the result. We can assume that our original triangle has vertices $0$, $1$ and $z $ (this $z$ is the shape of the triangle and depends only on it similarity class). We suppose that the auxiliary triangle has vertices $C_1$ with swing angles $\alpha_1$ and $\beta_2$ with respect to $AB$, $A_1$ with swing angles $\beta_1$ and $\gamma _2$ with respect to $BC$ and $B_1$ with swing angles $\gamma_1$ and $\alpha_2$ with respect to $CA$. Then $A_1B_1C_1$ is a positively oriented equilateral triangle if and only if $$(1-\lambda_1+\lambda_2)z+(1-\lambda_2)z \omega+\lambda_3\omega^2=0. $$ Here $\lambda_1=\frac{\tan \beta_2}{\tan \alpha_1+\tan \beta_2}+i\frac{\tan \alpha_1 \tan \beta_2}{\tan \alpha_1+\tan \beta_2}$ etc. and $\omega$ is the primitive cube root of $1$. (The classical theorem of Morley corresponds to both $\alpha$´s being a third of $\angle ABC$, etc.
Napoleon´s theorem is also included.).