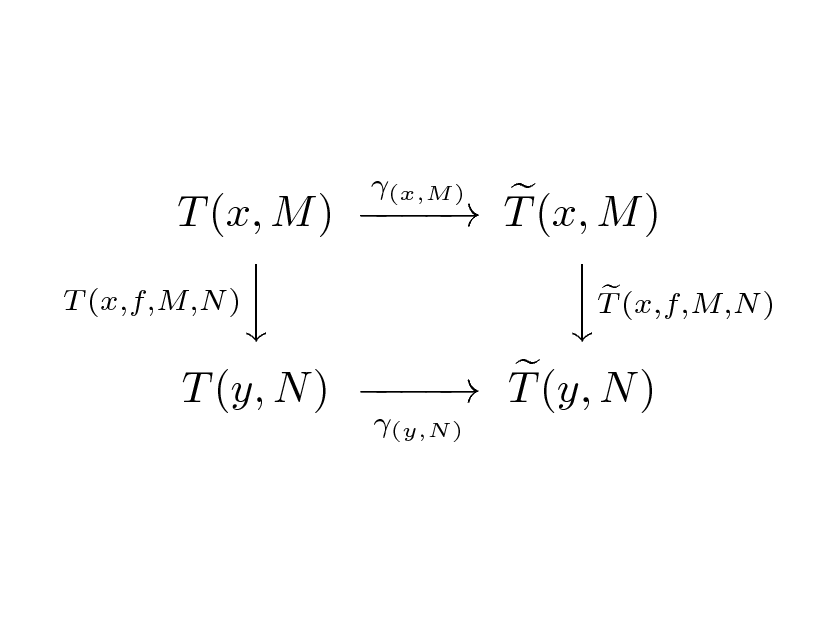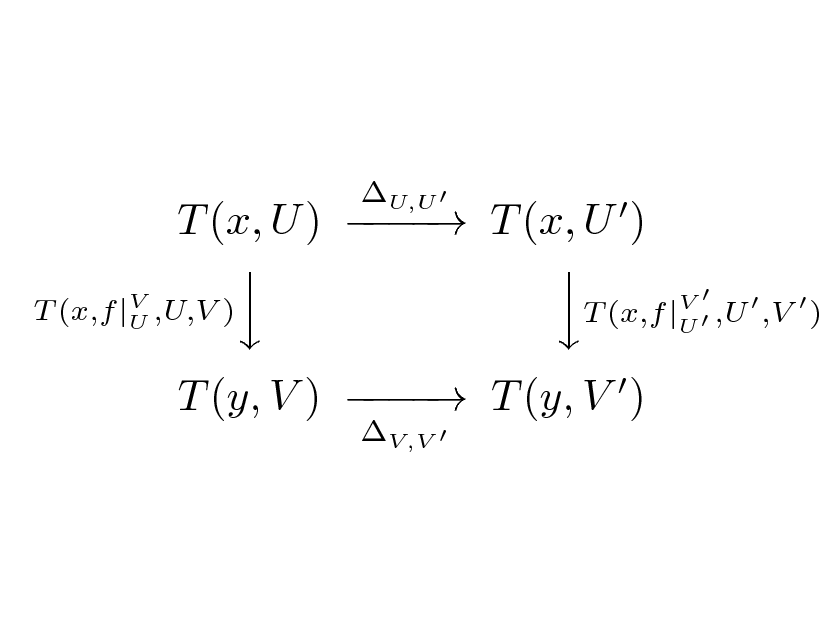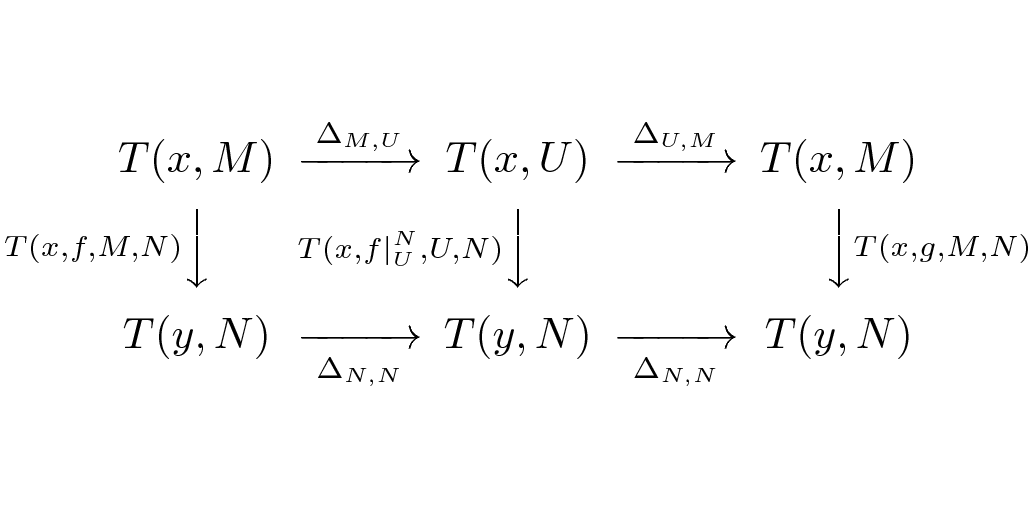Here is an explanation based on the category-theoretical formulation of tangent spaces.
In short: To give a definition of tangent spaces, one actually define a functor from the category of (pointed)manifolds to the category of vector spaces that is a natural extension of
the tangent space of Euclidean spaces satisfying the principle of localization(see below). It can be shown that the tangent space functor is uniquely determined by these two conditions, up to a natural isomorphism.
Hence to check that a concrete definition of tangent space is reasonable, one only need to show that this definition generalizes the concept of tangent spaces of Euclidean spaces, and satisfies the principle of localization.
If you have two such definitions, then they are equivalent in the sense that there exists a natural isomorphism between the functors representing the two definitions.
In detail: The category of pointed manifold $\mathcal M$ has objects in the form $(x,M),$ where $M$ is any smooth manifold and $x$ an element of $M.$
A morphism from $(x,M)$ to $(y,N)$ is an ordered quadruple $(x,f,M,N),$ in which $f$ is a functional relation on $M\times N$ with $\mathrm{dom}(f)$ a (not necessarily open)neighborhood of $x,$ $f$ differentiable at $x$ and $f(x)=y.$
More exactly, $f$ is a subset of $M\times N$ such that if $(x,y),(x,y')\in f$ then $y=y'.$
By definition, if $O$ is an open neighborhood of $x$ in $M$ such that $\mathrm{dom}(f)\subset O,$ and $O'$ is an open subset of $N$ with $\mathrm{Im}(f)\subset O',$ then $(x,f,O,O')$ is a morphism from $(x,O)$ to $(y,O').$
The composition of morphisms is given by
\begin{equation}
\boxed{(y,g,N,Q)\circ (x,f,M,N):=(x,g\circ f,M,Q)}
\end{equation}
where $g\circ f:=\{(x_1,x_3)|\ \exists \ x_2\in N,\ s.t. \ (x_1,x_2)\in f,\ (x_2,x_3)\in g \}$ is the composition of relations.
If we consider Euclidean spaces only, then we get a full subcategory $\mathcal M(\mathbb E)$ of $\mathcal M.$ Now we define the canonical tangent space of $(x,\mathbb R^n)$ to be simply $\mathbb R^n,$ and the canonical tangent map $T_{\rm canonical}(x,f,\mathbb R^n,\mathbb R^m):=D_xf,$
then we get a functor $T_{\rm canonical}:\mathcal M(\mathbb E)\to \mathsf{Vec}(\mathbb R).$
\begin{equation}
\boxed{T_{\rm canonical}\big[(x,\mathbb R^n)\stackrel{(x,f,\mathbb R^n,\mathbb R^m)}{\longrightarrow} (y,\mathbb R^m)\big]:=\mathbb R^n \stackrel{D_xf}{\longrightarrow}\mathbb R^m}
\end{equation}
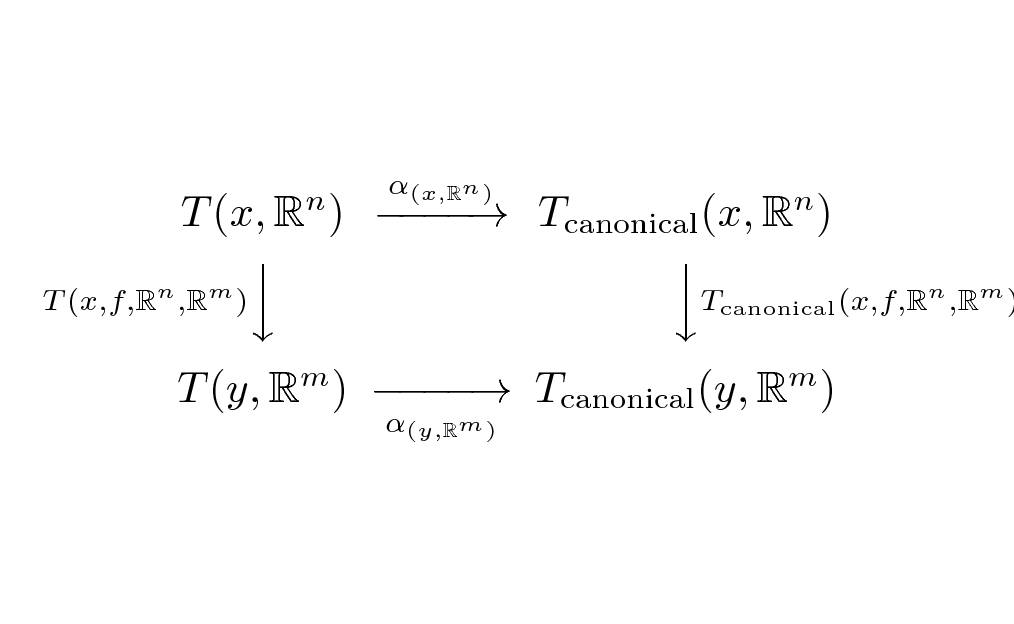
A tangent space functor defined on the full category $\mathcal M$ must generalize the concept of tangent spaces of Euclidean spaces, that is, it must be an extension of $T_{\rm canonical}.$ In fact this extension should be natural, that is to say,
the restriction of $T$ to $\mathcal M(\mathbb E)$ is nutural isomorphic to $T_{\rm canonical}.$ In other words,
for any object $(x,\mathbb R^n)$ there is an isomorphism $\alpha_{(x,\mathbb R^m)}:T(x,\mathbb R^n)\to T_{\rm canonical}(x,\mathbb R^n)=\mathbb R^n$ such that
if $(x,f,\mathbb R^n,\mathbb R^m):(x,\mathbb R^n)\to (y,\mathbb R^m)$ is a morphism, then the following diagram commutes:
We say that a functor $T:\mathcal M\to\mathsf{Vec}(\mathbb R)$ gives a definition of tangent spaces, if it satisfies the followng two conditions:
- $T$ is a natural extension of $T_{\rm canonical}.$
- (Principle of localization)If $(x,f,M,N),(x,g,M,N)$ are morphisms and $f,g$ coincide on a neighborhood of $x$ then $T(x,f,M,N)=T(x,g,M,N).$
Of course, if $T$ and $\widetilde T$ are two such functors, then their restriction to $\mathcal M(\mathbb E)$ are naturally isomorphic through the transformation $\beta:=\widetilde\alpha^{-1}\circ\alpha,$ since we have the commutative diagram
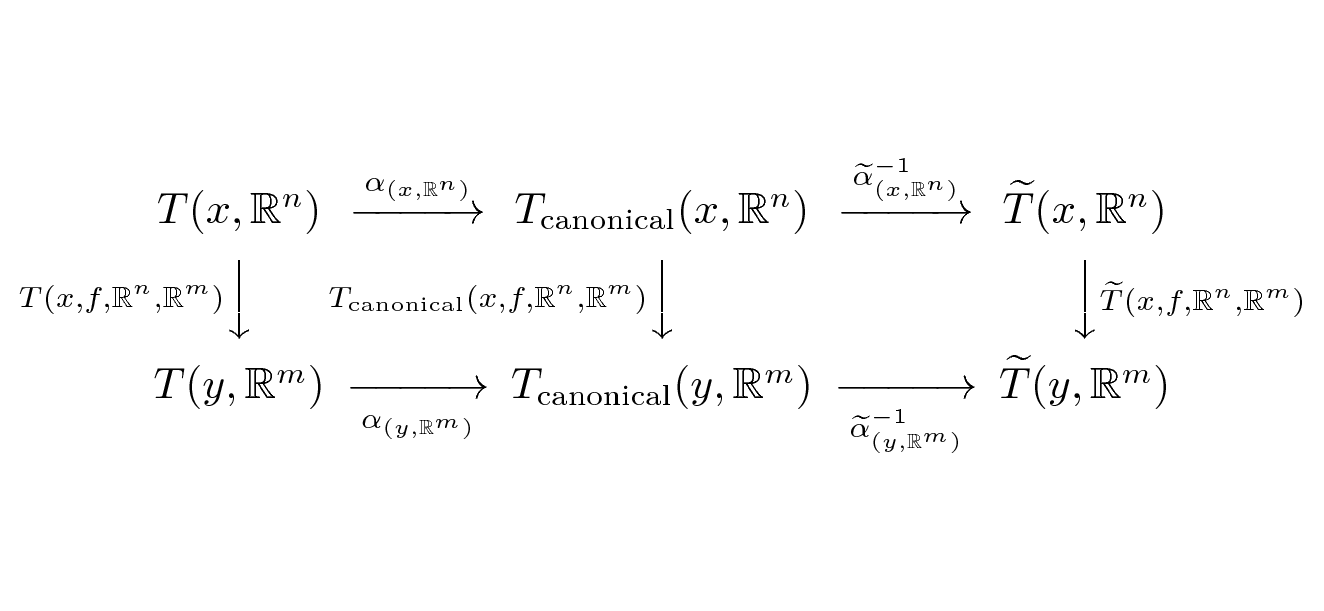
To show the uniqueness of tangent space functor, we need to find a natural isomorphism between $T$ and $\widetilde T.$
For any object $(x,M)$ of $\mathcal M,$ we define a vector space isomorphism
$\gamma_{(x,M)}:T(x,M)\to \widetilde T(x,M)$ by
\begin{equation}\boxed{\gamma_{(x,M)}:=\widetilde T(\varphi(x),\varphi^{-1},\mathbb R^n,M)\circ \beta_{(\varphi(x),\mathbb R^n)}\circ T(x,\varphi,M,\mathbb R^n)}\end{equation}
in which $(U,\varphi)$ is a coordinate chart for $x$ in $M.$ To show that $\gamma_{(x,M)}$ is well-defined, we have to verify that if
$(V,\psi)$ is another coordinate chart for $x$ in $M$ then
\begin{equation}\widetilde T(\varphi(x),\varphi^{-1},\mathbb R^n,M)\circ \beta_{(\varphi(x),\mathbb R^n)}\circ T(x,\varphi,M,\mathbb R^n)=\widetilde T(\psi(x),\psi^{-1},\mathbb R^n,M)\circ \beta_{(\psi(x),\mathbb R^n)}\circ T(x,\psi,M,\mathbb R^n)\end{equation}
In fact, principle of localization yields the commutative diagram
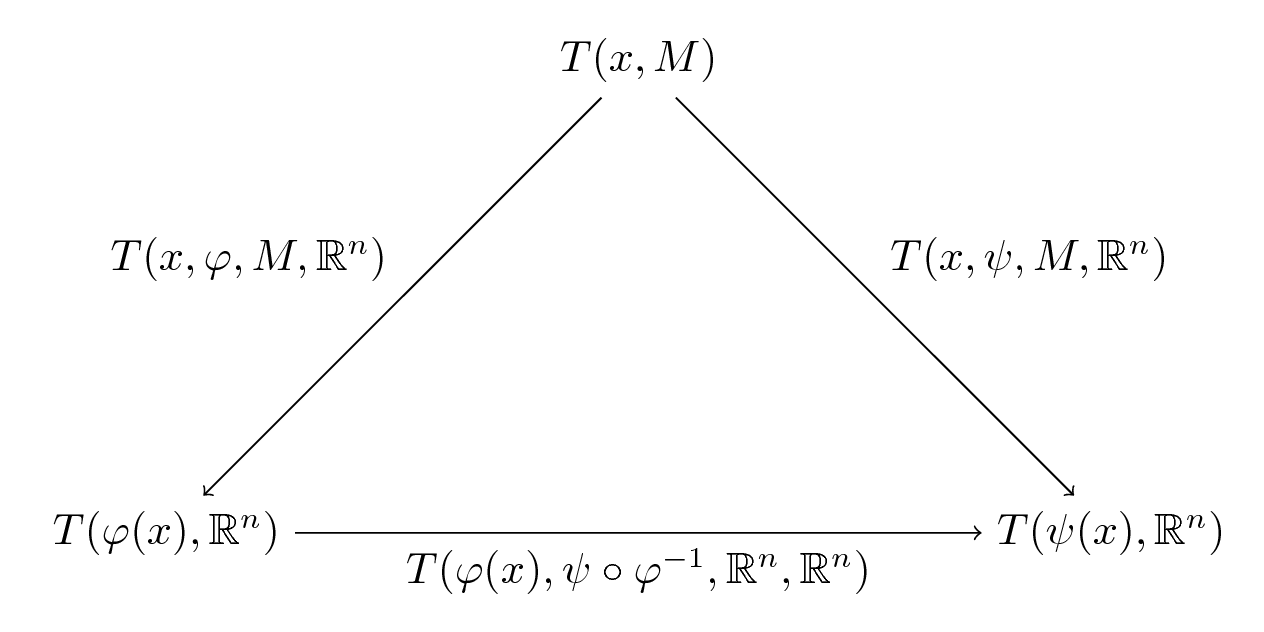
A similar digram commutes for the functor $\widetilde T.$ On the other hand, since $\psi\circ\varphi^{-1}$ is a functional relation on $\mathbb R^n\times\mathbb R^n,$ it follows that the following diagram commutes:
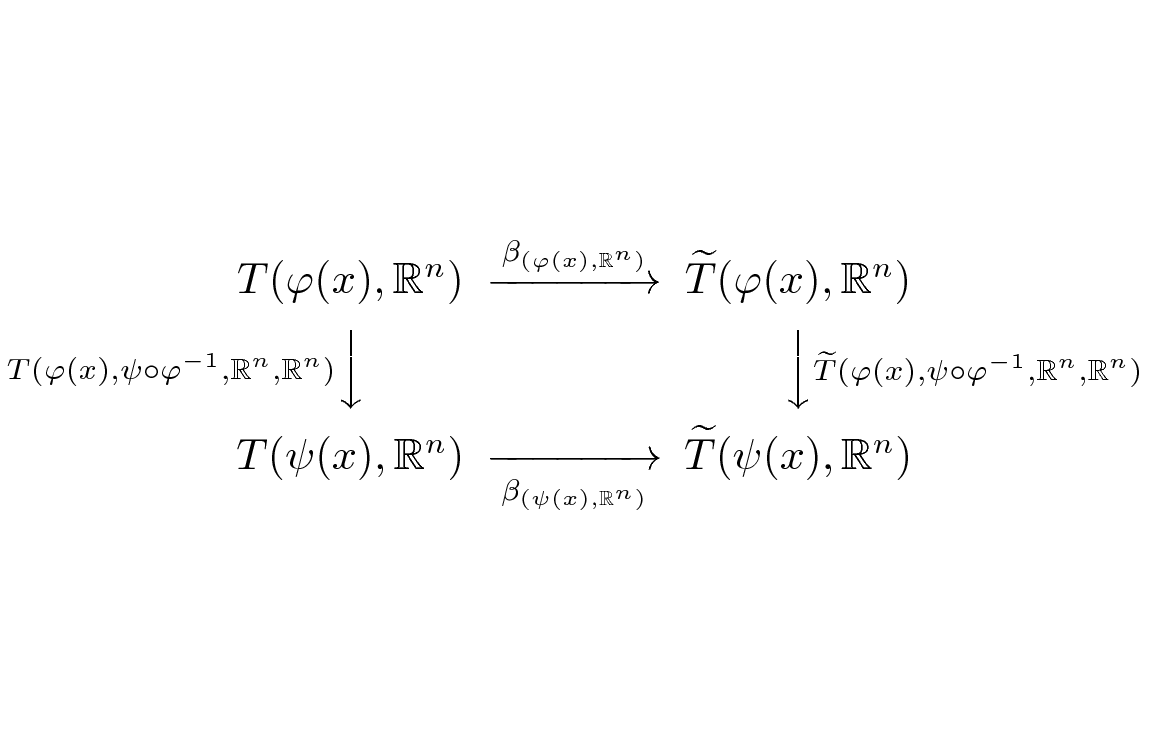
Thus we have a combined commutative diagram
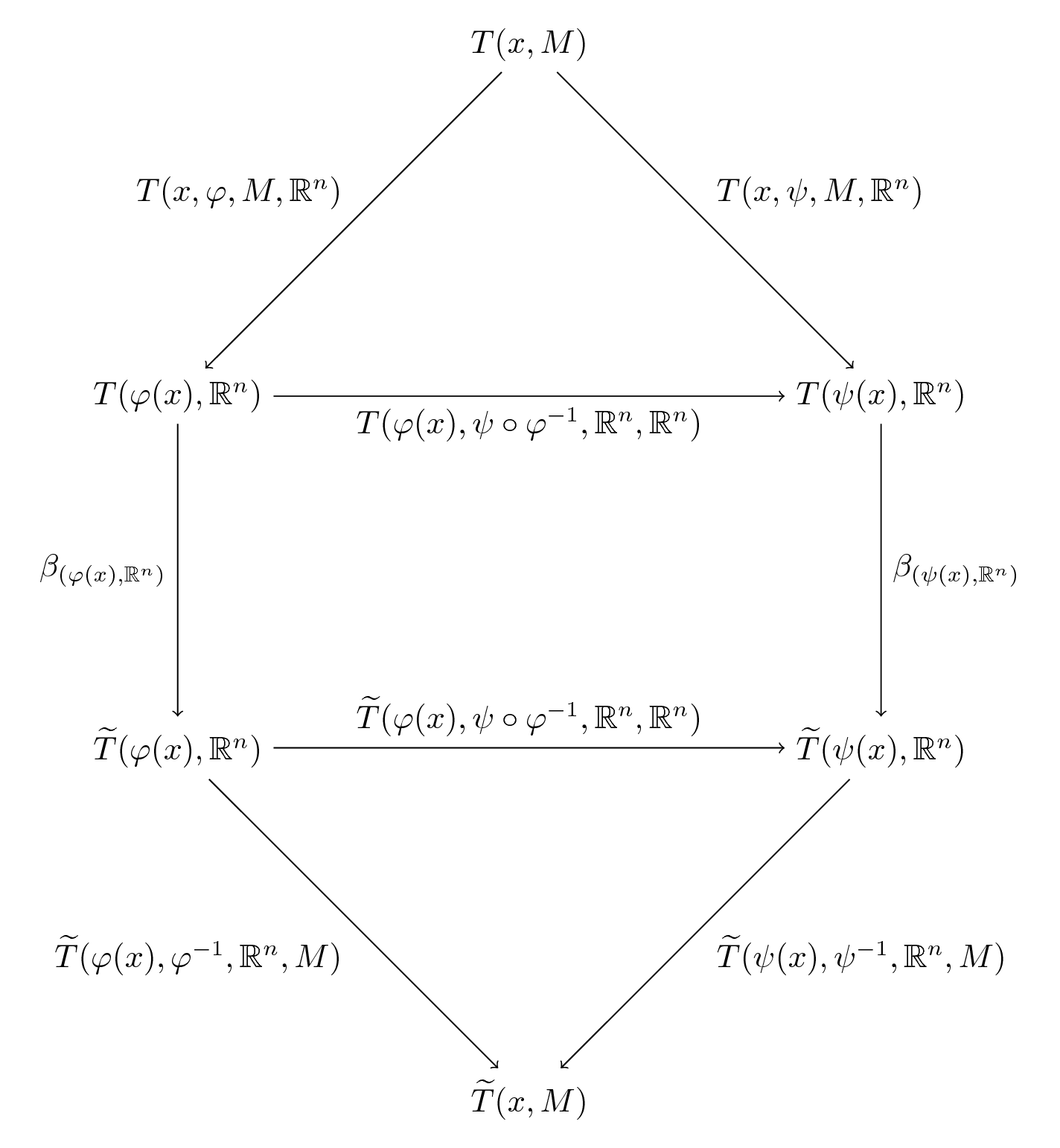
which is desired.
It remains to show that $\gamma$ is a natural isomorphism between $T$ and $\widetilde T.$
Let $(x,f,M,N)$ be a morphism from $(x,M)$ to $(y,N)$ and let $(U,\varphi)$ and $(V,\psi)$ be a coordinate chart for $x$ and $y,$ respectively, then $(\varphi(x),\psi\circ f\circ\varphi,\mathbb R^n,\mathbb R^n)$ is a morphism from $(\varphi(x),\mathbb R^n)$ to $(\psi(y),\mathbb R^n),$ hence
\begin{equation}\label{key}\widetilde T(\varphi(x),\psi\circ f\circ\varphi^{-1},\mathbb R^n,\mathbb R^n)\circ\beta_{\varphi(x),\mathbb R^n}=\beta_{\psi(y),\mathbb R^n}\circ T(\varphi(x),\psi\circ f\circ\varphi^{-1},\mathbb R^n,\mathbb R^n)\end{equation}
Plugging
\begin{equation}(\varphi(x),\psi\circ f\circ\varphi^{-1},\mathbb R^n,\mathbb R^n)=(y,\psi,N,\mathbb R^n)\circ (x,f,M,N)\circ(\varphi(x),\varphi^{-1},\mathbb R^n,M)\end{equation}
into the former equation gives
\begin{equation}\widetilde T(x,f,M,N)\circ \widetilde T(\varphi(x),\varphi^{-1},\mathbb R^n,M)\circ \beta_{(\varphi(x),\mathbb R^n)}\circ T(x,\varphi,M,\mathbb R^n)=\widetilde T(\psi(y),\psi^{-1},\mathbb R^n,N)\circ\beta_{(\psi(y),\mathbb R^n)}\circ T(y,\psi,N,\mathbb R^n)\circ T(x,f,M,N)\end{equation}
hence we have the commutative diagram
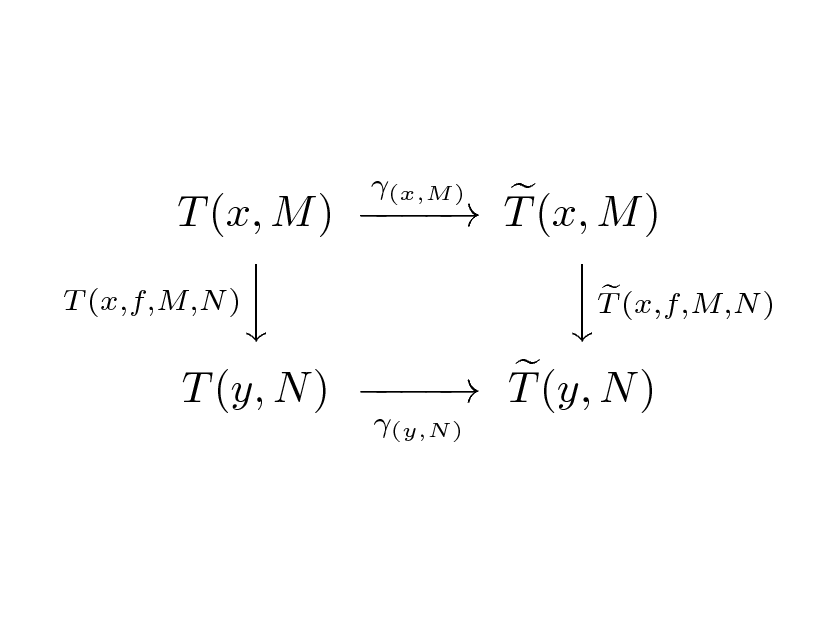as expected.
The principle of localization is equivalent to the following statement: for any two open subsets $U,U'$ of any manifold $M,$ if $x\in U\cap U'$ then there is an isomorphism $\Delta^{(x,M)}_{U,U'}$(abbreviated as $\Delta_{U,U'}$) satisfying the cocycle condition $\Delta_{U',U''}\circ \Delta_{U,U'}=\Delta_{U,U''},\ \Delta_{U,U}=\mathrm{id}$ and
if $(x,f,M,N)$ is a morphism from $(x,M)$ to $(y,N),$ then the following diagram commutes:
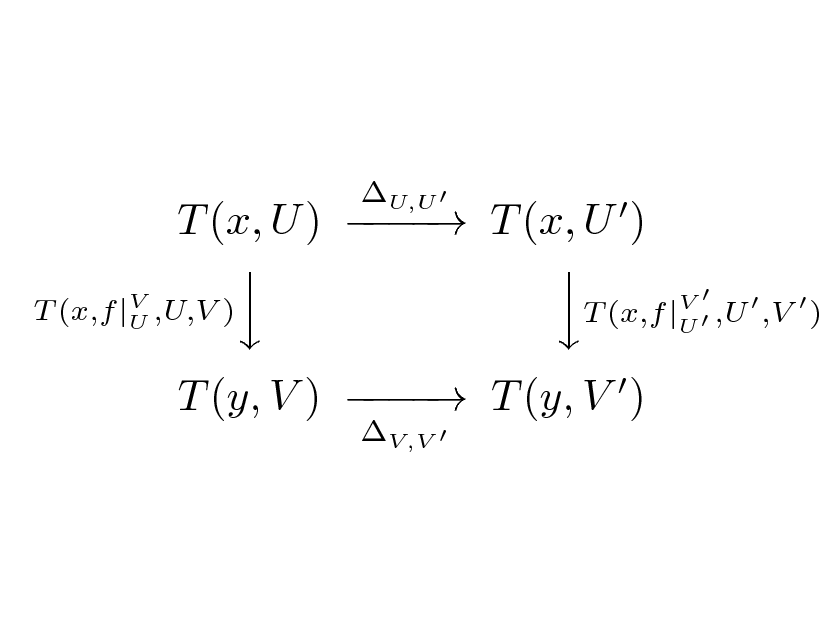Here $f|_{U}^V:=f\cap (U\times V)$ and it's easy to see that $(x,f|_U^V,U,V)$ is a morphism from $(x,U)$ to $(y,V).$
In fact, assume that the principle of localization hold, then defining
\begin{equation}\Delta_{U,U'}^{(x,M)}:=T(x,\mathrm{id}_{U\cap U'},U,U')\end{equation}
will make the cocycle diagram commute.
Conversely,assume that the cocycle diagram commute, and assume $f$ coincide $g$ on a neighborhood $U$ of $x,$ then we have the commutative diagram
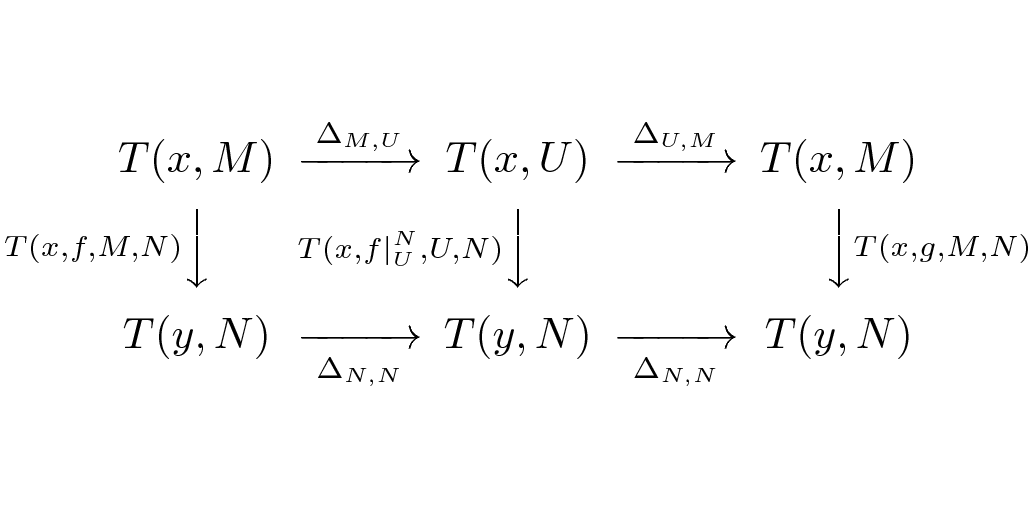Since $\Delta_{U,M}\circ\Delta_{M,U}=\mathrm{id},\ \Delta_{N,N}=\mathrm{id},$ it follows that $T(x,f,M,N)=T(x,g,M,N),$ which establishes the equivalence of the two statements.
