Suppose you have a supply of infinite-length, opaque, unit-radius cylinders, and you would like to block all visibility from a point $p \in \mathbb{R}^3$ to infinity with as few cylinders as possible. (The cylinders are infinite length in both directions.) The cylinders may touch but not interpenetrate, and they should be disjoint from $p$, leaving a small ball around $p$ empty. (Another variation would insist that cylinders be pairwise disjoint, i.e., not touching one another.)
A collection of parallel cylinders arranged to form a "fence" around $p$ do not suffice, leaving two line-of-sight $\pm$ rays to infinity. Perhaps a grid of cylinders in the pattern illustrated left below suffice, but at least if there are not many cylinders, there is a view from an interior point to infinity (right below).
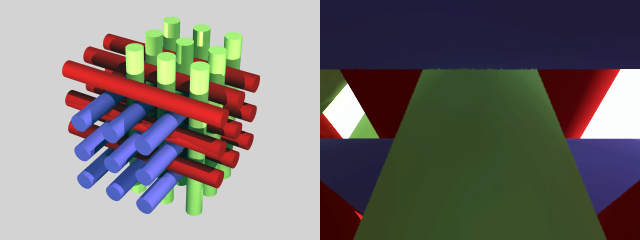
I feel like I am missing a simple construction that would obviously block all rays from $p$. Perhaps crossing the cylinders like the poles of a tipi (teepee) could help, but it seems this would at best lead to inefficient blockage. Suggestions welcome—Thanks!
Addendum1. Perhaps if the weaving above is rendered irregular by displacing the cylinders slightly by different amounts, so that cracks do not align, then a sufficient portion of the weaving will block all visibility.
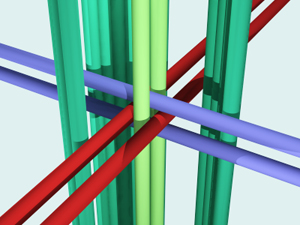
Here (left below) is the start of Gerhard's first suggested construction (a portion of the weaving above), which I don't see how to complete. But perhaps
seeing this depiction will aid intuition.

Addendum2. To the right above I added (three-quarters of) a forest along the lines (but not exactly as) Yaakov suggested.
