Do toy models of quantum mechanics help us better understand "regular" quantum mechanics? For example, if we look at quantum mechanics over a finite field $F$ (e.g. $\mathbb{Z}_2$), can this lead to new insights for "regular" quantum mechanics? Or do these toy models just help clarify our understanding of "regular" quantum mechanics without actually providing any new insight? In other words, what is the utility of having toy models of quantum mechanics?
-
2$\begingroup$ Don't you really mean $\mathbb F_2$ (the prime characteristic 2 field), instead of $\mathbb Z_2$ (the completion of $\mathbb Z$ along 2 -- which is of characteristic 0)? $\endgroup$– Julien PuydtCommented Mar 20, 2011 at 8:42
-
8$\begingroup$ @Snark: topologists and physicists usually use the notation " $\mathbb{Z}_n$ " for the ring $\mathbb{Z}/n$. $\endgroup$– QfwfqCommented Mar 20, 2011 at 13:55
-
5$\begingroup$ Toy models are certainly valuable, but I disagree that "quantum mechanics over a finite field" is a "toy" with which we can "better understand 'regular' quantum mechanics". Indeed, many of the standard axioms in QM have a lot to do with structure on the complex numbers: positivity, for example, or unitarity, or self-adjointness. It's not at all clear what the corresponding constructions should be in positive characteristic. Integrals are also less-well behaved, or anyway more nuanced. If you want to write down a theory of differential equations, among other things you have to ask (continued) $\endgroup$– Theo Johnson-FreydCommented Mar 20, 2011 at 14:02
-
2$\begingroup$ (continuation) yourself whether you want the things corresponding to "polynomials" to allow divided powers or not. You may want to identify one-parameter families of operators with their derivatives at the identity --- you may want Lie theory --- but not every Lie algebra integrates to an algebraic group, and in positive characteristic you don't have as good access to notions like "compact" or "negative definite" that can assure that they do. Which is all to say: "physics in characteristic p" is less a "toy" and more a hard area of research. (Even p-adic --- char=0 --- physics is worth doing!) $\endgroup$– Theo Johnson-FreydCommented Mar 20, 2011 at 14:06
-
3$\begingroup$ Historical note: in his book The Theory of Groups and Quantum Mechanics Hermann Weyl actually did consider these toy models of quantum mechanics. The case of $\mathbf Z_2$ models electron spin. He speculated that other finite groups may arise in the context of nuclear physics. It was Mackey who developed the theory of Heisenberg groups in the context of locally compact abelian groups (A Theorem of Stone and von Neumann, Duke Math. J., 1949). $\endgroup$– Amritanshu PrasadCommented Mar 21, 2011 at 0:46
10 Answers
Here is a really great blog post by frequent MO contributor Qiaochu Yuan, entitled "The Schrodinger Equation on Finite Graphs". This is, in my opinion, an excellent toy model which should really be presented in intro QM courses. The punchline is that representations of the automorphism of a graph should correspond to "particles" moving around on the graph. In a sequel post, Qiaochu mentions that this toy model shows up in quantum computing research (see this Wikipedia article and this recent preprint).
-
2$\begingroup$ I don't see how this is relevant. The linked blog post still has wavefunctions defined over the complex numbers. It seems that PEV is asking about wavefunctions taking values over finite fields. $\endgroup$ Commented Mar 20, 2011 at 16:26
-
7$\begingroup$ The OP gives finite fields as an example of a toy model, but I think the question was intended to include other toy models as well. T $\endgroup$ Commented Mar 20, 2011 at 17:09
-
1$\begingroup$ @Qiaochu, yes you're right; I read the question too hastily. Please see my comment at the top, though. Your blog post is a great example of regular quantum mechanics, which I think isn't the spirit of the question. $\endgroup$ Commented Mar 20, 2011 at 17:27
-
1$\begingroup$ @Steve: For a toy model to be good, I would say it should exhibit many of the properties of the real thing while behaving better in some ways. I think any model over finite fields is guaranteed to fail both of these tests; the model on finite graphs has the benefit that all groups are finite and all representations are finite-dimensional. $\endgroup$ Commented Mar 20, 2011 at 18:29
-
1$\begingroup$ @Daniel, I agree that finite dimensional Hilbert spaces simplify the theory. Qiaochu's post and your answer are great in that they provide basic examples of quantum mechanics. If this is what PEV is asking, then I also offer systems like the harmonic oscillator, a free particle in 1d, and even two spin-1/2 particles as other "toy models". All of these fit squarely within standard QM theory, and anyone interested in learning standard QM should study them. However, I interpret the question as asking for models that are distinct from standard QM. Let's call these toy theories... (cont.) $\endgroup$ Commented Mar 20, 2011 at 19:46
It's important to remember that quantum probability, which is part of quantum mechanics, is a generalization of classical probability that is really very similar in many ways, even though many of the answers are markedly different. Much of classical probability can be viewed as the theory of commutative von Neumann algebras, while quantum probability is the theory of non-commutative von Neumann algebras.
People don't have all that much temptation to make toy models of classical probability that, for instance, replace the real numbers by a finite field. In any case I don't know of any toy models that are all that useful. The most that I would say for either theory is that classical probability is a good toy model for quantum probability. It is an important toy model in information theory, for example --- both models already have channels, entropy, channel capacity, etc. And stochastic differential equations are an important toy model (or even an accurate method of calculation) for the Schrodinger equation, that the physicists sometimes call Wick rotation.
The other side of quantum mechanics is the mechanics, i.e., the forces, geometry, and dynamics on which one imposes quantum probability. For this part of the theory, I have heard that $p$-adic geometry shows some promise as a useful toy model or at least an interesting alternative model. I.e., the quantum probability stays the same but spacetime is replaced by a $p$-adic variety. I don't have great references for this at my fingertips though. Okay, here is an arXiv paper on $p$-adic quantum field theory, but I suspect that it's not the best example. (And, as in the link to Qiaochu's blog post, finite graphs and discretization are another type of toy model of space.)
Do toy models of quantum mechanics help us better understand "regular" quantum mechanics?
My answer is a resounding "yes!" First of all, let me say that I agree with Steve that it is better to talk about toy theories than toy models. In fact, I favor the terminology “foil theory” for this sort of thing. Essentially, if we want an answer to the question “why quantum theory?” we need to study the alternatives, the ways the world might have been – the foils to quantum theory. It is only against a landscape of possible theories that one can identify what is special about quantum theory.
One can certainly devise foil theories by a modification of certain features of the formalism of quantum theory. However a more fruitful approach, in my view, is to define novel foil theories by deriving their formalism from a set of physical principles within an operational framework. Recently, there have been several approaches of this sort. For instance, there has been a lot of work in the last few years on foil theories that allow violations of Bell inequalities that are stronger than those allowed in quantum theory, but which still do not allow superluminal signalling.
There have been several workshops on this topic. The first was in Cambridge in 2007. Matt Leifer provides a synopsis here: http://www.fqxi.org/community/forum/topic/86. The next two happened at the ETH in Zurich in 2008 and 2010: https://web.archive.org/web/20130801040747/http://www.qit.ethz.ch/workshops/IPLN2008. Finally, there will be a conference this year at Perimeter Institute: https://web.archive.org/web/20110524065750/http://www.perimeterinstitute.ca/Events/Conceptual_Foundations_and_Foils_for_Quantum_Information_Processing/Abstracts/. Looking up the participants of these workshops on the arxiv will yield lots of relevant papers.
My own work in this area starts with a classical statistical theory, and posits a principle that restricts how much information an agent can have about the classical state. The article “In defense of the epistemic view of quantum states: a toy theory”, http://arxiv.org/abs/quant-ph/0401052 describes one such foil theory, and the following talk of mine from 2008 summarizes the broader research program at a nontechnical level: http://pirsa.org/08020051/. The foil theories one obtains in this way (epistemically-restricted classical statistical theories) reproduce a wide variety of quantum phenomena, such as the noncommutativity of measurements, interference, many features of entanglement, no cloning, teleportation, mutually unbiased bases, and many others. What they suggest about the interpretation of quantum theory is that quantum states are states of incomplete knowledge rather than states of reality.
Regarding finite field versions of quantum mechanics, the following paper is a good place to start:
"Modal quantum theory", by Schumacher & Westmoreland, arXiv:1010.2929
In this paper, the authors present a discrete model of quantum theory that is similar to standard quantum mechanics, but it is based on finite field-valued amplitudes instead of complex amplitudes. The theory is surprisingly rich: it allows for entangled states and contains versions of the no-cloning theorem and Bell's theorem. It also has a distinctly different flavor when it comes to probabilities, namely (as the title suggets) it deals only with modality, the possibility or necessity of an outcome, rather than probability measures.
There is at least one other program for studying toy theories which comes to mind. These go by the name of "general probabilistic theories" or "convex operational theories". Here the idea is to replace quantum mechanics with a general convex space which becomes the space of quantum states. Then you decorate this space with things like tensor products to define composite systems and so on. It lets you ask questions about why quantum mechanics is special, since only some of the things we think of as being quintessentially "quantum" can be done in these other theories. In this regard, they do indeed shed important light on the nature of quantum mechanics.
The names I most associate to this approach are Barnum, Barrett, Leifer and Wilce, though I may have forgotten some others. Searching the quant-ph arxiv will turn up some papers for you. One which I've read personally, and it should get you started, is
"Information processing in convex operational theories", Barnum & Wilce, arXiv:0908.2352
Though it is not a finite field the non-archimedian versions of quantum mechanics have indeed been used quite a bit in the recent years. Here I would like to draw attention to a different approach, not to the $p$-adic numbers which have been mentioned already in several answers/comments.
One big flaw of the $p$-adics is that they are not ordered: there is no notion of positivity in the field of $p$-adics. Positivity on the other hand is crucial at many places in quantum physics (stability, probabilistic interpretations, ...). So one may wonder whether there are field extensions of $\mathbb{Q}$ which are ordered and still relevant in QM. Of course, if we stick to archimedian orders then the only field (which is also complete) are the reals, so this we all know. However, if we allow for non-archimedian orders then there are plenty more such fields. The most simple ones are perhaps the formal Laurent series $\mathbb{R}((\hbar))$ in a formal parameter $\hbar$. This is the quotient field of the (ordered) ring of formal power series $\mathbb{R}[[\hbar]]$.
As my notion already suggest this is the field underlying quantization theory itself if one (as a first step into the right direction) treats quantization as a (at this stage still) infinitesimal deformation of classical physics with deformation parameter $\hbar$, commonly known as deformation quantization. The non-achimedian order simply means that $\hbar$ is positive but smaller than e.g. $\frac{1}{n}$ for all $n \in \mathbb{N}$. So physically speaking the quantum effects are still "very small" at this stage of the theory.
It is now the positivity which allows to mimick many constructions from operator algebra also in this framework like e.g. a GNS construction, notions of pre Hilbert spaces, etc. Of course, for a honest quantum theory, $\hbar$ is not infinitesimally small, but has to be replaced by a real positive number. From this point of view the formal deformation quantization approach is only a first step, but still a very important one when it comes to the construction of quantum mechanical models for complicated classical theories.
OK, I hope this is not too off-topic, but I was inspired by the nice answers on the $p$-adics ;)
One problem with non-"regular" fields in quantum mechanics is that the Spectral Theorem may fail. For example, if I recall correctly there is a $3\times 3$ symmetric matrix with entries in $\mathbb Z_2$ that is not diagonalizable by any matrix over $\mathbb Z_2$.
-
2$\begingroup$ Also a 2 x 2. The point is that Z_2 is not algebraically complete: the polynomial z^2 + z + 1 has no roots in it. So e.g. the matrix [ 1 1; 1 0] has no eigenvalues in Z_2. $\endgroup$ Commented Mar 20, 2011 at 7:38
-
8$\begingroup$ The fact that $\mathbb{Z}_2$ is not algebraically closed is irrelevant - a symmetric matrix over the algebraic closure of $\mathbb{Z}_2$ need not be diagonalizable. For example if $$ A= \left(\begin{array}{ccc}0&1&0\\ 1&0&1 \\0&1&0\\ \end{array}\right) $$ then the minimal polynomial of $A$ in characteristic two is $x^3$, and so $A$ cannot be diagonalizable. $\endgroup$ Commented Mar 20, 2011 at 16:17
-
3$\begingroup$ I'm not sure this is a problem. In standard QM the spectral theorem means that observables just happen to coincide with selfadjoint operators. This is of course mathematically very handy, but not essential to modelling observables mathematically in the first place. I'd rather conclude that "nonstandard toy models of QM" might need to model observables by adapting a different formulation, such as POVMs, instead of selfadjoint operators. $\endgroup$ Commented Mar 20, 2011 at 20:18
-
$\begingroup$ The easiest example of a non-diagonalizable matrix is perhaps the non-zero nilpotent matrix $\left(\begin{array}{cc}1&1\\1&1\end{array}\right)$ over the field of two elements. $\endgroup$ Commented Mar 21, 2011 at 9:33
The above answers and comments do not mention monographs giving detailed expositions of non-Archimedean quantum mechanics and related subjects in analysis. See
V.S. Vladimirov, I.V. Volovich, and E.I. Zelenov, p-Adic Analysis and Mathematical Physics, World Scientific, 1994,
for quantum mechanics on $\mathbb Q_p$ with complex-valued wave functions, and
A. Khrennikov, Non-Archimedean Analysis: Quantum Paradoxes, Dynamical Systems and Biological Models, Kluwer, 1997,
for a completely non-Archimedean theory. Analysis over fields of positive characteristic including such things as representations of canonical commutation relations of quantum mechanics is given in
A.N. Kochubei, Analysis in Positive Characteristic, Cambridge University Press, 2009.
All the above are in fact not toy models, but the exploration of mathematical subjects emerging as one tries to develop non-Archimedean quantum theory. They are undoubtely interesting though it remains to be seen whether they correspond to any realistic physics.
This perhaps elaborates on Greg's answer regarding quantum field theory on the p-adics. One approach to studying "regular" quantum field theory over $\mathbb{R}^d$ is to consider probabilty measures on the space of fields $\phi:\mathbb{R}^d\rightarrow\mathbb{R}$. These are obtained as limits of Radon-Nikodym pertubations of better understood Gaussian measures. The mathematical analysis involved, typically based on rigorous renormalization group techniques, is very difficult. One can define analogous models over the p-adics where the fields $\phi$ become random generalized functions $\mathbb{Q}_p^d\rightarrow\mathbb{R}$. There the analysis is much easier, and therefore the p-adic case is a good "toy model" for the real case. The idea is to develop one's tools and methods on the p-adics first, and then add the necessary refinements to handle the real case as a second step. Most of the core difficulties of the renormalization group analysis are already present in the p-adic toy model. However some features of the "regular" real case such as the flow of the wave function renormalization coupling are specific to the real case and therefore cannot be studied with this toy model. This being said I also believe such models over the p-adics are worthy of study per se and not just as toy models for "regular" QFT over the reals, in which I agree with Theo's comment above.
Edit: I gave a few more details about this particular toy model in this MO answer. Also note that there has been quite a bit of action going on recently in this area since $p$-adics provide a simplified setting in which one can study the so-called AdS/CFT correspondence. See, e.g., Gubser's talk at the Strings 2016 conference.
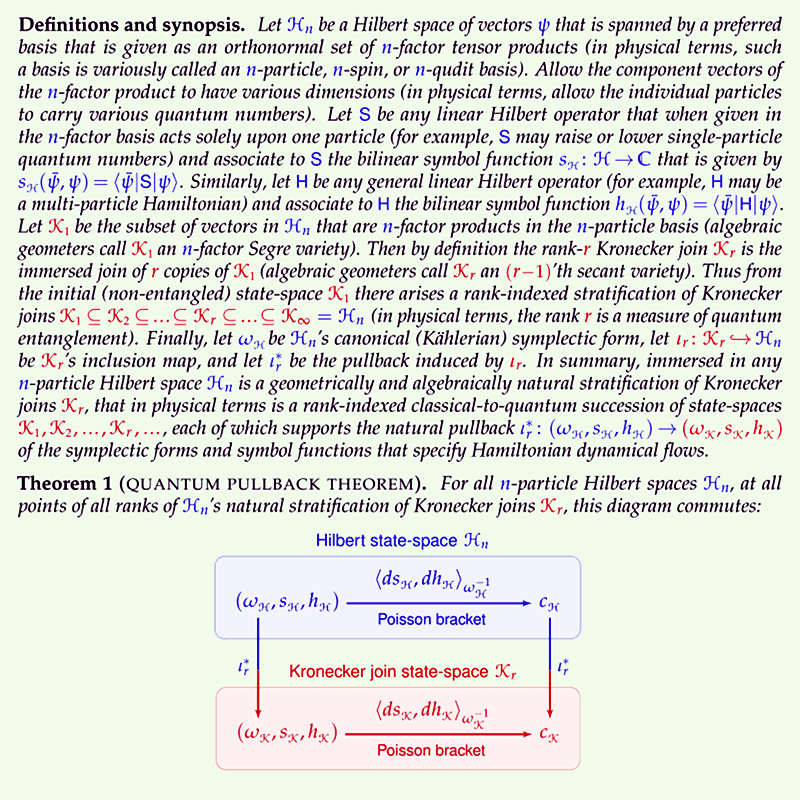
The preceding "toy models" focus mainly on algebraic properties of quantum state-spaces. Students who prefer a more geometric and/or PDE perspective on quantum dynamics may find it instructive to consider classical dynamical systems, which (historically) have always been a rich source of quantum dynamical "toy models".
Classical: The state-spaces of classical dynamical systems typically are endowed with both a symplectic structure and a metric structure. Canonical examples ("toy problems") include the Bloch equations and the Landau-Lifshitz-Gilbert equations: both have $S^2$ as their state-space; the symplectic and metric structures are natural; and in both cases dynamical trajectories unravel according to canonical fluctuation-dissipation relations.
Quantum: $C^n$ is the state-space; the symplectic and metric structures are natural (that is, Kählerian); and dynamical trajectories unravel according to canonical fluctuation-dissipation (that is, Lindbladian) relations.
In this geometric dynamical framework, what are often regarded as "spooky mysteries" of quantum mechanics are seen to be similarly present in classical theories; the main change is that global spectral theorems that apply in $C^n$ appear classically as local theorems involving dynamical flow.
These ideas are scattered throughout in the literature of math and physics, but there is at present no single textbook that develops this unified classical/quantum dynamical point of view. This for me defines " a book that you would like to write" (in Gil Kalai's phrase); further references to the literature can be found in that post.
In the meantime, a good "toy" exercise is to write down the symplectic and metric structures, and the Hamiltonian and Lindbladian potentials, that describe the Bloch equations in geometrically natural terms. In particular, an illuminating exercise is to derive via classical geometric methods the results that Charles Slichter's Principles of magnetic resonance derives quantum mechanically in Section 3.2, equation 3.7.
Note added: At the 52nd ENC Conference at Asilomar conference, we reduced the above ideas to practice, by redrafting Chapter III of Slichter's Principles of magnetic resonance in the language of geometric dynamics (PDF here, Wayback Machine). Here is the main theorem:
In essence, it turns out to be surprisingly easy to construct nonlinear quantum state-spaces that respect both thermodynamic "goodness" (that is, Kählerian structure) and quantum "goodness" (that is, Lindbladian structure).
Ought we to regard Kronecker state-spaces as legitimate quantum foil theories? Or are they merely useful computational idioms? Don't ask us ... as quantum simulations become more-and-more realistic, the division between quantum foil theories and practical computational tools is becoming less distinct.
The theory of nonlocal boxes is quite illuminating to the beginner (or expert) trying to get a handle on entanglement. (Google "nonlocal box" or "box world".)