More precisely, given an integer $n$, does there exist a non-periodic tiling, where there are infinitely many patches within the tiling, of indefinitely large area, with rotational symmetry of order $n$?
Question. For what values of $n$ can we find such tilings?
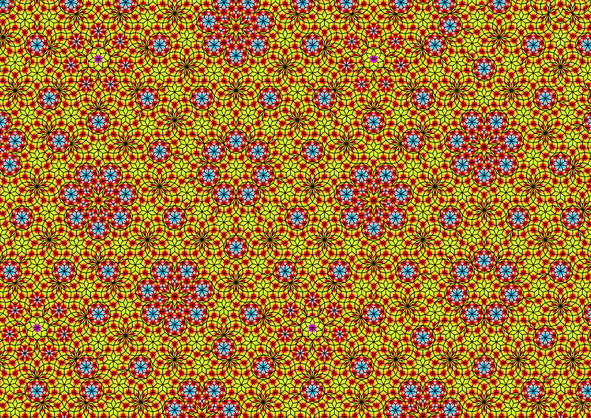
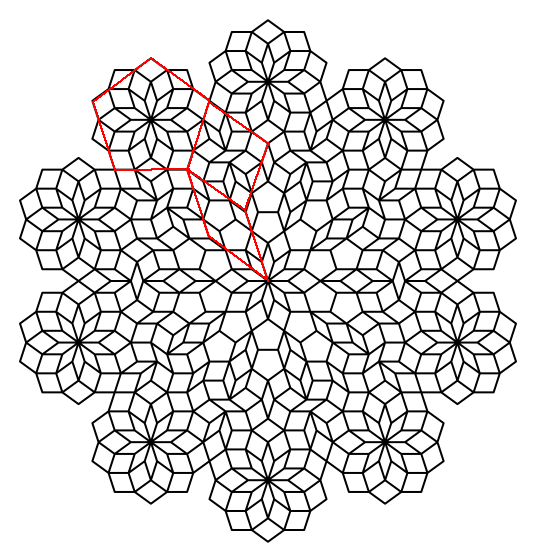
I have started to work on this problem. From the literature, I found examples for $n=5$ (Penrose tiling) and $n=7$ (Madison's $7$-fold). Then, from my own investigation, I found examples for $8$, $10$, $12$, and $16$.
Here are pictures of these tilings:
$n=5$
$n=7$
$n=8$
$n=10$
$n=12$
$n=16$