If I have a rectangle ABCD such that A and B touch two points of the outer circle and CD's touches one point of the inner circle, how could the maximum dimensions of the rectangle be calculated?
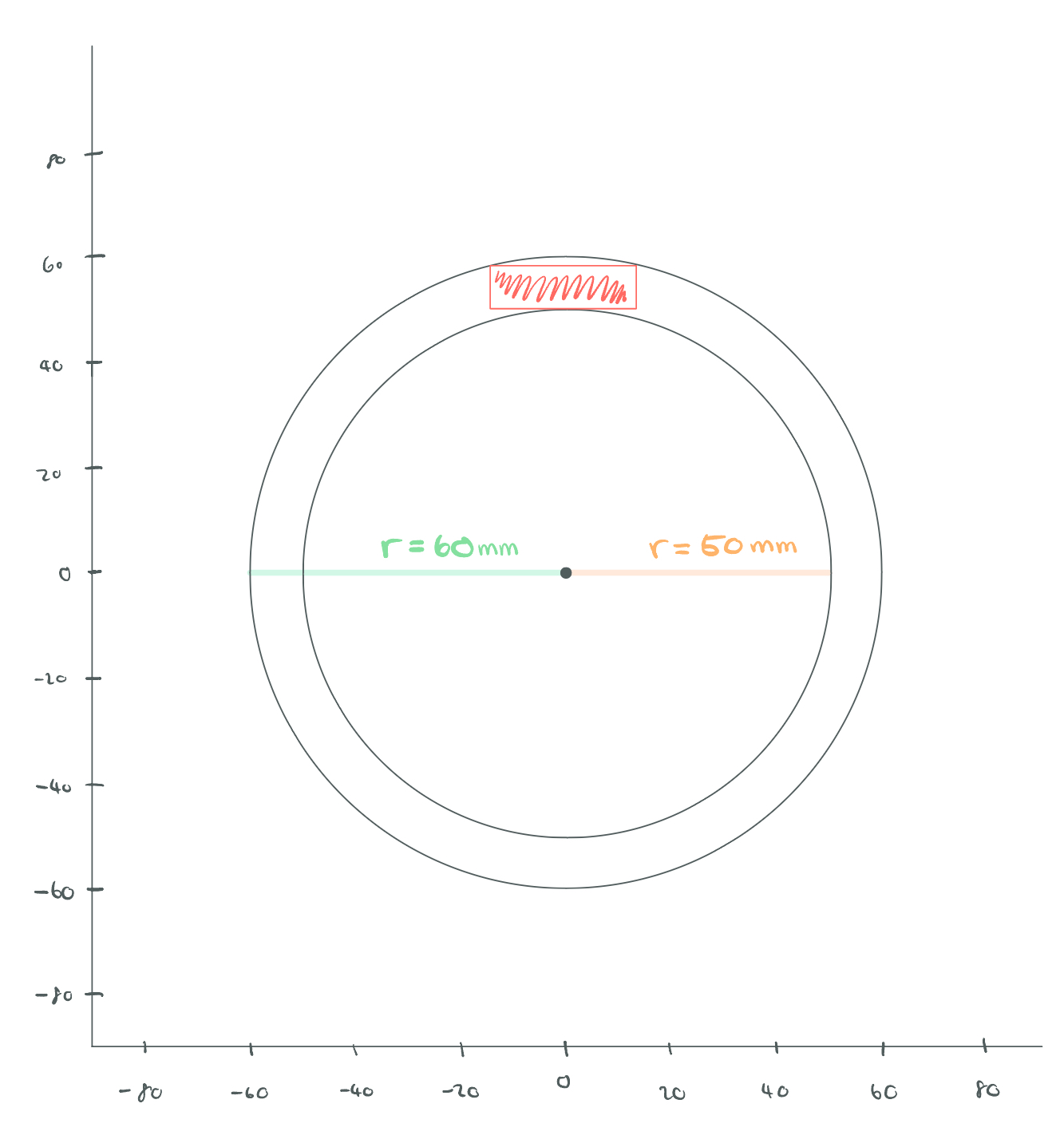
Example of rectangle inside two concentric circles.
If I have a rectangle ABCD such that A and B touch two points of the outer circle and CD's touches one point of the inner circle, how could the maximum dimensions of the rectangle be calculated?

Example of rectangle inside two concentric circles.
If $(x,y)$ is the coordinate of the upper right corner of the rectangle, with the origin at the center of the circles (inner radius $r_1$, outer radius $r_2$), then the area of the rectangle is $A=2x(y-r_1)$. Eliminate $y=\sqrt{r_2^2-x^2}$, differentiate $dA/dx$ and solve $dA/dx=0$ for $x$. For $r_1=50$, $r_2=60$ this gives the maximal area $$A_{\rm max}=\tfrac{1}{4} \sqrt{11900-500 \sqrt{313}} \left(\sqrt{500 \sqrt{313}+16900}-100 \sqrt{2}\right)=262.972$$
More generally, $$A_{\rm max}=\frac{1}{4} \sqrt{-r_1^2+4 r_2^2-\sqrt{r_1^4+8 r_1^2 r_2^2}} \left(-2 \sqrt{2}\, r_1+\sqrt{r_1^2+4 r_2^2+\sqrt{r_1^4+8 r_1^2 r_2^2}}\right).$$