Quoting myself from an earlier post:
Pólya's orchard problem asks for which radius $r$ of trees at each lattice point within a distance $R$ of the origin block all lines of sight to the exterior of the orchard. It has been established that rays to infinity are completely blocked iff $r \ge 1/\sqrt{R^2 + 1}$, when $R$ is an integer.
Q. Now I pose a variation of the same question, but for trees at each Gaussian prime.
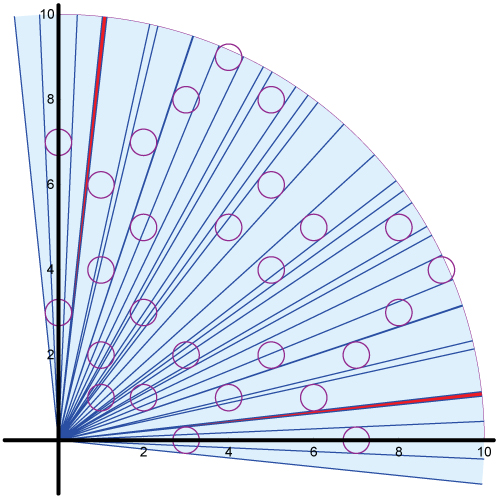
Here's an example for $R=10$. I set the tree radius to $0.31$, but the two thin red cones are not blocked: rays can reach beyond $R$. Increasing $r$ to $0.332$ closes up the sliver between primes $(3,0)$ and $(6,1)$, blocking all rays out to $R$.
I've made similar computations by overlaying on a red background the blue blocking cones for each prime, and gradually increasing $r$ until no underlying red cone remains.
This is a very crude calculation method, but it suggests that the $r \approx 1/R$ behavior for lattice point trees does not hold for Gaussian primes. In fact, $r$ seems to be constant (about $0.318$) for $20 \le R \le 100$, the limit of my calculations.