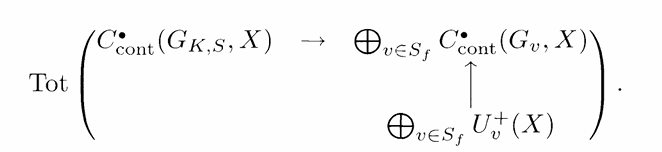3
$\begingroup$

Thanks for your reading. I'm studying Selmer complex book by Jan Nekovar. For the definition of Selmer complex I meet a problem.

In the introduction(page 9, 0.8.0) the author gives us a definition of Selmer complex. Let $X$ be a bounded complexes of admissible $R[G_{K,S}]$, where $R$ is a complete local Noetherian ring with finite residue field of $char$ $p$, K global field with $char(K) \neq p$, S the classical finite set of primes of K, $G_v$ the Galois group for the local field over prime $v \in S_f$. The local conditions we use here is a collection $\Delta(X)=(\Delta_v(X))_{v \in S_f}$, Where $\Delta_v(X)$ is a morphism of complexes of $R$-modules,

$i_v^+(X):U_v^+(X) \rightarrow C^\bullet_{cont}(G_v,X). $

Then Selmer complex is given by

enter image description here

As we know total complex is defined for double complex. But here for the content inside bracket, why it is a double complex by the direction of two arrows? It seems the two arrows can not make it a double complex? Then On the page 135 he gives us second definition in 6.1.2 enter image description here why the two form are the same? Thanks for your help!

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

You should view the morphisms of complexes of the notation with Tot as complexes of complexes concentrated in only two degrees with differential given by the morphism, which is a particularly simple case of a double complex. Then you can make the total complex of this double complex. A slight complication comes from the fact that there are two morphisms of complexes to consider and you are right that the meaning of Tot is never spelled out. The logical choice (which works) is to view them as a unique morphism from the direct sum of the two complexes $C_{\operatorname{cont}}^{\bullet}(G_{K,S},X)$ and of the complex $\bigoplus_{v\in S}U_{v}^+(X)$ to the complex $\bigoplus_{v\in S}C_{\operatorname{cont}}^{\bullet}(G_{v},X)$. Notice that the visual disposition of the complexes correctly implies that $\bigoplus_{v\in S}U_{v}^+(X)$ is shifted with respect to $C_{\operatorname{cont}}^{\bullet}(G_{K,S},X)$.

Now compare with the normalization of Cone which is spelled out in section (1.1.2), and you can check that both complexes are indeed the same (with the shift noted above explaining why $\operatorname{res}$ and $i^+_S(X)$ appear with opposite sign.

That being said, it is way more important you understand why Nekovář chose these definitions than to check formally that they are the same.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks for your explanation. So I should regard the two morphism in the first form as the morphism from the direct sum to the $\bigoplus_{v \in S} C^{\bullet}_{cont}(G_v,X)$? $\endgroup$
    – Rellw
    Commented Nov 28 at 20:50
  • $\begingroup$ i aslo have a quesiton that what's your meaning of "$\bigoplus_{v \in S} U^+_v(X)$ is shifted with respect to $C^\bullet_{cont}(G_{K,S},X)$"? I can understand that why finally our map should have two parts with opposite sign, because locally condition's aim is to restrict the image, making it within a set of $\bigoplus_{v \in S} C^{\bullet}_{cont}(G_v, X)$, which is given by $U_v^+(X)$. $\endgroup$
    – Rellw
    Commented Nov 28 at 21:03
  • $\begingroup$ Yes to the first question. By shift I mean that you should pay attention to the degrees of the complex, but since you have understood the restriction condition you are fine. Just read on (and read examples) and everything will make sense. $\endgroup$
    – Olivier
    Commented Nov 29 at 12:29

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .