I have the following infinite series $$S=\sum_{n=1}^\infty \frac{x^n}{n!}e^{\frac{c}{n}}, ~(x>0,c>0)$$ How could I perform this summation? Or is there any good analytical/closed-form approximation for this sum?
1 Answer
$\begingroup$
$\endgroup$
2
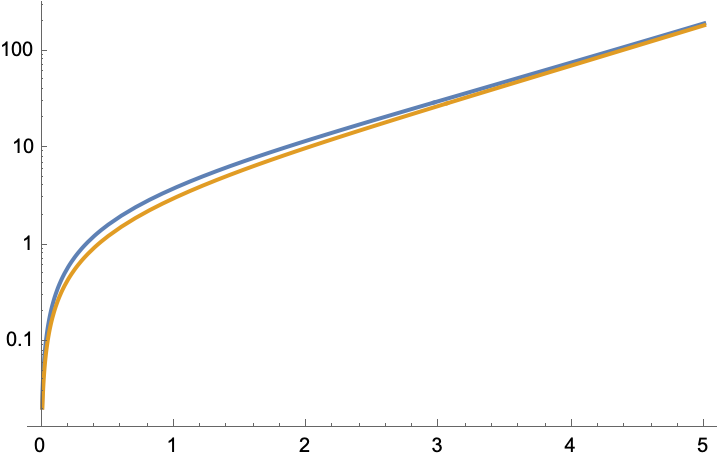
A closed-form answer is not forthcoming, but for $c\lesssim 1$ the small-$c$ approximation is quite accurate: $$S_0=\sum_{n=1}^\infty \frac{x^n}{n!}(1+c/n)=e^x-1+c \bigl[\text{Chi}(x)+\text{Shi}(x)-\ln x-\gamma_{\rm Euler} \bigr],$$ with Chi and Shi the hyperbolic cosine and sine integrals, respectively. The plot compares $S$ (blue) with $S_0$ (orange) as a function of $x$ for $c=1$.
answered Nov 20 at 12:08
-
$\begingroup$ The next ($k$th for $k \ge 2$) expansion coefficient in $c$ is easily written down with the help of generalized hypergeometric functions: $x \frac{c^{k}}{k!} \ _{k+1}F_{k+1}\left(1,..,1;2,..,2;x \right)$, with the $1$s and $2$s repeated $k+1$-times. $\endgroup$ Commented Nov 20 at 13:51
-
$\begingroup$ @Johannes Trost Sure, but then instead of the summation over n, we now need to do a summation over k. Btw, thanks. $\endgroup$– AD LeCommented Nov 20 at 14:54