$\newcommand{\Tr}{\mathrm{Tr}}$Lately I've been trying to gather more examples of centres and traces, hoping to write a comprehensive treatment on those on Clowder.
One of the examples I've been trying to understand better is the category of divisibility posets $\mathcal{D}$, which is defined as follows.
- For each $n\in\mathbb{N}_{\geq1}$, let $D_n$ be the poset consisting of the set $\{1,\ldots,n\}$ together with the preorder $\preceq$ given by declaring $k\preceq\ell$ if $k$ divides $\ell$.
- Then, let $\mathcal{D}$ be the full subcategory of the category $\mathsf{Pos}$ of posets and monotone maps spanned by the posets $D_n$ for all $n\in\mathbb{N}_{\geq1}$.
Recalling the definitions, the center of $\mathcal{D}$ is defined as \begin{align*} \mathrm{Z}(\mathcal{D})&\overset{\scriptstyle\mathrm{def}}{=}\int_{D_n\in\mathcal{D}}\mathrm{Hom}_{\mathcal{D}}(D_n,D_n)\\ &\cong\mathrm{Nat}(\mathrm{id}_{\mathcal{D}},\mathrm{id}_{\mathcal{D}}), \end{align*} while the trace of $\mathcal{D}$ is defined as \begin{align*} \mathrm{Tr}(\mathcal{D})&\overset{\scriptstyle\mathrm{def}}{=}\int^{D_n\in\mathcal{D}}\mathrm{Hom}_{\mathcal{D}}(D_n,D_n)\\ &\cong\left.\left(\coprod_{D_n\in\mathrm{Obj}(\mathcal{D})}\mathrm{Hom}_{\mathcal{D}}(D_n,D_n)\right)\middle/\mathord{\sim}\right., \end{align*} where $\sim$ is the equivalence relation generated by $f\circ g\sim g\circ f$. Finally, the quotient map $\mathrm{tr}$ from $\coprod_{D_n\in\mathrm{Obj}(\mathcal{D})}\mathrm{Hom}_{\mathcal{D}}(D_n,D_n)$ to $\mathrm{Tr}(\mathcal{D})$ is called the trace map of $\mathcal{D}$.
Looking at naturality with respect to the maps $[k]\colon D_1\to D_n$ given by $1\mapsto k$ shows the centre of $\mathcal{D}$ to be trivial. However, as is usual with centres and traces, computing the trace seems to be much, much harder.
Question. Is there a neat description of the trace of $\mathcal{D}$ and the trace map?
(By "neat" here I'm thinking of something like how conjugacy classes of symmetric groups may be described as Young diagrams.)
Here are some miscellaneous notes:
Numerical Experiments. The trace of the category $\mathcal{D}_m$ spanned by the divisibility posets $D_1,\ldots,D_m$ is described for $1\leq m\leq8$ here.
In particular, the cardinality of $\mathrm{Tr}(\mathcal{D}_m)$ for $1\leq m\leq8$ is $(1,2,4,6,11,21,39,59)$, for which OEIS gives nothing.
For example, the equivalence classes in $\mathrm{Tr}(\mathcal{D}_3)$ are the following, where e.g. $(1,3,2)$ denotes the map $D_3\to D_3$ sending $(1,2,3)$ to $(1,3,2)$:
Class 1:
D_1 -> D_1: (1,)
D_2 -> D_2: (1, 1)
D_2 -> D_2: (2, 2)
D_3 -> D_3: (1, 1, 1)
D_3 -> D_3: (1, 1, 2)
D_3 -> D_3: (1, 3, 1)
D_3 -> D_3: (2, 2, 2)
D_3 -> D_3: (3, 3, 3)
Class 2:
D_2 -> D_2: (1, 2)
D_3 -> D_3: (1, 1, 3)
D_3 -> D_3: (1, 2, 1)
D_3 -> D_3: (1, 2, 2)
D_3 -> D_3: (1, 3, 3)
Class 3:
D_3 -> D_3: (1, 2, 3)
Class 4:
D_3 -> D_3: (1, 3, 2)
Numerical evaluation suggests that increasing $m$ does not add new identifications between endomorphisms of $D_k$ and $D_\ell$ for $k,\ell\leq m$, i.e. if $f\colon D_k\to D_k$ and $g\colon D_\ell\to D_\ell$ are not equal in $\mathrm{Tr}(\mathcal{D}_n)$, then they remain not equal in $\mathrm{Tr}(\mathcal{D}_{n+1})$. In other words, the natural map $$\mathrm{Tr}(\mathcal{D}_n)\to\mathrm{Tr}(\mathcal{D}_{n+1})$$ seems to be injective. (Take this with a grain of salt however; I was only able to run these experiments for very small $n$.)
Fixed Points and Invariants. One strategy for computing traces is to find invariants under the relation $f\circ g\sim g\circ f$.
In our case, the number of fixed points of an endomorphism of $\mathcal{D}$ is one such invariant (by the same argument given in MO 469617).
However, this does not give a complete characterization of the trace map, since e.g. the maps $(1,1,1)$ and $(1,3,2)$ in $\mathcal{D}_3$ have the same number of fixed points but belong to different elements of $\mathrm{Tr}(\mathcal{D}_3)$.
Relation to Conjugacy Classes. I believe there's an isomorphism $$\mathrm{Tr}(\mathcal{D}_n)\cong\mathrm{Cl}(\mathrm{End}(D_n)),$$ where $\mathrm{Cl}(\mathrm{End}(D_n))$ denotes the set of ($\sim^*_p$) conjugacy classes of $\mathrm{End}(D_n)$.
Proof. Let $n,k\in\mathbb{N}$ with $k<n$. We have a commutative diagram in $\mathcal{D}_{n}$ of the form
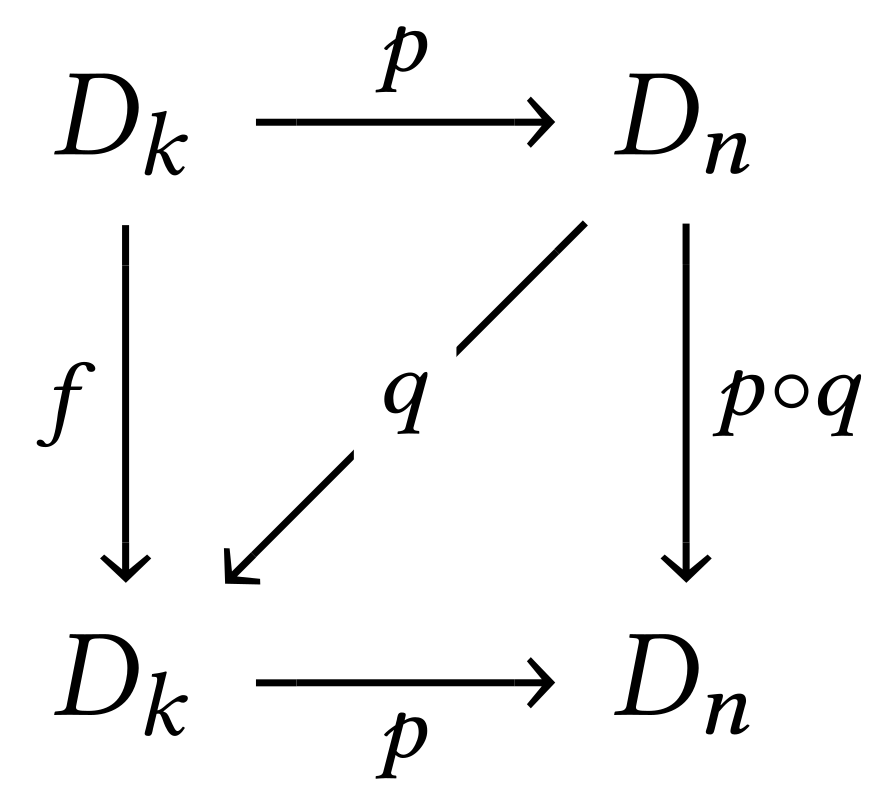
where $p$ is given by $f$ composed with the inclusion $D_k\to D_n$ and $q$ is defined as follows:
- If $i\leq k$, then $q(i)=i$.
- If $i\geq k$, then we have $i=ab$ for some $a,b\in\mathbb{N}$ with $a\in D_k$ and $b$ as small as possible. We then define $q(i)=a$.
With this definition, if $i\mid j$, then $q(i)\mid q(j)$, so $q$ is indeed a morphism of posets. The existence of this commutative diagram gives $[f]=[p\circ q]$ in $\mathrm{Tr}(\mathcal{D}_n)$, so the map $\mathrm{Tr}(\mathcal{D}_n)\to\mathrm{Cl}(D_n)$ sending $f$ to $p\circ q$ is well defined. Injectivity and surjectivity of this map then follow from the fact that the relation $\sim$ on $\mathcal{D}_n$ restricts to the ($\sim^*_p$) conjugacy relation in $\mathrm{End}(D_n)$.
