4
$\begingroup$

I am writing a thesis on algebraic logic, I wonder if there is any recent research on an idea mentioned in Yuri Manin's book on algebraic geometry and in another Russian textbook on differential geometry (in which they briefly mentioned this idea).

Basically the idea is to associate Boolean logic with Boolean rings (which appears in many places). But further research seems needed to extend this to first-order logics.

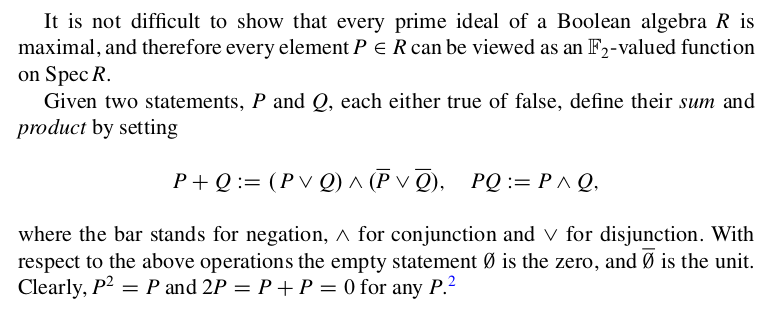
This is from Yuri Manin's 2018 book, Introduction to the Theory of Schemes:

enter image description here enter image description here

but I cannot find where he developed the idea further.

The following is from another Russian text, Basic Ideas and Concepts of Differential Geometry, by D.V. Alekseevskij, A.M. Vinogradov, V.V. Lychagin.

The Russian title is: Itogi nauki i tekhniki, Sovremennye problemy matematiki, FundamentaFnye napravleniya, Vol. 28, Geometriya 1, Publisher VINITI, Moscow 1988.

From Chapter 1:

enter image description here enter image description here enter image description here enter image description here

I am not aware of any more research further along this direction. How can I do a more thorough search of the literature? Or if anyone can point to some relevant research would be greatly appreciated.

I am already aware of the recent literature in (abstract) algebraic logic and categorical logic, but not this particular idea which is inspired from algebraic geometry.

Improve this question
$\endgroup$
4
  • 2
    $\begingroup$ Maybe Spencer Breiner's thesis, Scheme representation for first-order logic, could be relevant. $\endgroup$
    – Emily
    Commented Oct 23 at 11:19
  • 2
    $\begingroup$ The spectrum of a Boolean ring is usually called (by people other than algebraic geometers) the Stone space of the ring. This representation of arbitrary Boolean rings as rings of sets was proved by M. Stone in the 1930's. Googling "Stone space" should produce plenty of references about this (and generalizations). $\endgroup$
    – Andreas Blass
    Commented Oct 23 at 14:33
  • 3
    $\begingroup$ The old way of algebra-ising first-order logic is with cylindric algebras, but probably people prefer to think about Lawvere hyperdoctrines these days. $\endgroup$
    – Zhen Lin
    Commented Oct 23 at 14:50
  • 1
    $\begingroup$ @Emily 100%. Thanks, it looks like I missed a lot of important development... but the thesis provides other references. $\endgroup$
    – YKY
    Commented Oct 23 at 17:50

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .