21
$\begingroup$

Draw $n$ random chords in a circle, where each chord connects two independent uniformly random points on the circle.

As $n\to\infty$, which kind of polygon (triangle, quadrilateral, pentagon, etc.) occupies the most total area?

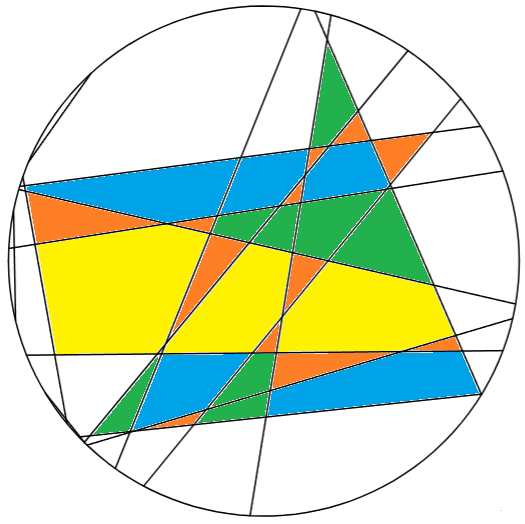
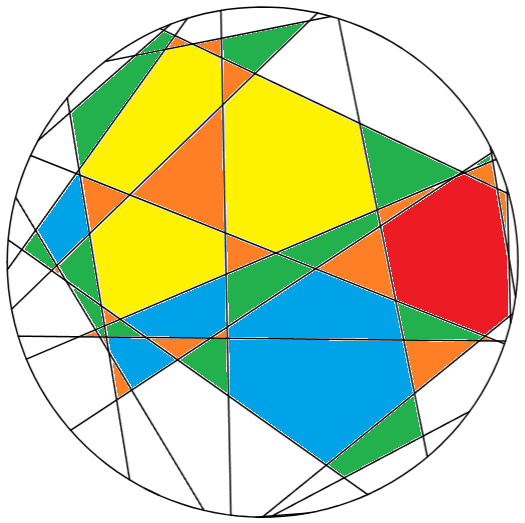
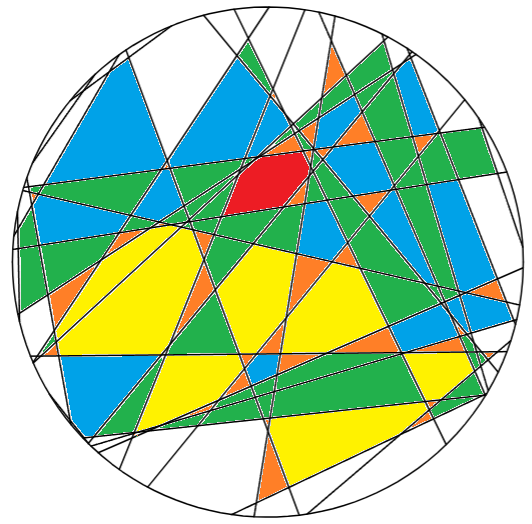
In the following examples, the polygons are color-coded.

$n=15$:

enter image description here

$n=20$:

enter image description here

$n=30$:

enter image description here

Triangles seem to be the most numerous, but smallest (in terms of average size). As the number of sides increases, the polygons seem to get fewer, but bigger.

I guess that, as $n\to\infty$, one kind of polygon should emerge as the most dominant, in terms of total area.

I made a random chord generator. Unfortunately, it does not color-code the polygons. (For the examples shown above, I color-coded the polygons manually.)

Context: This is another question of mine about many random chords in a circle. Earlier questions are here and here.

Improve this question
$\endgroup$
4
  • 4
    $\begingroup$ Empirically it seems to be pentagons. Out of $1000$ trials of $5m$ chords for $m \in [1, 20]$ pentagons took the lead at $20$ chords with $416$ wins (vs $395$ for quadrilaterals), and by $100$ chords they were winning more than $80\%$ of trials, with quadrilaterals picking up the rest. $\endgroup$
    – Peter Taylor
    Commented Oct 25 at 12:09
  • 1
    $\begingroup$ Wouldn't the fraction of the area covered by $d$-gons converge as $n\to\infty$ to the side distribution $p_d$ of the Crofton cell of the Poisson line process? See the summary of Hilhorst & Calka in Section 1.2 of arXiv:0802.1869, which claims that $p_d$ has mean $\pi^2/2=4.93\ldots$ and peaks at pentagons $d=5$, citing Matheron. $\endgroup$
    – Timothy Budd
    Commented Oct 27 at 10:22
  • $\begingroup$ @TimothyBudd Is the side distribution of the Crofton cell the same as the side distribution of a typical cell? $\endgroup$
    – Dan
    Commented Oct 27 at 14:25
  • 1
    $\begingroup$ No, see the same paragraph of the paper Hilhorst & Calka. The Crofton cell is biased by the area, compared to the typical cell. $\endgroup$
    – Timothy Budd
    Commented Oct 28 at 7:52

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .