I found a very explicit counterexample by running some numbers in Rust:
- Take $\mathbb C := (0 \rightarrow 1)$ the walking arrow, so that $\mathbb C^{\textsf{op}} \times \mathbb C$ becomes a commutative square of arrows $A\,;B = C\,;D$ (we take $A,B$ to always be on the upper-half of the hexagon and $C,D$ to be on the bottom-half).
- Take $\mathbb D := \{\{0,1\}\}$ the subcategory of $\textsf{Set}$ consisting of just the set $\{0,1\}$.
Then consider the following situation, where $\textsf K_0 : \{0,1\} \rightarrow \{0,1\}$ indicates the constant map in $0$:
- Take $F = G : \mathbb C^{\textsf{op}} \times \mathbb C \rightarrow \mathbb D$ doing the only possible thing on objects and mapping $A,B,C,D \mapsto \textsf K_0$. Functoriality is satisfied since $\textsf{K}_0\,;\textsf{K}_0 = \textsf{K}_0\,;\textsf{K}_0$.
- Take $H : \mathbb C^{\textsf{op}} \times \mathbb C \rightarrow \mathbb D$ maps $A,B,C \mapsto \textsf K_0$ and $D \mapsto\textsf{id}$. Functoriality is satisfied since $\textsf{K}_0\,;\textsf{K}_0 = \textsf{K}_0\,;\textsf{id}$.
Consider the following hexagons, where $\alpha_{i\in\{0,1\}} = \textsf{swap}$ and $\beta_{i\in\{0,1\}} := \textsf{id}$:
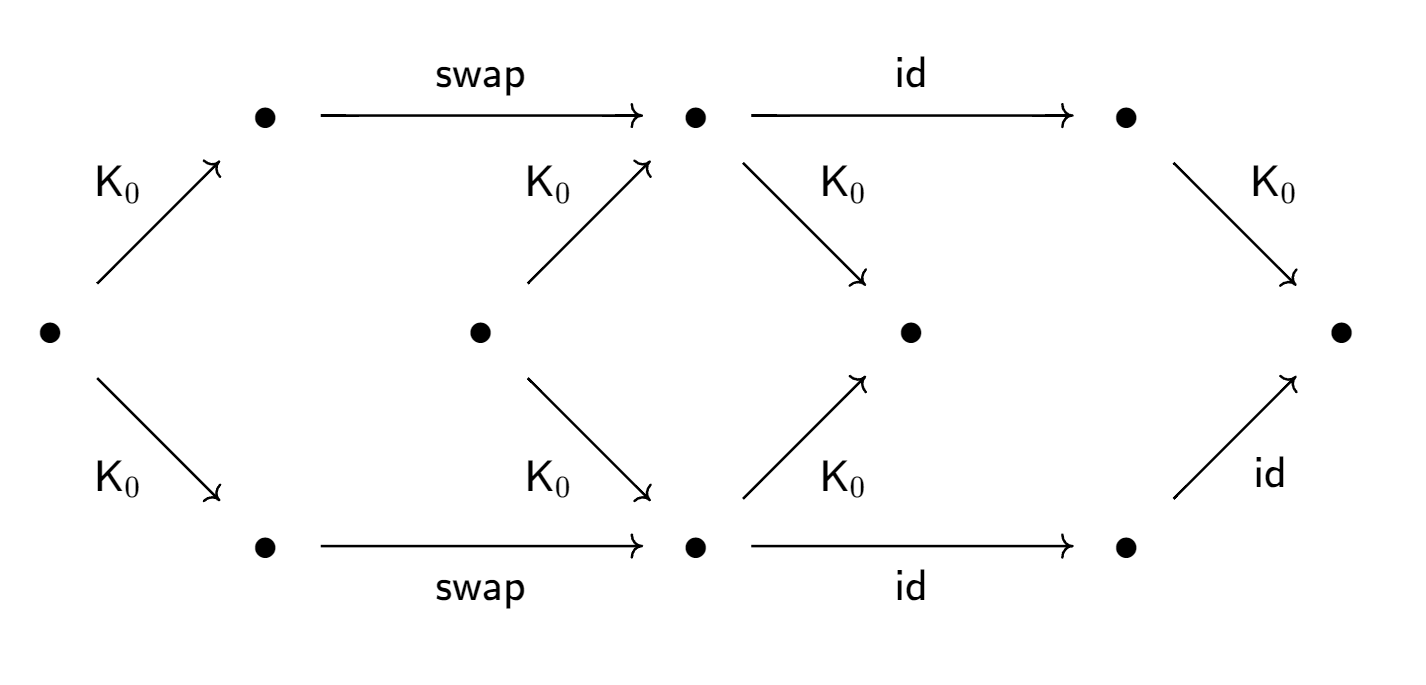
Clearly the hexagons commute since the $\textsf K_0$s collapse everything together; but now the top side of the outer hexagon is $\textsf K_0$, and the bottom side is $\textsf K_1$.
Here is the Rust code I used to generate this counterexample!
Inspired by @varkor's comment, here is an even simpler example where the codomain is the 2-element monoid $\mathbb D := \{\textsf{id}, \color{red}{a}\}$ such that $\color{red}{a} \,; \color{red}{a} = \color{red}{a}$.
Consider the following situation, where black morphisms indicate the identity $\textsf{id}$ and red morphisms indicate the element/morphism $\color{red}{a}$:
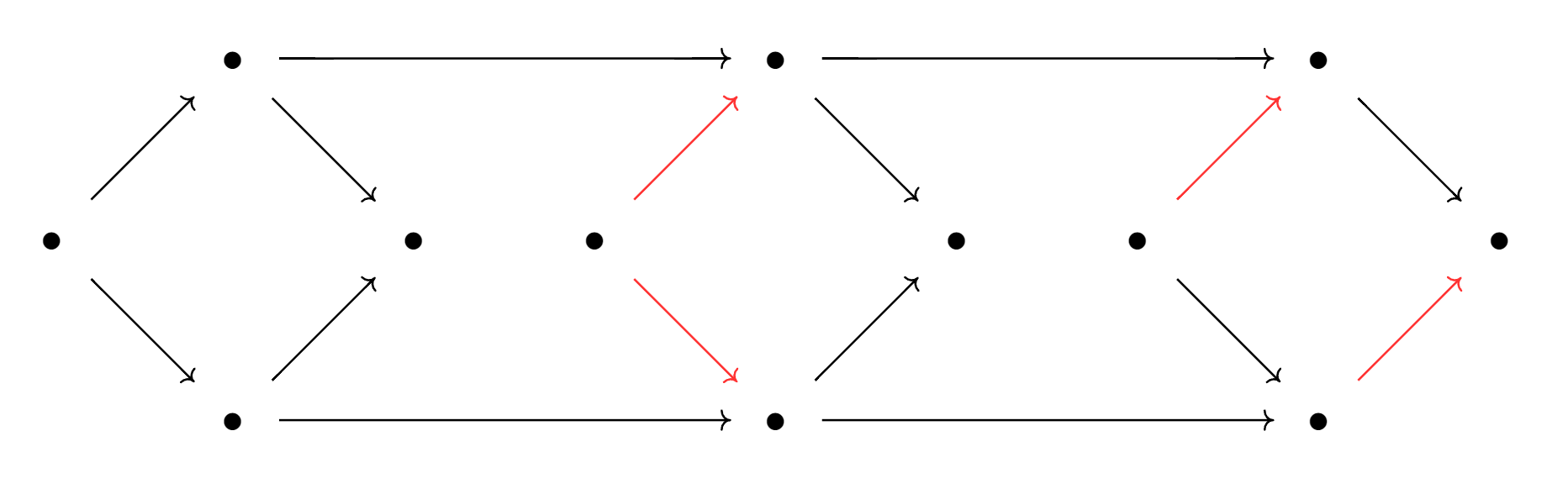
Functoriality holds since each square is either trivial or has at least one red morphism on both the top and bottom sides.
The hexagon on the left commutes since it only has identities, and the hexagon on the right commutes since each side has at least one red morphism.
However, the outer hexagon does not commute since the top side is the identity $\textsf{id}$ but the bottom side is $\color{red}{a}$.
(More formally, the functors are defined as follows,
- $F : \mathbb{C}^{\textsf{op}} \times \mathbb{C} \rightarrow \mathbb{D}$ sends $A,B,C,D\mapsto \textsf{id}$,
- $G : \mathbb{C}^{\textsf{op}} \times \mathbb{C} \rightarrow \mathbb{D}$ sends $A,C\mapsto \color{red}{a}$ and $B,D\mapsto \textsf{id}$,
- $H : \mathbb{C}^{\textsf{op}} \times \mathbb{C} \rightarrow \mathbb{D}$ sends
$A,D\mapsto \color{red}{a}$ and $B,C\mapsto \textsf{id}$,
and the dinatural families $\alpha,\beta$ are given by $\alpha_{i} = \beta_{i} = \textsf{id}$.)



