51
$\begingroup$

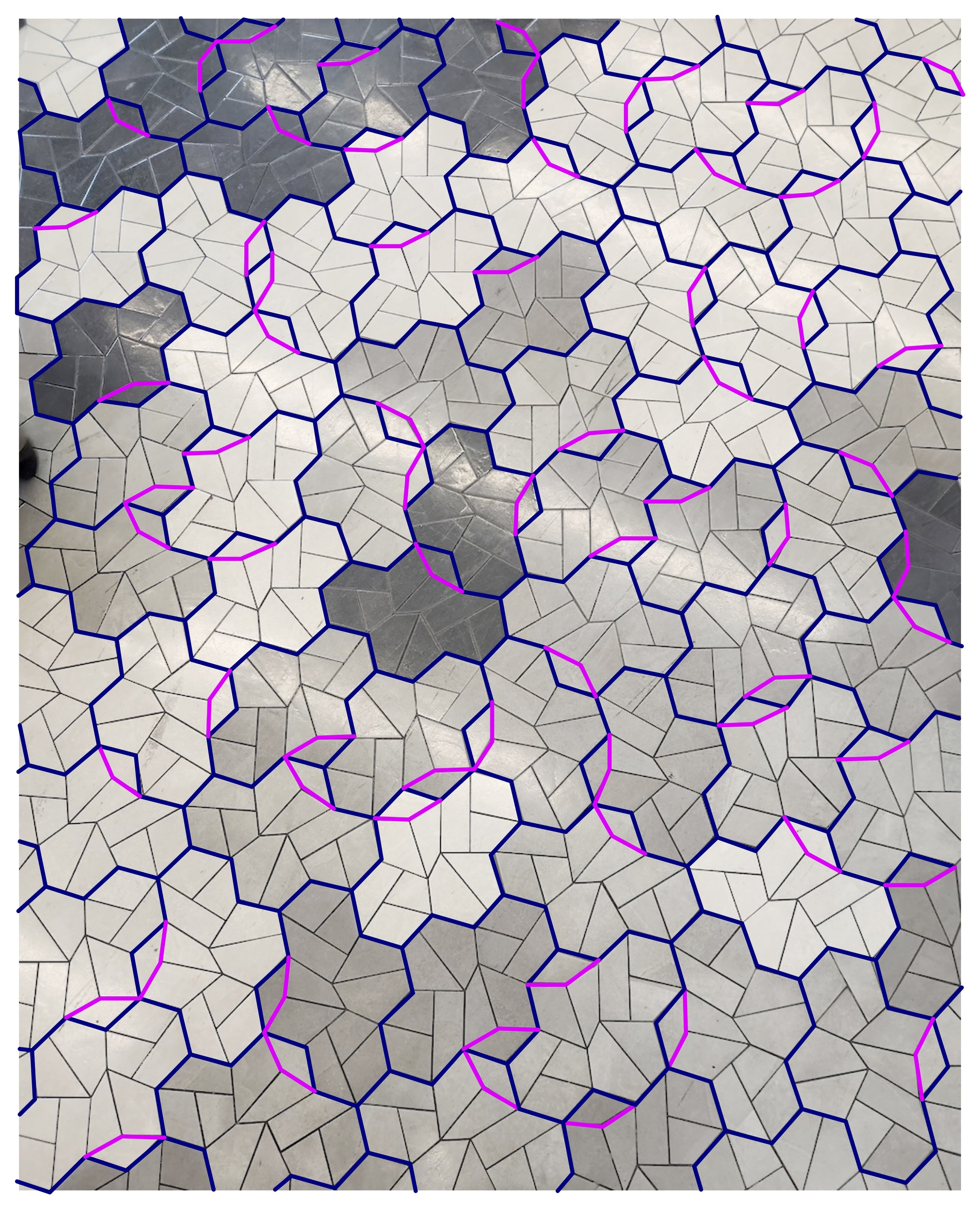
I noticed that the new terminal at LaGuardia Airport (in New York) has an intriguing design for the tiles on at least one of their floor areas. It bears a superficial resemblance to aperiodic tilings such as the Penrose tiling, with its occasional "arc-like" patterns. Then again, it seems to be merely based on a deformed hexagonal grid. But then again, the aperiodic hat tiling itself is based on a grid, so a grid pattern alone doesn't rule out the idea that there might be some mathematical significance here! Is it aperiodic? Does it have any mathematical interest? (Perhaps learning who designed/selected the pattern would help; I also don't know anything about that.) FWIW, the Laguardia B FAQ doesn't mention anything about the floors.

Here's a representative sample:

Improve this question
$\endgroup$
7
  • 3
    $\begingroup$ @GerryMyerson -- that quote refers to wall tiles in terminal C, the floor tiles are in terminal B $\endgroup$
    – Carlo Beenakker
    Commented Sep 10 at 6:41
  • 4
    $\begingroup$ @Carlo, you may be right, but the quote I gave begins, "Our handmade mosaic tiles cover nearly 25,000 square feet of LaGuardia Airport's newly renovated Terminal B. Designed by critically acclaimed painter Laura Owens, this monumental ceramic tile mosaic," $\endgroup$
    – Gerry Myerson
    Commented Sep 10 at 7:15
  • 4
    $\begingroup$ you are right, the wall tiles are also in terminal B, not C, my mistake $\endgroup$
    – Carlo Beenakker
    Commented Sep 10 at 7:24
  • 5
    $\begingroup$ It looks to me like, topologically, this is the standard hexagonal tiling, but the hexagons are irregular and each is subdivided into two or three pieces. $\endgroup$
    – David E Speyer
    Commented Sep 10 at 15:55
  • 5
    $\begingroup$ It seems to be made of groups of four hexagons. $\endgroup$
    – Ben Burns
    Commented Sep 10 at 17:08

3 Answers 3

Reset to default
47
$\begingroup$

You can view this pattern as consisting of major and minor tiles. The major tiles are the union of four hexagons. These tiles are all identically subdivided into eleven minor tiles.

Four-hexagon tile

In the picture below the edges of the major tiles are highlighted in blue. The pink lines are one type of edge of the minor tiles. When they line up in a certain way, these give rise to the arcs observed in the question.

Tiling with highlighted edges

Ignoring the minor tiles, the tiling is periodic. The fundamental domain consists of seven major tiles:

Fundamental domain

The distribution of the pink edges in the previous picture suggests that the orientations of the major tiles (there are two possibilities for each one) are random. And finally, here is the original picture with the same coloring overlaid:

Colored tile overlay

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Excellent image-editing! Thank you! The next question is: are the four-hex units put together in any particular way — periodically, randomly, in some interesting way? Of course the answer might be "we can't tell from this small sample." $\endgroup$
    – Quuxplusone
    Commented Sep 11 at 12:49
  • 2
    $\begingroup$ The layout of the major tiles appears to be periodic, but their orientations seem to be random. I'll add another pictures with more colors to illustrate this in a little while. $\endgroup$
    – N M
    Commented Sep 11 at 18:06
27
$\begingroup$

Concerning the secondary question who designed the pattern:

The tiled floor in LaGuardia terminal B was designed by HOK and installed by Consolidated Flooring. They received an award for this. The description does not mention any math connection:

The design draws on the architecture, history, and geography of New York city. The flooring was used masterfully to accentuate the design elements, encourage traffic flow, and hide heavy traffic wear throughout the grand space.

Improve this answer
$\endgroup$
13
$\begingroup$

For those who can't quite see the underlying hexagonal tiling mentioned in David Speyer's comment, I have highlighted part of it below. Please excuse my crude digital editing skills.

enter image description here.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .