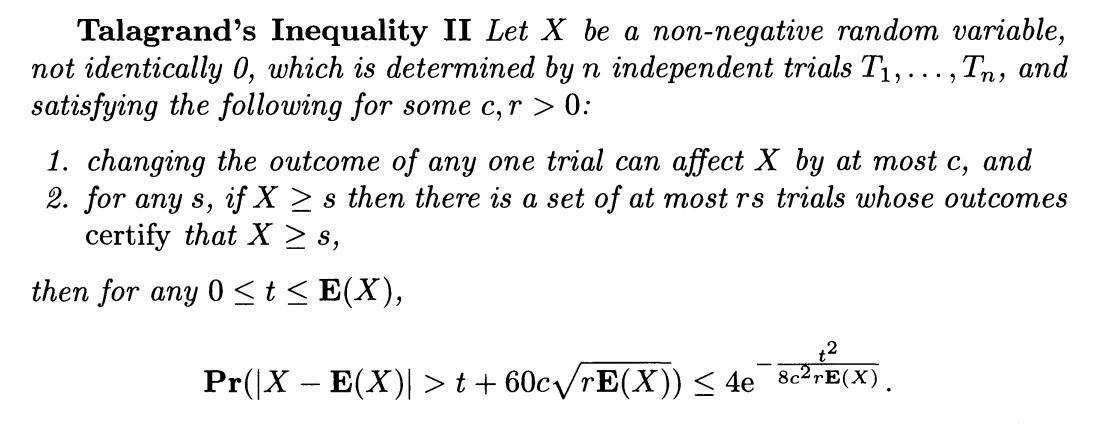3
$\begingroup$

Recently I am trying to use Talagrand concentration inequality to do something on graphs. I find a version from the book of Molloy and Reed ''Graph Colouring and Probabilistics Method''. I attached a picture from that book below. I am very confused with that whether the bound $E[X]$ for $t$ is necessary. Can we ignore it when apply Talagrand inequality? If we can do so, is there any publication talking about this?

enter image description here

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ In Concentration Inequalities: A Nonasymptotic Theory of Independence academic.oup.com/book/26549, they go over many of variations of concentration inequalities that work for arbitrarily large t. $\endgroup$
    – Thomas Kojar
    Commented Sep 7 at 3:50

0

Reset to default

You must log in to answer this question.