5
$\begingroup$
  1. Let a hexagon $AB'CA'BC'$ let $AB' \cap A'B=C''$, $BC' \cap B'C = A''$, $CA' \cap C'A = B''$ then three conditions as follows equivalent:
  • Three lines $AA', BB', CC'$ are concurrent (let the point of concurrence is $M$);
  • Three lines $AA'', BB'', CC''$ are concurrent (let the point of concurrence is $N$);
  • Three lines $A'A'', B'B'', C'C''$ are concurrent (let the point of concurrence is $P$);
  1. If $AA', BB', CC'$ are concurrent then three points $M, N, P$ are collinear.

Have you ever seen this result before? I am looking for a proof of this result.

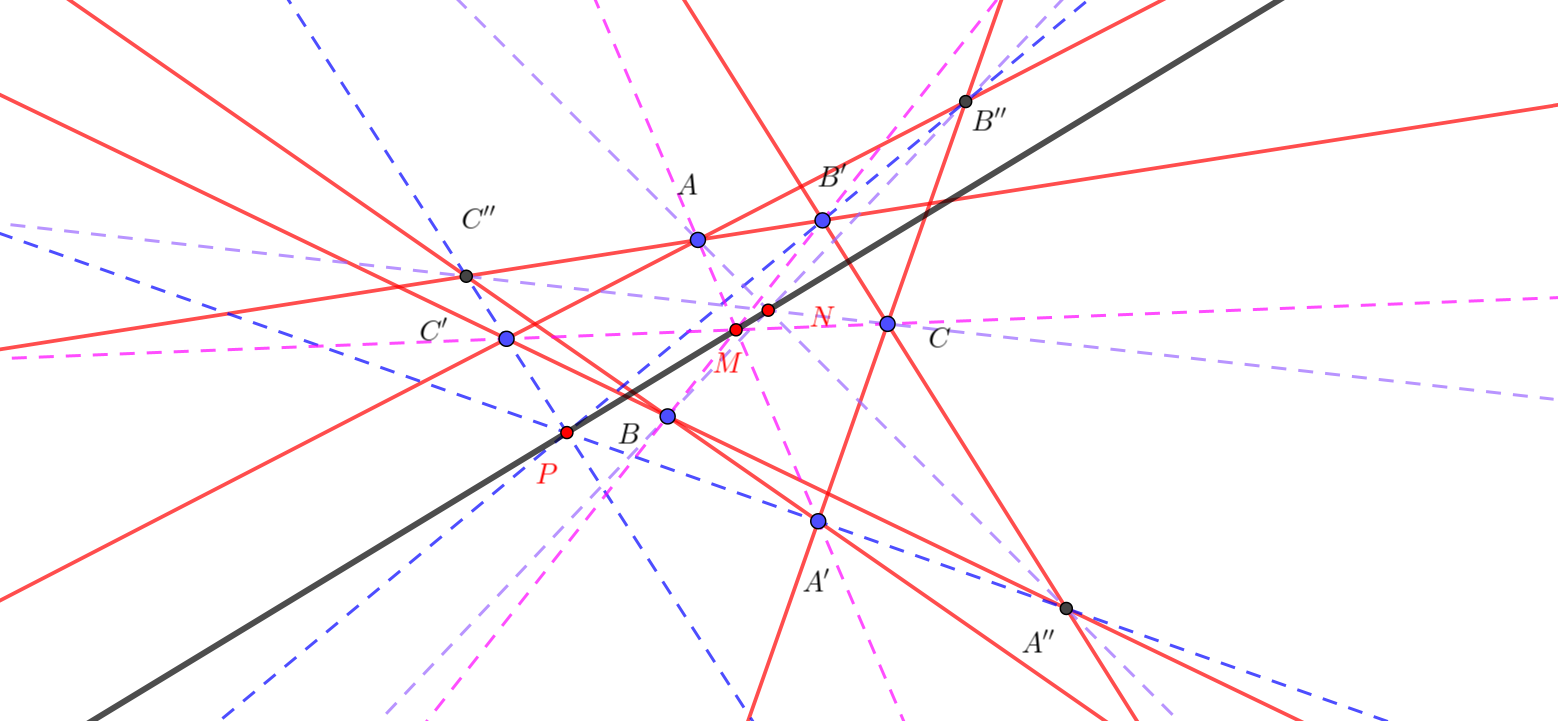
enter image description here

Improve this question
$\endgroup$
7
  • 2
    $\begingroup$ I don't think it's entirely fair to call this a "theorem" if you don't have a proof of the statement. $\endgroup$
    – Wojowu
    Commented Aug 29 at 9:46
  • $\begingroup$ Thank You I will correct title $\endgroup$
    – Đào Thanh Oai
    Commented Aug 29 at 10:55
  • 1
    $\begingroup$ I have worked out a (computational) proof (needs to be double checked to earn the name of a theorem) which also gives a quantitative version for the cases where you don't have concurrency (i.e. computes the relationships between the triangles bounded by the three lines in each case). $\endgroup$
    – terceira
    Commented Aug 29 at 11:49
  • 2
    $\begingroup$ Recent work by Fomin an Pylyavskyy, Incidences and tilings, provides a new perspective on this type of incidence theorem, and in particular suggests that there ought to be a proof of your result that consists of finding an appropriate tiling of a compact surface, and then applying their master theorem. That doesn't directly answer your question about whether the result is new, but their paper has lots of information about existing incidence theorems. $\endgroup$
    – Timothy Chow
    Commented Aug 29 at 14:59
  • 1
    $\begingroup$ This is a picture of a cube in 3D :) $\endgroup$
    – Piotr Achinger
    Commented Aug 29 at 17:34

1 Answer 1

Reset to default
14
$\begingroup$

All these conditions are equivalent to six lines $AB'C''=a$, $CB'A''=b$, $CA'B''=c$, $BA'C''=d$, $BC'A''=e$, $AC'B''=f$ being tangent to the same conic (by Brianchon theorem and its inverse). For these six lines, there are 60 Brianchon points which can be partitioned onto 20 collinear triples. This was proved by Steiner in 1828, see, for example,

[1] J. Conway and A. Ryba, The Pascal Mysticum demystified, Math. Intelligencer 34 (2012), 4–8

(this paper is on the dual language: 60 Pascal lines instead of 60 Brianchon points, etc).

Your triple is a Steiner one. To see this, we use the notation from [1]: a Brianchon point is defined by the cyclic permutations of the six lines, which is considered as the element of the symmetric group $S_6$. There are two 6-cycles which give the same Brianchon point (they are mutually inverse). Steiner's claim is that if three cycles $\pi_1$, $\pi_2$, $\pi_3$ have the same square $\pi_1^2=\pi_2^2=\pi_3^2$, then three corresponding Brianchon points are collinear. Your points $M,N,P$ are Brianchon points for the 6-cycles $(abcdef)$, $(afcbed)$, $(badcfe)$ respectively, these cycles have the same square $(ace)(bdf)$.

Improve this answer
$\endgroup$
6
  • $\begingroup$ How can proof these points are collinear? $\endgroup$
    – Đào Thanh Oai
    Commented Aug 29 at 13:14
  • $\begingroup$ About Pascal line: The 60 Pascal lines intersect three at a time through 20 Steiner points (some of which are shown as the filled circles in the above figures). In the symmetrical case of the regular hexagon inscribed in a circle, the 20 Steiner points degenerate into seven distinct points arranged at the vertices and center of a regular hexagon centered at the origin of the circle. The 60 Pascal line also intersect three at a time in 60 Kirkman points. Each Steiner point lines together….. See in here $\endgroup$
    – Đào Thanh Oai
    Commented Aug 29 at 15:40
  • $\begingroup$ Your reference here but I don’t think it is the answer for second question. $\endgroup$
    – Đào Thanh Oai
    Commented Aug 29 at 23:29
  • $\begingroup$ I think it does answer, I added the detailed explanation $\endgroup$
    – Fedor Petrov
    Commented Aug 30 at 4:52
  • $\begingroup$ Please help me check again. Because, I think with six lines $a, b, c, d, f$ above, there are only (there are maximum) $3$ Brianchon points $M, N, P$. $\endgroup$
    – Đào Thanh Oai
    Commented Aug 30 at 6:31

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .